Condorcet paradox

The Condorcet paradox (also known as voting paradox or the paradox of voting) is a situation noted by the Marquis de Condorcet in the late 18th century,[1][2][3] in which collective preferences can be cyclic, even if the preferences of individual voters are not cyclic. This is paradoxical, because it means that majority wishes can be in conflict with each other. When this occurs, it is because the conflicting majorities are each made up of different groups of individuals.

Thus an expectation that transitivity on the part of all individuals' preferences should result in transitivity of societal preferences is an example of a fallacy of composition.

Example

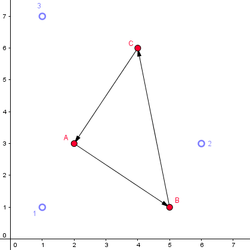
3 blue dots in a triangle. 3 red dots in a triangle, connected by arrows that point counterclockwise.
Voters (blue) and candidates (red) plotted in a 2-dimensional preference space. Arrows show the order in which voters prefer the candidates.

Suppose we have three candidates, A, B, and C, and that there are three voters with preferences as follows (candidates being listed left-to-right for each voter in decreasing order of preference):

Voter First preference Second preference Third preference
Voter 1 A B C
Voter 2 B C A
Voter 3 C A B

If C is chosen as the winner, it can be argued that B should win instead, since two voters (1 and 2) prefer B to C and only one voter (3) prefers C to B. However, by the same argument A is preferred to B, and C is preferred to A, by a margin of two to one on each occasion. Thus the society's preferences show cycling: A is preferred over B which is preferred over C which is preferred over A. A paradoxical feature of relations between the voters' preferences described above is that although the majority of voters agree that A is preferable to B, B to C, and C to A, all three coefficients of rank correlations between the voters' preferences are negative (namely, -.5), as calculated with Spearman's rank correlation coefficient formula designed by Charles Spearman much later.[4]

Cardinal ratings

Note that in the graphical example, the voters and candidates are not symmetrical, but the ranked voting system "flattens" their preferences into a symmetrical cycle.[5] Cardinal voting systems provide more information than rankings, allowing a winner to be found.[6][7] For instance, under Score voting, the ballots might be:[8]

A B C
1 6 3 0
2 0 6 1
3 5 0 6
Total: 11 9 7

Candidate A gets the largest score, and is the winner, as A is the nearest to all voters.

Necessary condition for the paradox

Suppose that x is the fraction of voters who prefer A over B and that y is the fraction of voters who prefer B over C. It has been shown[9] that the fraction z of voters who prefer A over C is always at least (x + y – 1). Since the paradox (a majority preferring C over A) requires z < 1/2, a necessary condition for the paradox is that

Implications

When a Condorcet method is used to determine an election, the voting paradox of cyclical societal preferences implies that the election has no Condorcet winner: no candidate who can win a one-on-one election against each other candidate. The several variants of the Condorcet method differ on how they resolve such ambiguities when they arise to determine a winner.[10] Note that using only rankings, there is no fair and deterministic resolution to the trivial example given earlier because each candidate is in an exactly symmetrical situation.

Situations having the voting paradox can cause voting mechanisms to violate the axiom of independence of irrelevant alternatives—the choice of winner by a voting mechanism could be influenced by whether or not a losing candidate is available to be voted for.

One important implication of the possible existence of the voting paradox in a practical situation is that in a two-stage voting process, the eventual winner may depend on the way the two stages are structured. For example, suppose the winner of A versus B in the open primary contest for one party's leadership will then face the second party's leader, C, in the general election. In the earlier example, A would defeat B for the first party's nomination, and then would lose to C in the general election. But if B were in the second party instead of the first, B would defeat C for that party's nomination, and then would lose to A in the general election. Thus the structure of the two stages makes a difference for whether A or C is the ultimate winner.

Likewise, the structure of a sequence of votes in a legislature can be manipulated by the person arranging the votes, to ensure a preferred outcome.

See also

References

  1. ↑ Marquis de Condorcet. "Essai sur l'application de l'analyse à la probabilité des décisions rendues à la pluralité des voix" (PNG) (in French). Retrieved 2008-03-10.
  2. ↑ Condorcet, Jean-Antoine-Nicolas de Caritat; Sommerlad, Fiona; McLean, Iain (1989-01-01). The political theory of Condorcet. Oxford: University of Oxford, Faculty of Social Studies. pp. 69–80, 152–166. Clearly, if anyone’s vote was self-contradictory (having cyclic preferences), it would have to be discounted, and we should therefore establish a form of voting which makes such absurdities impossible
  3. ↑ Gehrlein, William V. "Condorcet's paradox and the likelihood of its occurrence: different perspectives on balanced preferences*". Theory and Decision. 52 (2): 171–199. ISSN 0040-5833. doi:10.1023/A:1015551010381. Here, Condorcet notes that we have a ‘contradictory system’ that represents what has come to be known as Condorcet’s Paradox.
  4. ↑ Poddiakov, A., & Valsiner, J. (2013). Intransitivity cycles and their transformations: How dynamically adapting systems function. In L. Rudolph (Ed.), Qualitative Mathematics for the Social Sciences: Mathematical Models for Research on Cultural Dynamics (pp. 343–391). Abingdon, NY: Routledge.
  5. ↑ Procaccia, Ariel D.; Rosenschein, Jeffrey S. (2006-09-11). Klusch, Matthias; Rovatsos, Michael; Payne, Terry R., eds. The Distortion of Cardinal Preferences in Voting (PDF). Lecture Notes in Computer Science. Springer Berlin Heidelberg. pp. 317–331. ISBN 9783540385691. doi:10.1007/11839354_23. agents' cardinal (utility-based) preferences are embedded into the space of ordinal preferences. This often gives rise to a distortion in the preferences, and hence in the social welfare of the outcome
  6. ↑ Poundstone, William (2008). Gaming the vote: Why elections aren't fair (and what we can do about it). Hill & Wang. p. 158. ISBN 0809048922. OCLC 276908223. This is the fundamental problem with two-way comparisons. There is no accounting for degrees of preference. ... Cycles result from giving equal weight to unequal preferences. ... The paradox obscures the fact that the voters really do prefer one option.
  7. ↑ Kok, Jan; Shentrup, Clay; Smith, Warren. "Condorcet cycles". RangeVoting.org. Retrieved 2017-02-09. ...any method based just on rank-order votes, fails miserably. Range voting, which allows voters to express strength of preferences, would presumably succeed in choosing the best capital A.
  8. ↑ In this example, the available scores are 0-6, and each voter normalizes their max/min scores to this range, while selecting a score for the middle that is proportional to distance.
  9. ↑ Silver, Charles. "The voting paradox", The Mathematical Gazette 76, November 1992, 387–388.
  10. ↑ Lippman, David (2014). "Voting Theory". Math in society. ISBN 1479276537. OCLC 913874268. There are many Condorcet Methods, which vary primarily in how they deal with ties, which are very common when a Condorcet winner does not exist.

Further reading

This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.