Boundary layer thickness

This page describes some parameters used to measure the properties of boundary layers. Consider a stationary body with a fluid flowing around it, like the semi-infinite flat plate with air flowing over the top of the plate (assume the flow and the plate extends to infinity in the positive/negative direction perpendicular to the $x-y$ plane). At the solid walls of the body the fluid satisfies a no-slip boundary condition and has zero velocity, but as you move away from the wall, the velocity of the flow asymptotically approaches the free stream mean velocity. Therefore, it is impossible to define a sharp point at which the boundary layer becomes the free stream, yet this layer has a well-defined characteristic thickness. The parameters below provide a useful definition of this characteristic, measurable thickness. Also included in this boundary layer description are some parameters useful in describing the shape of the boundary layer.

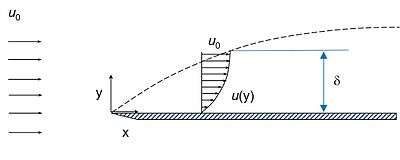
Schematic drawing depicting fluid flow over a flat plate.

99% Boundary Layer thickness

The boundary layer thickness, δ, is the distance across a boundary layer from the wall to a point where the flow velocity has essentially reached the 'free stream' velocity, $u_{0}$ . This distance is defined normal to the wall. It is customarily defined as the point $y$ where:

u(y)=0.99u_{o}

at a point on the wall $x$ . For laminar boundary layers over a flat plate, the Blasius solution to the flow governing equations gives:^[1]

\delta \approx 5.0{\sqrt {{\nu x} \over u_{0}}}

\delta \approx 5.0x/{\sqrt {\mathrm {Re} _{x}}}

For turbulent boundary layers over a flat plate, the boundary layer thickness is given by:^[2]

\delta \approx 0.37x/{\mathrm {Re} _{x}}^{1/5}

where

{\mathrm {Re}}_{x}=\rho u_{0}x/\mu

\delta

is the overall thickness (or height) of the boundary layer

{\mathrm {Re}}_{x}

is the Reynolds number

\rho

is the density

u_{0}

is the freestream velocity

x

is the distance downstream from the start of the boundary layer

\mu

is the dynamic viscosity

\nu =\mu /\rho

is the kinematic viscosity

The turbulent boundary layer thickness formula assumes 1) the flow is turbulent right from the start of the boundary layer and 2) the turbulent boundary layer behaves in a geometrically similar manner (i.e. the velocity profiles are geometrically similar along the flow in the x-direction, differing only by stretching factors in $y$ and $u(y)$ ^[3]). Neither one of these assumptions are true for the general turbulent boundary layer case so care must be excersised in applying this formula.

The velocity thickness can also be referred to as the Soole ratio, although the gradient of the thickness over distance would be adversely proportional to that of velocity thickness.

Displacement thickness

The displacement thickness, δ^* or δ₁ is the distance by which a surface would have to be moved in the direction perpendicular to its normal vector away from the reference plane in an inviscid fluid stream of velocity $u_{0}$ to give the same flow rate as occurs between the surface and the reference plane in a real fluid.^[4]

In practical aerodynamics, the displacement thickness essentially modifies the shape of a body immersed in a fluid to allow an inviscid solution. It is commonly used in aerodynamics to overcome the difficulty inherent in the fact that the fluid velocity in the boundary layer approaches asymptotically to the free stream value as distance from the wall increases at any given location.

The definition of the displacement thickness for compressible flow is based on mass flow rate:

{\delta ^{*}}=\int _{0}^{\infty }{\left(1-{\rho (y)u(y) \over \rho _{0}u_{0}}\right)\,{\mathrm {d}}y}

The definition for incompressible flow can be based on volumetric flow rate, as the density is constant:

{\delta ^{*}}=\int _{0}^{\infty }{\left(1-{u(y) \over u_{0}}\right)\,{\mathrm {d}}y}

where $\rho _{0}$ and $u_{0}$ are the density and velocity in the 'free stream' outside the boundary layer, and $y$ is the coordinate normal to the wall.

For turbulent boundary layer calculations, the time averaged density and velocity at the edge of the boundary layer must be used. In the equations above, $\rho _{0}$ and $u_{0}$ are therefore replaced with $\rho _{e}$ and $u_e$ .

The displacement thickness is used to calculate the boundary layer's shape factor.

Momentum thickness

The momentum thickness, θ or δ₂, is the distance by which a surface would have to be moved parallel to itself towards the reference plane in an inviscid fluid stream of velocity $u_{0}$ to give the same total momentum as exists between the surface and the reference plane in a real fluid.^[5]

The definition of the momentum thickness for compressible flow is based on mass flow rate:

\theta =\int _{0}^{\infty }{{\rho (y)u(y) \over \rho _{0}u_{o}}{\left(1-{u(y) \over u_{o}}\right)}}\,{\mathrm {d}}y

The definition for incompressible flow can be based on volumetric flow rate, as the density is constant:

\theta =\int _{0}^{\infty }{{u(y) \over u_{o}}{\left(1-{u(y) \over u_{o}}\right)}}\,{\mathrm {d}}y

Where $\rho _{0}$ and $u_{0}$ are the density and velocity in the 'free stream' outside the boundary layer, and $y$ is the coordinate normal to the wall.

For a flat plate at zero angle of attack with a laminar boundary layer, the Blasius solution gives.^[6]

\theta \approx 0.664{\sqrt {{\nu x} \over u_{o}}}

The influence of fluid viscosity creates a wall shear stress, $\tau _{w}$ , which extracts energy from the mean flow. The boundary layer can be considered to possess a total momentum flux deficit, due to the frictional dissipation given by:

\rho \int _{0}^{\infty }{u(y)\left(u_{o}-u(y)\right)}\,\mathrm {d} y.

Energy thickness

The energy thickness, $\delta _{3}$ , is the distance by which a surface would have to be moved parallel to itself towards the reference plane in an inviscid fluid stream of velocity $u_{0}$ to give the same total kinetic energy as exists between the surface and the reference plane in a real fluid.^[7]

The definition of the energy thickness for compressible flow is based on mass flow rate:

\delta _{3}=\int _{0}^{\infty }{{\rho (y)u(y) \over \rho _{0}u_{o}}{\left(1-{u(y)^{2} \over u_{o}^{2}}\right)}}\,\mathrm {d} y

The definition for incompressible flow can be based on volumetric flow rate, as the density is constant:

\delta _{3}=\int _{0}^{\infty }{{u(y) \over u_{o}}{\left(1-{u(y)^{2} \over u_{o}^{2}}\right)}}\,\mathrm {d} y

Where $\rho _{0}$ and $u_{0}$ are the density and velocity in the 'free stream' outside the boundary layer, and $y$ is the coordinate normal to the wall.

Shape factor

A shape factor is used in boundary layer flow to determine the nature of the flow.

H = \frac {\delta^*}{\theta}

where H is the shape factor, $\delta^*$ is the displacement thickness and θ is the momentum thickness. The higher the value of H, the stronger the adverse pressure gradient. A high adverse pressure gradient can greatly reduce the Reynolds number at which transition into turbulence may occur.

Conventionally, H = 2.59 (Blasius boundary layer) is typical of laminar flows, while H = 1.3 - 1.4 is typical of turbulent flows.^[8]

Moment Method

A relatively new method^[9]^[10] for describing the thickness and shape of the boundary layer utilizes the moment method commonly used to describe a random variable's probability distribution. The moment method was developed from the observation that the plot of the second derivative of the Blasius boundary layer for laminar flow over a plate looks very much like a Gaussian distribution curve.^[11] It is straightforward to cast the properly scaled velocity profile and its first two derivatives into suitable integral kernels.

The velocity profiles central moments are defined as:

{\zeta _{n}}=\int _{0}^{\infty }{(y-m)^{n}{1 \over \delta ^{*}}(1-{u(y) \over u_{0}})\mathrm {d} y}

where the mean location is given by:

m=\int _{0}^{\infty }{y{1 \over \delta ^{*}}(1-{u(y) \over u_{0}})\mathrm {d} y}

There are some advantages to also include descriptions of moments of the boundary layer profile derivatives with respect to the height above the wall. Consider the first derivative velocity profile central moments given by:

{\kappa _{n}}=\int _{0}^{\infty }{(y-{\delta ^{*}})^{n}{du(y)/u_{0} \over dy}\mathrm {d} y}

where the mean location is the displacement thickness $\delta^*$ .

Finally the second derivative velocity profile central moments are given by:

{\lambda _{n}}=\int _{0}^{\infty }{(y-{\eta })^{n}{d^{2}\{-\eta u(y)/u_{0}\} \over dy^{2}}\mathrm {d} y}

where the mean location is given by:

{\eta }={\mu u_{0} \over \tau _{w}}

With the moments and the mean locations defined, the boundary layer thickness and shape can be described in terms of the boundary layer widths (variance), skewnesses, and excesses (excess kurtosis). Experimentally, it is found that the turbulent boundary layer thickness defined as $\delta _{m}=m+3\sigma _{m}$ where $\sigma _{m}=\zeta _{2}^{1/2}$ , tracks the 99% thickness very well.^[12]

Taking a cue from the boundary layer momentum balance equations, the second derivative boundary layer moments, ${\lambda _{n}}$ track the thickness and shape of that portion of the boundary layer where the viscous forces are significant. Hence the moment method makes it possible to track and quantify the inner viscous region using ${\lambda _{n}}$ moments whereas the outer region of the turbulent boundary layer is tracked using ${\zeta _{n}}$ and ${\kappa _{n}}$ moments.

Calculation of the derivative moments without the need to take derivatives is simplified by using integration by parts to reduce the moments to simply integrals based on the displacement thickness kernel:

{\alpha _{n}}=\int _{0}^{\infty }{y^{n}(1-{u(y) \over u_{0}})\mathrm {d} y}

This means that the first derivative skewness, for example, can be calculated as:

\gamma _{1}=\kappa _{3}/\kappa _{2}^{3/2}=(2\delta ^{*3}-6\delta ^{*}\alpha _{1}+3\alpha _{3})/(-\delta ^{*2}+2\alpha _{1})^{3/2}

This parameter was shown to track the boundary layer shape changes that accompany the laminar to turbulent boundary layer transition.^[13]

Notes

↑ Schlichting, p.140.
↑ Schlichting, p. 638.
↑ Schlichting, p.152.
↑ Schlichting, p. 140.
↑ Schlichting, p. 141.
↑ Schlichting, p. 141.
↑ Rosenhead, Louis, ed. Laminar boundary layers. Clarendon Press, 1963.
↑ Schlichting, p. 454.
↑ Weyburne, 2006.
↑ Weyburne, 2014.
↑ Weyburne, 2006, p. 1678.
↑ Weyburne, 2014, p. 26.
↑ Weyburne, 2014, p. 25.

References

Schlichting, Hermann (1979). Boundary-Layer Theory, 7th ed., McGraw Hill, New York, U.S.A.
Weyburne, David (2006). "A mathematical description of the fluid boundary layer," Applied Mathematics and Computation, vol. 175, pp. 1675–1684
Weyburne, David (2014). "New thickness and shape parameters for the boundary layer velocity profile," Experimental Thermal and Fluid Science, vol. 54, pp. 22–28

This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.

Boundary layer thickness

99% Boundary Layer thickness

Displacement thickness

Momentum thickness

Energy thickness

Shape factor

Moment Method

Further reading

Notes

References