Menger sponge

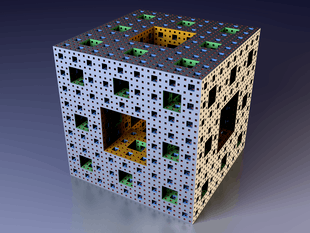
Image 1: An illustration of M4, the sponge after four iterations of the construction process.

In mathematics, the Menger sponge (also known as the Menger universal curve) is a fractal curve. It is a three-dimensional generalization of the Cantor set and Sierpinski carpet, though it is slightly different from a Sierpinski sponge. It was first described by Karl Menger in 1926, in his studies of the concept of topological dimension.[1][2]

Construction

Image 3: A sculptural representation of iterations 0 (bottom) to 3 (top).

The construction of a Menger sponge can be described as follows:

  1. Begin with a cube (Image 2 - first from left).
  2. Divide every face of the cube into 9 squares, like a Rubik's Cube. This will sub-divide the cube into 27 smaller cubes.
  3. Remove the smaller cube in the middle of each face, and remove the smaller cube in the very center of the larger cube, leaving 20 smaller cubes (Image 2 - second from left). This is a level-1 Menger sponge (resembling a Void Cube).
  4. Repeat steps 2 and 3 for each of the remaining smaller cubes, and continue to iterate ad infinitum.

The second iteration gives a level-2 sponge (Image 2 - third from left), the third iteration gives a level-3 sponge (Image 2 - 4th from left), and so on. The Menger sponge itself is the limit of this process after an infinite number of iterations.

Image 2: An illustration of the iterative construction of a Menger sponge up to M3, the third iteration.
Menger sponge animation through (4) recursion steps.

Properties

True view of the cross-section of a level-4 Menger sponge through its centroid and perpendicular to a space diagonal. In this interactive SVG, the cross-sections are true-view and to scale.

The nth stage of the Menger sponge, Mn, is made up of 20n smaller cubes, each with a side length of (1/3)n. The total volume of Mn is thus (20/27)n. The total surface area of Mn is given by the expression 2(20/9)n + 4(8/9)n.[3][4] Therefore the construction's volume approaches zero while its surface area increases without bound. Yet any chosen surface in the construction will be thoroughly punctured as the construction continues, so that the limit is neither a solid nor a surface; it has a topological dimension of 1 and is accordingly identified as a curve.

Each face of the construction becomes a Sierpinski carpet, and the intersection of the sponge with any diagonal of the cube or any midline of the faces is a Cantor set. The cross section of the sponge through its centroid and perpendicular to a space diagonal is a regular hexagon punctured with hexagrams arranged in six-fold symmetry.[5]

The sponge's Hausdorff dimension is log 20/log 3 ≅ 2.727. The Lebesgue covering dimension of the Menger sponge is one, the same as any curve. Menger showed, in the 1926 construction, that the sponge is a universal curve, in that every curve is homeomorphic to a subset of the Menger sponge, where a curve means any compact metric space of Lebesgue covering dimension one; this includes trees and graphs with an arbitrary countable number of edges, vertices and closed loops, connected in arbitrary ways. In a similar way, the Sierpinski carpet is a universal curve for all curves that can be drawn on the two-dimensional plane. The Menger sponge constructed in three dimensions extends this idea to graphs that are not planar, and might be embedded in any number of dimensions.

The Menger sponge is a closed set; since it is also bounded, the Heine–Borel theorem implies that it is compact. It has Lebesgue measure 0. Because it contains continuous paths, it is an uncountable set.

Formal definition

Formally, a Menger sponge can be defined as follows:

where M0 is the unit cube and

MegaMenger

A model of a tetrix viewed through the centre of the Cambridge Level-3 MegaMenger at the 2015 Cambridge Science Festival
One of the MegaMengers, at the University of Bath

MegaMenger is a project aiming to build the largest fractal model, pioneered by Matt Parker of Queen Mary University of London and Laura Taalman of James Madison University. Each small cube is made from 6 interlocking folded business cards, giving a total of 960 000 for a level-four sponge. The outer surfaces are then covered with paper or cardboard panels printed with a Sierpinski carpet design to be more aesthetically pleasing.[6] In 2014, twenty level-three Menger sponges were constructed, which combined would form a distributed level-four Menger sponge.[7]

Similar fractals

Sierpinski-Menger snowflake. Eight corner cubes and the one central cube are kept each time at the lower and lower recursion steps. This peculiar three dimensional fractal has the Hausdorff dimension of the natively two dimensional object like the plane i.e. log 9/log 3=2

See also

Notes and references

  1. ↑ Menger, Karl (1928), Dimensionstheorie, B.G Teubner Publishers
  2. ↑ Menger, Karl (1926), "Allgemeine Räume und Cartesische Räume. I.", Communications to the Amsterdam Academy of Sciences. English translation reprinted in Edgar, Gerald A., ed. (2004), Classics on fractals, Studies in Nonlinearity, Westview Press. Advanced Book Program, Boulder, CO, ISBN 978-0-8133-4153-8, MR 2049443
  3. ↑ Wolfram Demonstrations Project, Volume and Surface Area of the Menger Sponge
  4. ↑ University of British Columbia Science and Mathematics Education Research Group, Mathematics Geometry: Menger Sponge
  5. ↑ Chang, Kenneth (27 June 2011). "The Mystery of the Menger Sponge". Retrieved 8 May 2017 – via NYTimes.com.
  6. ↑ Tim Chartier. "A Million Business Cards Present a Math Challenge". Retrieved 2015-04-07.
  7. ↑ "MegaMenger". Retrieved 2015-02-15.
  8. ↑ Robert Dickau (2014-08-31). "Cross Menger (Jerusalem) Cube Fractal". Robert Dickau. Retrieved 2017-05-08.
  9. ↑ Wade, Lizzie. "Folding Fractal Art from 49,000 Business Cards". Retrieved 8 May 2017.
  10. ↑ W., Weisstein, Eric. "Tetrix". mathworld.wolfram.com. Retrieved 8 May 2017.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.