Fabius function

Graph of the Fabius function on the interval [0,1].

Extension of the function to the nonnegative real numbers.

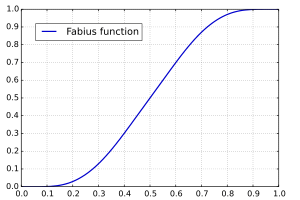
In mathematics, the Fabius function is an example of an infinitely differentiable function that is nowhere analytic, found by Jaap Fabius (1966). It was also written down as the Fourier transform of

by Børge Jessen and Aurel Wintner (1935).

The Fabius function is defined on the unit interval, and is given by the probability distribution of

where the ξ_n are independent uniformly distributed random variables on the unit interval.

This function satisfies the functional equation f ′(x) = 2f (2x) for 0 ≤ x ≤ 1/2; here f ′ denotes the derivative of f. There is a unique extension of f to the nonnegative real numbers which satisfies the same equation. This extension can be defined by f (x + 1) = 1 − f (x) for 0 ≤ x ≤ 1 and f (x + 2^r) = −f (x) for 0 ≤ x ≤ 2^r with r a positive integer. The sequence of intervals within which this function is positive or negative follows the same pattern as the Thue–Morse sequence.

References

• Fabius, J. (1966), "A probabilistic example of a nowhere analytic C ^∞-function", Zeitschrift für Wahrscheinlichkeitstheorie und Verwandte Gebiete, 5: 173–174, MR 0197656, doi:10.1007/bf00536652 
• Jessen, Børge; Wintner, Aurel (1935), "Distribution functions and the Riemann zeta function", Trans. Amer. Math. Soc., 38: 48–88, MR 1501802, doi:10.1090/S0002-9947-1935-1501802-5 
• Dimitrov, Youri (2006). Polynomially-divided solutions of bipartite self-differential functional equations (Thesis). 
• Haugland, Jan Kristian (2016). "Evaluating the Fabius function". arXiv:1609.07999 .