Eratosthenes
| Eratosthenes | |
|---|---|
 | |
| Born |
276 BC Cyrene |
| Died |
194 BC Alexandria |
| Occupation |
|
| Known for | Sieve of Eratosthenes |
Eratosthenes of Cyrene (/ɛrəˈtɒsθəniːz/; Greek: Ἐρατοσθένης ὁ Κυρηναῖος, IPA: [eratostʰénɛːs]; c. 276 BC[1] – c. 195/194 BC[2]) was a Greek mathematician, geographer, poet, astronomer, and music theorist. He was a man of learning, becoming the chief librarian at the Library of Alexandria. He invented the discipline of geography, including the terminology used today.[3]
He is best known for being the first person to calculate the circumference of the Earth, which he did by applying a measuring system using stadia, a standard unit of measure during that time period. His calculation was remarkably accurate. He was also the first to calculate the tilt of the Earth's axis (again with remarkable accuracy). Additionally, he may have accurately calculated the distance from the Earth to the Sun and invented the leap day.[4] He created the first map of the world, incorporating parallels and meridians based on the available geographic knowledge of his era.
Eratosthenes was the founder of scientific chronology; he endeavored to revise the dates of the chief literary and political events from the conquest of Troy. Eratosthenes dated the sack of Troy to 1183 BC. In number theory, he introduced the sieve of Eratosthenes, an efficient method of identifying prime numbers.
He was a figure of influence in many fields. According to an entry[5] in the Suda (a 10th-century reference), his critics scorned him, calling him Beta (the second letter of the Greek alphabet) because he always came in second in all his endeavors.[6] Nonetheless, his devotees nicknamed him Pentathlos after the Olympians who were well rounded competitors, for he had proven himself to be knowledgeable in every area of learning. Eratosthenes yearned to understand the complexities of the entire world.[7]
Life
The son of Aglaos, Eratosthenes was born in 276 BC in Cyrene. Now part of modern-day Libya, Cyrene had been founded by the Greeks centuries earlier and became the capital of Pentapolis (North Africa), a country of five cities: Cyrene, Arsinoe, Berenice, Ptolemias, and Apollonia, Cyrenaica. Alexander the Great conquered Cyrene in 332 BC, and following his death in 323 BC, its rule was given to one of his generals, Ptolemy I Soter, the founder of the Ptolemaic Kingdom. Under Ptolemaic rule the economy prospered, based largely on the export of horses and silphium, a plant used for rich seasoning and medicine.[3] Cyrene became a place of cultivation, where knowledge blossomed. Like any young Greek, Eratosthenes would have studied in the local gymnasium, where he would have learned physical skills and social discourse as well as reading, writing, arithmetic, poetry, and music.[8]
Eratosthenes went to Athens to further his studies. There he was taught Stoicism by its founder, Zeno of Citium, in philosophical lectures on living a virtuous life.[9] He then studied under Ariston of Chios, who led a more cynical school of philosophy. He also studied under the head of the Platonic Academy, who was Arcesilaus of Pitane. His interest in Plato led him to write his very first work at a scholarly level, Platonikos, inquiring into the mathematical foundation of Plato's philosophies.[10] Eratosthenes was a man of many perspectives and investigated the art of poetry under Callimachus.[11] He was a talented and imaginative poet. He wrote poems: one in hexameters called Hermes, illustrating the god's life history; and another, in elegiacs called Erigone, describing the suicide of the Athenian maiden Erigone (daughter of Icarius).[12] He wrote Chronographies, a text that scientifically depicted dates of importance, beginning with the Trojan War. This work was highly esteemed for its accuracy. George Syncellus was later able to preserve from Chronographies a list of 38 kings of the Egyptian Thebes. Eratosthenes also wrote Olympic Victors, a chronology of the winners of the Olympic Games. It is not known when he wrote his works, but they highlighted his abilities.
These works and his great poetic abilities led the pharaoh Ptolemy III Euergetes to seek to place him as a librarian at the Library of Alexandria in the year 245 BC. Eratosthenes, then thirty years old, accepted Ptolemy's invitation and traveled to Alexandria, where he lived for the rest of his life. Within about five years he became Chief Librarian, a position that the poet Apollonius Rhodius had previously held. As head of the library Eratosthenes tutored the children of Ptolemy, including Ptolemy IV Philopator who became the fourth Ptolemaic pharaoh. He expanded the library's holdings: in Alexandria all books had to be surrendered for duplication. It was said that these were copied so accurately that it was impossible to tell if the library had returned the original or the copy. He sought to maintain the reputation of the Library of Alexandria against competition from the Library of Pergamum. Eratosthenes created a whole section devoted to the examination of Homer, and acquired original works of great tragic dramas of Aeschylus, Sophocles and Euripides.[13]
Eratosthenes made several important contributions to mathematics and science, and was a friend of Archimedes. Around 255 BC, he invented the armillary sphere. In On the Circular Motions of the Celestial Bodies, Cleomedes credited him with having calculated the Earth's circumference around 240 BC, using knowledge of the angle of elevation of the Sun at noon on the summer solstice in Alexandria and on Elephantine Island near Syene (modern Aswan, Egypt).
Eratosthenes believed there was good and bad in every nation and criticized Aristotle for arguing that humanity was divided into Greeks and barbarians, and that the Greeks should keep themselves racially pure.[14] As he aged he contracted ophthalmia, becoming blind around 195 BC. Losing the ability to read and to observe nature plagued and depressed him, leading him to voluntarily starve himself to death. He died in 194 BC at 82 in Alexandria.[15]
Measurement of the Earth's circumference
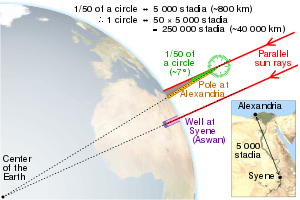
Eratosthenes calculated the circumference of the Earth without leaving Egypt. He knew that at local noon on the summer solstice in Syene (modern Aswan, Egypt), the Sun was directly overhead. (Syene is at latitude 24°05′ North, near to the Tropic of Cancer, which was 23°42′ North in 100 BC[16]) He knew this because the shadow of someone looking down a deep well at that time in Syene blocked the reflection of the Sun on the water. He measured the Sun's angle of elevation at noon on the same day in Alexandria. The method of measurement was to make a scale drawing of that triangle which included a right angle between a vertical rod and its shadow. This turned out to be about 7°, or 1/50th of the way around a circle. Taking the Earth as spherical, and knowing both the distance and direction of Syene, he concluded that the Earth's circumference was fifty times that distance.
His knowledge of the size of Egypt was founded on the work of many generations of surveying trips. Pharaonic bookkeepers gave a distance between Syene and Alexandria of 5,000 stadia (a figure that was checked yearly).[17] Some say that the distance was corroborated by inquiring about the time that it took to travel from Syene to Alexandria by camel. Carl Sagan said that Eratosthenes paid a man to walk and measure the distance. Some claim Eratosthenes used the Olympic stade of 176.4 m, which would imply a circumference of 44,100 km, an error of 10%,[17] but the 184.8 m Italian stade became (300 years later) the most commonly accepted value for the length of the stade,[17] which implies a circumference of 46,100 km, an error of 15%.[17] It was unlikely, even accounting for his extremely primitive measuring tools, that Eratosthenes could have calculated an accurate measurement for the circumference of the Earth. He made five important assumptions (none of which is perfectly accurate):[17][18]
- That the distance between Alexandria and Syene was 5000 stadia,
- That Alexandria is due north of Syene
- That Syene lies on the Tropic of Cancer
- That the Earth is a perfect sphere.
- That light rays emanating from the Sun are parallel.
Eratosthenes later rounded the result to a final value of 700 stadia per degree, which implies a circumference of 252,000 stadia, likely for reasons of calculation simplicity as the larger number is evenly divisible by 60.[17] In 2012, Anthony Abreu Mora repeated Eratosthenes' calculation with more accurate data; the result was 40,074 km, which is 66 km different (0.16%) from the currently accepted polar circumference of the Earth.[18]
Seventeen hundred years after Eratosthenes' death, while Christopher Columbus studied what Eratosthenes had written about the size of the Earth, he chose to believe, based on a map by Toscanelli, that the Earth's circumference was one-third smaller. Had Columbus set sail knowing that Eratosthenes' larger circumference value was more accurate, he would have known that the place that he made landfall was not Asia, but rather the New World.[19]
"Father of geography"
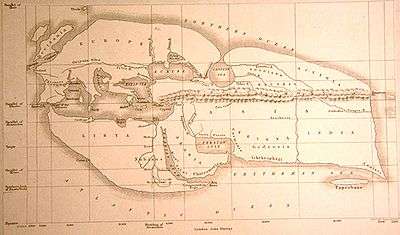
Eratosthenes now continued from his knowledge about the Earth. Using his discoveries and knowledge of its size and shape, he began to sketch it. In the Library of Alexandria he had access to various travel books, which contained various items of information and representations of the world that needed to be pieced together in some organized format.[20] In his three-volume work Geography (Greek: Geographika), he described and mapped his entire known world, even dividing the Earth into five climate zones:[21] two freezing zones around the poles, two temperate zones, and a zone encompassing the equator and the tropics.[22] He had invented geography. He created terminology that is still used today. He placed grids of overlapping lines over the surface of the Earth. He used parallels and meridians to link together every place in the world. It was now possible to estimate one's distance from remote locations with this network over the surface of the Earth. In the Geography the names of over 400 cities and their locations were shown: this had never been achieved before.[3] Unfortunately, his Geography has been lost to history, but fragments of the work can be pieced together from other great historians like Pliny, Polybius, Strabo, and Marcianus.
- The first book was something of an introduction and gave a review of his predecessors, recognizing their contributions that he compiled in the library. In this book Eratosthenes denounced Homer as not providing any insight into what he now described as geography. His disapproval of Homer's topography angered many who believed the world depicted in the Odyssey to be legitimate.[7][23] He also commented on the ideas of the nature and origin of the Earth: he had thought of Earth as an immovable globe; while on its surface was a place that was changing. He had hypothesized that at one time the Mediterranean was a vast lake that covered the countries that surrounded it; and had only become connected to the ocean to the west when a passage had opened up sometime in its history.
- In the second book is his discovery about the circumference of the Earth. This is where, according to Pliny, "The world was grasped." Eratosthenes described his famous story of the well in Syene, described above. This book would now be considered a text on mathematical geography.
- His third book of the Geography contained political geography. He cited countries and used parallel lines to divide the map into sections, to give accurate descriptions of the realms. This was a breakthrough, and can be considered the beginning of geography.[24]
Achievements
Eratosthenes was described by the Suda Lexicon as a Πένταθλος (Pentathlos) which can be translated as "All-Rounder", for he was skilled in a variety of things: He was a true polymath. He was nicknamed Beta because he was great at many things and tried to get his hands on every bit of information but never achieved the highest rank in anything; Strabo accounts Eratosthenes as a mathematician among geographers and a geographer among mathematicians.[25]
- Eusebius of Caesarea in his Preparatio Evangelica includes a brief chapter of three sentences on celestial distances (Book XV, Chapter 53). He states simply that Eratosthenes found the distance to the Sun to be "σταδίων μυριάδας τετρακοσίας καὶ ὀκτωκισμυρίας" (literally "of stadia myriads 400 and 80,000") and the distance to the Moon to be 780,000 stadia. The expression for the distance to the Sun has been translated either as 4,080,000 stadia (1903 translation by E. H. Gifford), or as 804,000,000 stadia (edition of Edouard des Places, dated 1974–1991). The meaning depends on whether Eusebius meant 400 myriad plus 80,000 or "400 and 80,000" myriad. With a stade of 185 m, 804,000,000 stadia is 149,000,000 km, approximately the distance from the Earth to the Sun.
- Eratosthenes also calculated the Sun's diameter. According to Macrobious, Eratosthenes made the diameter of the Sun to be about 27 times that of the Earth.[24] The actual figure is approximately 109 times.[26]
- During his time at the Library of Alexandria, Eratosthenes devised a calendar using his predictions about the ecliptic of the Earth. He calculated that there are 365 days in a year and that every fourth year there would be 366 days.[27]
- He was also very proud of his solution for Doubling the Cube. His motivation was that he wanted to produce catapults. Eratosthenes constructed a mechanical line drawing device to calculate the cube, called the mesolabio. He dedicated his solution to King Ptolemy, presenting a model in bronze with it a letter and an epigram.[28] Archimedes was Eratosthenes' friend and he, too, worked on the war instrument with mathematics. Archimedes dedicated his book The Method to Eratosthenes, knowing his love for learning and mathematics.[29]
Prime numbers

Eratosthenes proposed a simple algorithm for finding prime numbers. This algorithm is known in mathematics as the Sieve of Eratosthenes.
In mathematics, the sieve of Eratosthenes (Greek: κόσκινον Ἐρατοσθένους), one of a number of prime number sieves, is a simple, ancient algorithm for finding all prime numbers up to any given limit. It does so by iteratively marking as composite, i.e., not prime, the multiples of each prime, starting with the multiples of 2. The multiples of a given prime are generated starting from that prime, as a sequence of numbers with the same difference, equal to that prime, between consecutive numbers. This is the sieve's key distinction from using trial division to sequentially test each candidate number for divisibility by each prime.
Works
Eratosthenes was one of the most pre-eminent scholarly figures of his time, and produced works covering a vast area of knowledge before and during his time at the Library. He wrote on many topics — geography, mathematics, philosophy, chronology, literary criticism, grammar, poetry, and even old comedies. Unfortunately, there are only fragments left of his works after the Destruction of the Library of Alexandria.[30]
Titles
- Platonikos
- Hermes
- Erigone
- Chronographies
- Olympic Victors
- Περὶ τῆς ἀναμετρήσεως τῆς γῆς (On the Measurement of the Earth)[31] (lost, summarized by Cleomedes)
- Гεωγραϕικά (Geographika)[32] (lost, criticized by Strabo)
- Arsinoe (a memoir of queen Arsinoe; lost; quoted by Athenaeus in the Deipnosophistae)
- Ariston (concerning Aristo of Chios' addiction to luxury); lost; quoted by Athenaeus in the Deipnosophistae)[33]
- A fragmentary collection of Hellenistic myths about the constellations, called Catasterismi (Katasterismoi), was attributed to Eratosthenes, perhaps to add to its credibility.
See also
Eponyms
- Eratosthenes (crater) on the Moon
- Eratosthenian period in the lunar geologic timescale
- Eratosthenes Seamount in the eastern Mediterranean Sea
References
- ↑ The Suda states that he was born in the 126th Olympiad, (276–272 BC). Strabo (Geography, i.2.2), though, states that he was a "pupil" (γνωριμος) of Zeno of Citium (who died 262 BC), which would imply an earlier year-of-birth (c. 285 BC) since he is unlikely to have studied under him at the young age of 14. However, γνωριμος can also mean "acquaintance", and the year of Zeno's death is by no means definite. Cf. Eratosthenes entry in the Dictionary of Scientific Biography (1971)
- ↑ The Suda states he died at the age of 80, Censorinus (De die natali, 15) at the age of 81, and Pseudo-Lucian (Makrobioi, 27) at the age of 82.
- 1 2 3 Roller, Duane W. Eratosthenes' Geography. New Jersey: Princeton University Press, 2010.
- ↑ Alfred, Randy (June 19, 2008). "June 19, 240 B.C.: The Earth Is Round, and It's This Big". Wired. Retrieved 2013-06-22.
- ↑ Entry ε 2898
- ↑ See also Asimov, Isaac. Asimov's Biographical Encyclopedia of Science and Technology, new revised edition. 1975. Entry #42, "Eratosthenes", Page 29. Pan Books Ltd, London. ISBN 0-330-24323-3. This was also asserted by Carl Sagan 31 minutes into his Cosmos episode The Shores of the Cosmic Ocean
- 1 2 Chambers, James T. "Eratosthenes of Cyrene." Dictionary Of World Biography: The Ancient World (January 1998): 1–3.
- ↑ Bailey, Ellen. 2006. "Eratosthenes of Cyrene." Eratosthenes Of Cyrene 1–3. Book Collection Nonfiction: High School Edition.
- ↑ Rist, J.M. "Zeno and Stoic Consistency," in Phronesis. Vol. 22, No. 2, 1977.
- ↑ Chambers, James T. "Eratosthenes of Cyrene." in Dictionary Of World Biography: The Ancient World January 1998: 1–3.
- ↑ Bailey, Ellen. "Eratosthenes of Cyrene," in Eratosthenes of Cyrene 1–3. Book Collection Nonfiction: High School Edition, 2006.
- ↑ Chambers, James T. "Eratosthenes of Cyrene" in Dictionary Of World Biography: The Ancient World (January 1998): 1–3.
- ↑ Chambers, James T. "Eratosthenes of Cyrene" Dictionary Of World Biography: The Ancient World, January 1998.
- ↑
- p439 Vol. 1 William Woodthorpe Tarn Alexander the Great. Vol. I, Narrative; Vol. II, Sources and Studies0. Cambridge: Cambridge University Press, 1948. (New ed., 2002 (paperback, ISBN 0-521-53137-3)).
- ↑ Bailey, Ellen. "Eratosthenes of Cyrene." Eratosthenes Of Cyrene (January 2006): 1–3.
- ↑ Balasubramaniam, R. (10 August 2017). "Story of the Delhi Iron Pillar". Foundation Books – via Google Books.
- 1 2 3 4 5 6 "Eratosthenes and the Mystery of the Stades - How Long Is a Stade? - Mathematical Association of America". www.maa.org.
- 1 2 "How did Eratosthenes measure the circumference of the earth?". 3 July 2012.
- ↑ Gow, Mary. "Measuring the Earth: Eratosthenes and His Celestial Geometry, p. 6 (Berkeley Heights, NJ: Enslow, 2010).
- ↑ Smith, Sir William. "Eratosthenes", in A Dictionary of Greek and Roman Biography and Mythology. Ann Arbor, Michigan: University of Michigan Library, 2005.
- ↑ Morris, Terry R. "Eratosthenes of Cyrene." in Encyclopedia Of The Ancient World. November 2001.
- ↑ 2011. "Eratosthenes." Hutchinson's Biography Database 1.
- ↑ Eckerman, Chris. Review of (D.W.) Roller 'Eratosthenes' Geography. Fragments Collected and Translated, with Commentary and Additional Material. The Classical Review. 2011.
- 1 2 Smith, Sir William. "Eratosthenes," in A Dictionary of Greek and Roman Biography and Mythology. Ann Arbor, Michigan: University of Michigan Library, 2005.
- ↑ Dicks, D.R. "Eratosthenes," in Complete Dictionary of Scientific Biography. New York: Charles Scribner's Sons, 1971.
- ↑ "Ask an Astronomer". Cool Cosmos.
- ↑ Greek Scholar's Work Shows Usefulness of Measurement." Manawatu Standard, June 19, 2012., 07, Newspaper Source Plus
- ↑ Zhumud, Leonid. Plato as "Architect of Science". in Phonesis. Vol. 43 (3) 1998. 211–244.
- ↑ Chondros, Thomas G. Archimedes Life Works and Machines. in Mechanism and Machine Theory. Vol.45(11) 2010. 1766–1775.
- ↑ Dicks, D.R. "Eratosthenes", in Complete Dictionary of Scientific Biography. New York: Charles Scribner's Sons, 1971.
- ↑ Mentioned by Hero of Alexandria in his Dioptra. See p. 272, vol. 2, Selections Illustrating the History of Greek Mathematics, tr. Ivor Thomas, London: William Heinemann Ltd.; Cambridge, MA: Harvard University Press, 1957.
- ↑ Dicks, D.R. "Eratosthenes," in Complete Dictionary of Scientific Biography. New York: Charles Scribner's Sons, 1971.
- ↑ Smith, Andrew. "Athenaeus: Deipnosophists - Book 7". www.attalus.org.
Further reading
- Aujac, G. (2001). Eratosthène de Cyrène, le pionnier de la géographie. Paris: Édition du CTHS. 224p.
- Bulmer-Thomas, Ivor (1939–1940). Selections Illustlating the History of Greek Mathematics. Cambridge, MA: Harvard University Press.
- Diller, A (1934). "Geographical Latitudes in Eratosthenes, Hipparchus and Posidonius". Klio. 27 (3): 258–269.
- Dorofeeva, A. V. (1988). "Eratosthenes (ca. 276–194 B.C.)". Mat. V Shkole (in Russian) (4): i.
- Dutka, J. (1993). "Eratosthenes' measurement of the Earth reconsidered". Arch. Hist. Exact Sci. 46 (1): 55–66. doi:10.1007/BF00387726.
- El'natanov, B. A. (1983). "A brief outline of the history of the development of the sieve of Eratosthenes". Istor.-Mat. Issled. (in Russian). 27: 238–259.
- Fischer, I (1975). "Another look at Eratosthenes' and Posidonius' determinations of the Earth's circumference". Quarterly Journal of the Royal Astronomical Society. 16: 152–167. Bibcode:1975QJRAS..16..152F.
- Fowler, D. H.; Rawlins, Dennis (1983). "Eratosthenes' ratio for the obliquity of the ecliptic". Isis. 74 (274): 556–562. doi:10.1086/353361.
- Fraser, P. M. (1970). "Eratosthenes of Cyrene". Proceedings of the British Academy. 56: 175–207.
- Fraser, P. M. (1972). Ptolemaic Alexandria. Oxford: Clarendon Press.
- Fuentes González, P. P., "Ératosthène de Cyrène", in R. Goulet (ed.), Dictionnaire des Philosophes Antiques, vol. III, Paris, Centre National de la Recherche Scientifique, 2000, pp. 188–236.
- Geus K. (2002). Eratosthenes von Kyrene. Studien zur hellenistischen Kultur- und Wissenschaftgeschichte. München: Verlag C.H. Beck. (Münchener Beiträge zur Papyrusforschung und antiken Rechtsgeschichte. Bd. 92) X, 412 S.
- Goldstein, B. R. (1984). "Eratosthenes on the "measurement" of the Earth". Historia Math. 11 (4): 411–416. doi:10.1016/0315-0860(84)90025-9.
- Gulbekian, E. (1987). "The origin and value of the stadion unit used by Eratosthenes in the third century B.C". Archive for History of Exact Sciences. 37 (4): 359–363. doi:10.1007/BF00417008.
- Honigmann, E. (1929). Die sieben Klimata und die πολεις επισημοι. Eine Untersuchung zur Geschichte der Geographie und Astrologie in Altertum und Mittelalter. Heidelberg: Carl Winter's Universitätsbuchhandlung. 247 S.
- Knaack, G. (1907). "Eratosthenes". Pauly–Wissowa VI: 358–388.
- Manna, F. (1986). "The Pentathlos of ancient science, Eratosthenes, first and only one of the "primes"". Atti Accad. Pontaniana (N.S.) (in Italian). 35: 37–44.
- Muwaf, A.; Philippou, A. N. (1981). "An Arabic version of Eratosthenes writing on mean proportionals". J. Hist. Arabic Sci. 5 (1–2): 147–174.
- Nicastro, Nicholas (2008). Circumference: Eratosthenes and the ancient quest to measure the globe. New York: St. Martin's Press. ISBN 0-312-37247-7.
- O'Connor, John J.; Robertson, Edmund F., "Eratosthenes", MacTutor History of Mathematics archive, University of St Andrews.
- Marcotte, D. (1998). "La climatologie d'Ératosthène à Poséidonios: genèse d'une science humaine". G. Argoud, J.Y. Guillaumin (eds.). Sciences exactes et sciences appliquées à Alexandrie (IIIe siècle av J.C. – Ier ap J.C.). Saint Etienne: Publications de l'Université de Saint Etienne: 263–277.
- McPhail, Cameron (2011). Reconstructing Eratosthenes' Map of the World: a Study in Source Analysis. A Thesis Submitted for the Degree of Master of Arts at the University of Otago. Dunedin, New Zealand.
- Pfeiffer, Rudolf (1968). History of Classical Scholarship From the Beginnings to the End of the Hellenistic Age. Oxford: Clarendon Press.
- Rawlins, D. (1982). "Eratosthenes' geodesy unraveled : was there a high-accuracy Hellenistic astronomy". Isis. 73 (2): 259–265. doi:10.1086/352973.
- Rawlins, D. (1982). "The Eratosthenes – Strabo Nile map. Is it the earliest surviving instance of spherical cartography? Did it supply the 5000 stades arc for Eratosthenes' experiment?". Arch. Hist. Exact Sci. 26 (3): 211–219.
- Rawlins, D. (2008). "Eratosthenes's large Earth and tiny universe" (PDF). DIO. 14: 3–12.
- Roller, Duane W. (2010). Eratosthenes' Geography: Fragments collected and translated, with commentary and additional material. Princeton: Princeton University Press. ISBN 978-0-691-14267-8.
- Shcheglov, D.A. (2004/2006). "Ptolemy's System of Seven Climata and Eratosthenes' Geography". Geographia Antiqua 13: 21–37.
- Shcheglov, D.A. (2006). "Eratosthenes' Parallel of Rhodes and the History of the System of Climata". Klio. 88: 351–359. doi:10.1524/klio.2006.88.2.351.
- Strabo (1917). The Geography of Strabo. Horace Leonard Jones, trans. New York: Putnam.
- Taisbak, C. M. (1984). "Eleven eighty-thirds. Ptolemy's reference to Eratosthenes in Almagest I.12". Centaurus. 27 (2): 165–167. Bibcode:1984Cent...27..165T. doi:10.1111/j.1600-0498.1984.tb00766.x.
- Thalamas, A. (1921). La géographe d'Ératosthène. Versailles.
- Wolfer, E. P. (1954). Eratosthenes von Kyrene als Mathematiker und Philosoph. Groningen-Djakarta.
External links
| Wikimedia Commons has media related to Eratosthenes. |
| Wikisource has original works written by or about: Eratosthenes |
- English translation of the primary source for Erastothenes and the size of the earth at Roger Pearse.
- Bernhardy, Gottfried: Eratosthenica Berlin, 1822 (PDF) (Latin/Greek), Reprinted Osnabruck 1968 (German)
- Eratosthenes' sieve in Javascript
- About Eratosthenes' methods, including a Java applet
- How the Greeks estimated the distances to the Moon and Sun
- Measuring the Earth with Eratosthenes' method
- List of ancient Greek mathematicians and contemporaries of Eratosthenes
- New Advent Encyclopedia article on the Library of Alexandria
- Eratosthenes' sieve in classic BASIC all-web based interactive programming environment
- Following in the footsteps of Eratosthenes : project fr:La main à la pâte.
- Open source Physics Computer Model about Eratosthenes estimation of radius and circumference of Earth
- Eratosthenes, video
| Preceded by Apollonius of Rhodes |
Head of the Library of Alexandria | Succeeded by Aristophanes of Byzantium |