Doubochinski's pendulum

Doubochinski’s pendulum is a classical oscillator interacting with a high-frequency field in such a way that the oscillator takes on a discrete set of stable regimes of oscillation, each at a frequency near to the proper frequency of the oscillator, but each with a distinct, "quantized" amplitude. [1] [2] [3] [4] [5] [6] [7] The phenomenon of amplitude quantization in this sort of coupled system was first discovered by the brothers Danil and Yakov Doubochinski in 1968–69.
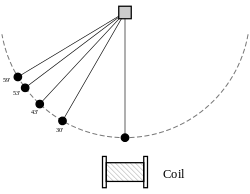
A simple demonstration apparatus consists of a mechanical pendulum interacting with a magnetic field (Fig. 1). [1] [2] [3] [4] [8] [9] [10] [11] [12] [13] [14]
The system is composed of two interacting oscillatory processes: (1) a pendulum arm with a natural frequency on the order of 0.5–1 Hz, with a small permanent magnet fixed at its moving end; and (2) a stationary electromagnet (solenoid) positioned under the equilibrium point of the pendulum’s trajectory and supplied with alternating current of fixed frequency, typically in the range of 10–1000 Hz. The mechanical pendulum arm and solenoid are configured in such a way, that the pendulum arm interacts with the oscillating magnetic field of the solenoid only over a limited portion of its trajectory – the so-called "zone of interaction" – outside of which the strength of the magnetic field drops off rapidly to zero. This spatial inhomogeniety of the interaction is key to the quantized behavior and other unusual properties of the system.
When released at any arbitrary starting position, the pendulum’s motion evolves into one of a discrete set of stable oscillation modes, having sharply differing amplitudes, but approximately the same period of oscillation – close to the pendulum’s undisturbed period (Fig. 2). In each such mode, the energy lost by friction in the pendulum’s motion, is compensated by an average net energy transfer from the oscillating magnetic field, in a self-regulating manner. [3] [4] [5] [11] [12] [15] [16] [17] [18] The stability of each amplitude-mode is maintained by a constant self-adjustment of the phase relationship between the pendulum and the high-frequency field. Through its interaction with the field, the pendulum extracts the amount of energy needed to compensate its frictional losses for a given period. The pendulum compensates for the changes in the strength of the magnetic field, [13] [14] [19] [20] by slightly shifting the phase of its entry into the zone of interaction, while maintaining almost exactly the same amplitude and frequency. The values of the quantized amplitudes – and the corresponding energies of the quantized modes – are essentially independent of the strength of the alternating current supplied to the electromagnet, over a very large range. The higher the applied frequency, the larger the array of stable modes that become accessible to the pendulum (See Table 1).
| Frequency of the magnetic field (Hz) | Pendulum amplitudes | |||||||
| 5 | 68° | |||||||
| 20 | 30° | 59° | 74° | 85° | ||||
| 50 | 30° | 43° | 53° | 59° | 68° | 74° | 80° | 85° |
References
- 1 2 L.A.Vainshtein; Ya.B.Doubochinski (1978). "On the low-frequency oscillations under the influence of high-frequency force". Zh. Tekh. Fiz [Sov. Phys.-Tech. Phys]. 48. [23] (1494): 1321 [745].
- 1 2 D.B.Doubochinski; Ya.B.Duboshinsky; et al. (1979). "Discrete modes of a system subject to an inhomogeneous high-frequency force". Zh. Tech. Fiz [Sov. Phys.-Tech. Phys]. 49. [24]: 1160 [642].
- 1 2 3 P.S.Landa (2001). Nonlinear Oscillations and Waves in Dynamical Systems (PDF). Kluwer Academic Publishers. p. 307.
- 1 2 3 J.Tennenbaum (Winter 2005). "Amplitude Quantization as an Elementary Property of Macroscopic. Vibrating Systems" (PDF). 21st Century Science & Technology.
- 1 2 Weldon J. Wilson (2010). "Amplitude Quantization as a Fundamental Property of Coupled Oscillator Systems". Professor of Engineering and Physics, Weldon Wilson's Home Page.
- ↑ Weldon J.Wilson (2012). "Senior Engineering Design Possible Projects List, 2011-2012" (PDF). Professor of Engineering and Physics, Weldon Wilson's Home Page.
- ↑ D.B.Doubochinski; J.Tennenbaum (23 April 2013). Theory and applications of the macroscopic quantization effect in nonlinearly-coopled vibrating systems. 1st Euro-Mediterranean Conference on Structural Dynamics and Vibroacoustics. Marrakech, Morocco: MEDYNA 2013.
- ↑ D.B.Doubochinski; Ya.B.Doubochinski (1982). "Wave excitation of an oscillator having a discrete series of stable amplitudes". Dokl. Akad. Nauk SSSR [Sov. Phys. Doklady]. 3. [27]: 605 [564].
- ↑ D.I.Penner; D.B.Duboshinskii (1973). "Asynchronous excitation of undamped oscillations". Soviet Physics Uspekhi. 16 (1): 158–160. doi:10.1070/PU1973v016n01ABEH005156.
- ↑ V.N.Damgov; D.B.Duboshinskii; Ia.B.Duboshinskii (1986). "The excitation of undamped oscillations with a discrete series of stable amplitudes". Bolgarskaia Akademiia Nauk, Doklady (in Russian). 39 (9): 47–50. Bibcode:1986BlDok..39...47D.
- 1 2 V.Damgov; I.Popov (1989). ""Discrete" Oscillations and Multiple Attractors in Kick-excited Systems" (PDF) (Discrete Dynamics in Nature and Society): 2, 3, 25, 26.
- 1 2 D.B.Doubochinski; Ya.B.Doubochinski (1991). "Amorcage argumen-taire d’oscillations entretenues avec une serie discrete d’amplitudes stable". EDF Bulletin de la direction des etudes et recherches, serie C, Mathematiques, Informatique: 11–20.
- 1 2 Martin Beech (2014). The Pendulum Paradigm: Variations on a Theme and the Measure of Heaven and Earth. Universal Publishers. p. 290.
- 1 2 "Preparation to the Young Physicists’ Tournaments’ 2015" (PDF). 2014.
- ↑ P.S.Landa; Y.B.Duboshinskii (1989). "Self-oscillatory systems with high-frequency energy sources". Turpion Limited.
- ↑ V.N.Damgov; D.B.Douboshinsky (March 1992). "The wave nature and dynamical quantization of the solar system". An International Journal of Solar System Science. 56 (3): 233–242. doi:10.1007/bf00116289.
- ↑ "Doubochinski’s site".
- ↑ D.B.Doubochinski; J.Tennenbaum (2012). New Physical Effect Permits Factor-of-Ten Reduction in Energy Requirements for Cooling. (PDF). International Conference on Advanced Material and Manufacturing Science. ICAMMS 2012. p. M1191.
- ↑ Doubochinski Danil B.; Tennenbaum Jonathan (2014). "New Physical Effect Permits Factor-of-Ten Reduction in EnergyRequirements for Cooling.". Advanced Materials Research. Trans Tech Publications, Switzerland. 875-877: 1842–1846. doi:10.4028/www.scientific.net/AMR.875-877.1842.
- ↑ Doubochinski Danil; Tennenbaum Jonathan (June 2015). "A New Dynamical Conception of Physical Objects and Their Interactions.". Quantum Matter. American Scientific Publishers. 4 (3): 251–257. doi:10.1166/qm.2015.1281.
- D.B.Doubochinsky; J.B.Doubochinsky; V.N.Damgov (1987). "Energetics of the excitation of stable oscillations by a coordinate-nonlinear periodic force.". Comptes-rendus de l'Académie Bulgare des Sciences. 40 (4): 57.
- D.Doubochinski; J.Tennenbaum (2007). "The Macroscopic Quantum Effect in Nonlinear Oscillating Systems: a Possible Bridge between Classical and Quantum Physics". arXiv:0711.4892
 .
.
- D.Doubochinski; J.Tennenbaum (2008). "On the Fundamental Properties of Coupled Oscillating Systems". arXiv:0712.2575
 .
.
- D.Doubochinski; J.Tennenbaum (2008). "On the General Nature of Physical Objects and their Interactions, as Suggested by the Properties of Argumentally-Coupled Oscillating Systems". arXiv:0808.1205
 .
.
- Danil Doubochinski (24 August 2015). Doubochinski’s reverse-parametrical pendulum and motor. 22eme Congres Francais de Mecanique. Lyon, France: CFM2015.