Insect flight

Insects are the only group of invertebrates that have evolved wings and flight. Two insect groups, the dragonflies and the mayflies, have flight muscles attached directly to the wings. Other insects have the flight muscles attached to the thorax, making it oscillate and indirectly causing the wings to beat. Some very small insects make use not of steady-state aerodynamics but of the Weis-Fogh clap and fling mechanism, generating large lift forces at the expense of wear and tear on the wings. Many insects can hover, maintaining height and controlling their position. Some insects such as moths have the forewings coupled to the hindwings so these can work in unison.
Insects first flew in the Carboniferous, some 350 million years ago. Wings may have evolved from appendages on the sides of existing limbs, which already had nerves, joints, and muscles used for other purposes. These may initially have been used for sailing on water, or to slow the rate of descent when gliding.
Mechanisms
Direct flight


Unlike other insects, the wing muscles of the Ephemeroptera (Mayflies) and Odonata (dragonflies and damselflies) insert directly at the wing bases, which are hinged so that a small movement of the wing base downward, lifts the wing itself upward, very much like rowing through the air. Dragonflies and damselflies have fore and hind wings similar in shape and size. Each operates independently, which gives a degree of fine control and mobility in terms of the abruptness with which they can change direction and speed, not seen in other flying insects. This is not surprising, given that odonates are all aerial predators, and they have always hunted other airborne insects.[1]
Indirect flight


Other than the two orders with direct flight muscles, all other living winged insects fly using a different mechanism, involving indirect flight muscles. This mechanism evolved once, and is the defining feature (synapomorphy) for the infraclass Neoptera; it corresponds, probably not coincidentally, with the appearance of a wing-folding mechanism, which allows Neopteran insects to fold the wings back over the abdomen when at rest (though this ability has been lost secondarily in some groups, such as in the butterflies).[1]
In the higher groups with two functional pairs of wings, both pairs are linked together mechanically in various ways, and function as a single wing, although this is not true in the more primitive groups. There are also exceptions to be found among the more advanced Neoptera; the ghost moth is able to unlock its pair of wings and move them independently, allowing them to hover like dragonflies.[2] What all Neoptera share, however, is the way the muscles in the thorax work: these muscles, rather than attaching to the wings, attach to the thorax and deform it; since the wings are extensions of the thoracic exoskeleton, the deformations of the thorax cause the wings to move as well. A set of dorsal longitudinal muscles compress the thorax from front to back, causing the dorsal surface of the thorax (notum) to bow upward, making the wings flip down. A set of tergosternal muscles pull the notum downward again, causing the wings to flip upward. [1][3] In a few groups, the downstroke is accomplished solely through the elastic recoil of the thorax when the tergosternal muscles are relaxed. Several small sclerites at the wing base have other, separate, muscles attached and these are used for fine control of the wing base in such a way as to allow various adjustments in the tilt and amplitude of the wing beats. One of the final refinements that has appeared in some of the higher Neoptera (Coleoptera, Diptera, and Hymenoptera) is a type of muscular or neural control system whereby a single nerve impulse causes a muscle fiber to contract multiple times; this allows the frequency of wing beats to exceed the rate at which the nervous system can send impulses. This specialized form of muscle is termed asynchronous muscle. The overall effect is that many higher Neoptera can beat their wings much faster than insects with direct flight muscles.[1]
Aerodynamics
There are two basic aerodynamic models of insect flight: creating a leading edge vortex, and using clap and fling.
Leading edge vortex
Most insects use a method that creates a spiralling leading edge vortex. These flapping wings move through two basic half-strokes. The downstroke starts up and back and is plunged downward and forward. Then the wing is quickly flipped over (supination) so that the leading edge is pointed backward. The upstroke then pushes the wing upward and backward. Then the wing is flipped again (pronation) and another downstroke can occur. The frequency range in insects with synchronous flight muscles typically is 5 to 200 hertz (Hz). In those with asynchronous flight muscles, wing beat frequency may exceed 1000 Hz. When the insect is hovering, the two strokes take the same amount of time. A slower downstroke, however, provides thrust.[4][5]
Identification of major forces is critical to understanding insect flight. The first attempts to understand flapping wings assumed a quasi-steady state. This means that the air flow over the wing at any given time was assumed to be the same as how the flow would be over a non-flapping, steady-state wing at the same angle of attack. By dividing the flapping wing into a large number of motionless positions and then analyzing each position, it would be possible to create a timeline of the instantaneous forces on the wing at every moment. The calculated lift was found to be too small by a factor of three, so researchers realized that there must be unsteady phenomena providing aerodynamic forces. There were several developing analytical models attempting to approximate flow close to a flapping wing. Some researchers predicted force peaks at supination. With a dynamically scaled model of a fruit fly, these predicted forces later were confirmed. Others argued that the force peaks during supination and pronation are caused by an unknown rotational effect that fundamentally is different from the translational phenomena. There is some disagreement with this argument. Through computational fluid dynamics, some researchers argue that there is no rotational effect. They claim that the high forces are caused by an interaction with the wake shed by the previous stroke.[4][5]
Similar to the rotational effect mentioned above, the phenomena associated with flapping wings are not completely understood or agreed upon. Because every model is an approximation, different models leave out effects that are presumed to be negligible. For example, the Wagner effect says that circulation rises slowly to its steady-state due to viscosity when an inclined wing is accelerated from rest. This phenomenon would explain a lift value that is less than what is predicted. Typically, the case has been to find sources for the added lift. It has been argued that this effect is negligible for flow with a Reynolds number that is typical of insect flight. The Wagner effect was ignored, consciously, in at least one recent model.[5] One of the most important phenomena that occurs during insect flight is leading edge suction. This force is significant to the calculation of efficiency. The concept of leading edge suction first was put forth to describe vortex lift on sharp-edged delta wings. At high angles of attack, the flow separates over the leading edge, but reattaches before reaching the trailing edge. Within this bubble of separated flow is a vortex. Because the angle of attack is so high, a lot of momentum is transferred downward into the flow. These two features create a large amount of lift force as well as some additional drag. The important feature, however, is the lift. Because the flow has separated, yet it still provides large amounts of lift, this phenomenon is called stall delay. This effect was observed in flapping insect flight and it was proven to be capable of providing enough lift to account for the deficiency in the quasi-steady-state models. This effect is used by canoeists in a sculling draw stroke.[4][5]
All of the effects on a flapping wing may be reduced to three major sources of aerodynamic phenomena: the leading edge vortex, the steady-state aerodynamic forces on the wing, and the wing’s contact with its wake from previous strokes. The size of flying insects ranges from about 20 micrograms to about 3 grams. As insect body mass increases, wing area increases and wing beat frequency decreases. For larger insects, the Reynolds number (Re) may be as high as 10000. For smaller insects, it may be as low as 10. This means that viscous effects are much more important to the smaller insects, although the flow is still laminar, even in the largest fliers.[5][6]


Another interesting feature of insect flight is the body tilt. As flight speed increases, the insect body tends to tilt nose-down and become more horizontal. This reduces the frontal area and therefore, the body drag. Since drag also increases as forward velocity increases, the insect is making its flight more efficient as this efficiency becomes more necessary. Additionally, by changing the geometric angle of attack on the downstroke, the insect is able to keep its flight at an optimal efficiency through as many manoeuvres as possible. The development of general thrust is relatively small compared with lift forces. Lift forces may be more than three times the insect's weight, while thrust at even the highest speeds may be as low as 20% of the weight. This force is developed primarily through the less powerful upstroke of the flapping motion.[5][7]

Clap and fling

The second method of flight, clap and fling, or the Weis-Fogh mechanism, functions differently. In this process, found in very small insects such as thrips and chalcid wasps, the wings clap together above the insect's body and then fling apart. As they fling open, the air gets sucked in and creates a vortex over each wing. This bound vortex then moves across the wing and, in the clap, acts as the starting vortex for the other wing. By this effect, circulation and thus, lift are increased to the extent of being higher, in most cases, than the typical leading edge vortex effect. One of the reasons this method is not employed by more insects is the inevitable damage and wear to the wings caused by repeated clapping. It is prevalent, however, among insects that are very small and experience low Reynolds numbers.[4][5] The mechanism is also employed by the marine mollusc Limacina helicina, a sea butterfly.[8][9]
- Clap and fling flight mechanism after Sane 2003
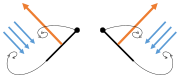 Clap 1: wings close over back
Clap 1: wings close over back Clap 2: leading edges touch, wing rotates around leading edge, vortices form
Clap 2: leading edges touch, wing rotates around leading edge, vortices form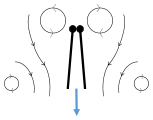 Clap 3: trailing edges close, vortices shed, wings close giving thrust
Clap 3: trailing edges close, vortices shed, wings close giving thrust
- Black (curved) arrows: flow; Blue arrows: induced velocity; Orange arrows: net force on wing
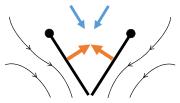 Fling 1: wings rotate around trailing edge to fling apart
Fling 1: wings rotate around trailing edge to fling apart Fling 2: leading edge moves away, air rushes in, increasing lift
Fling 2: leading edge moves away, air rushes in, increasing lift Fling 3: new vortex forms at leading edge, trailing edge vortices cancel each other, perhaps helping flow to grow faster (Weis-Fogh 1973)
Fling 3: new vortex forms at leading edge, trailing edge vortices cancel each other, perhaps helping flow to grow faster (Weis-Fogh 1973)
Governing equations
A wing moving in fluids experiences a fluid force, which follows the conventions found in aerodynamics. The force component normal to the direction of the far field flow relative to the wing is referred to as lift (L), and the force component in the opposite direction of the flow is drag (D). At the Reynolds numbers considered here, an appropriate force unit is 1/2(ρU2S), where ρ is the density of the fluid, S the wing area, and U the wing speed. The dimensionless forces are called lift (CL) and drag (CD) coefficients, that is:[4]
CL and CD are constants only if the flow is steady. A special class of objects such as airfoils may reach a steady state when it slices through the fluid at a small angle of attack. In this case, the inviscid flow around an airfoil can be approximated by a potential flow satisfying the no-penetration boundary condition. The Kutta-Joukowski theorem of a 2D airfoil further assumes that the flow leaves the sharp trailing edge smoothly, and this determines the total circulation around an airfoil. The corresponding lift is given by Bernoulli's principle (Blasius theorem):[4]
The flows around birds and insects can be considered incompressible: The Mach number, or speed while moving through air, is typically 1/300 and the wing frequency is about 10–103 Hz. Using the governing equation as the Navier-Stokes equation being subject to the no-slip boundary condition, the equation is:[4]
Where u(x, t) is the flow field, p the pressure, ρ the density of the fluid, ν the kinematic viscosity, ubd the velocity at the boundary, and us the velocity of the solid. By choosing a length scale, L, and velocity scale, U, the equation can be expressed in nondimensional form containing the Reynolds number, Re=UL/ν . There are two obvious differences between an insect wing and an airfoil: An insect wing is much smaller and it flaps. Using a dragonfly as an example, Its chord (c) is about 1 cm, its wing length (l) about 4 cm, and its wing frequency (f) about 40 Hz. The tip speed (u) is about 1 m/s, and the corresponding Reynolds number, Re=uc/ν about 103. At the smaller end, a Chalcid wasp has a wing length of about 0.5–0.7 mm and beats its wing at about 400 Hz. Its Reynolds number is about 25. The range of Reynolds number in insect flight is about 10 to 104, which lies in between the two limits that are convenient for theories: inviscid steady flows around an airfoil and Stokes flow experienced by a swimming bacterium. For this reason, this intermediate range is not well understood. On the other hand, it is perhaps the most ubiquitous regime among the things we see. Falling leaves and seeds, fishes, and birds all encounter unsteady flows similar to that seen around an insect.[4]
In addition to the Reynolds number, there are at least two other relevant dimensionless parameters. A wing has three velocity scales: the flapping velocity with respect to the body (u), the forward velocity of the body (U0), and the pitching velocity (Ωc). The ratios of them form two dimensionless variables, U0/u and Ωc/u, the former is often referred to as the advance ratio, and it is also related to the reduced frequency, fc/U0.[4]
If an insect wing is rigid, for example, a Drosophila wing is approximately so, its motion relative to a fixed body can be described by three variables: the position of the tip in spherical coordinates, (Θ(t),Φ(t)), and the pitching angle ψ(t), about the axis connecting the root and the tip. To estimate the aerodynamic forces based on blade-element analysis, it is also necessary to determine the angle of attack (α). The typical angle of attack at 70% wingspan ranges from 25° to 45° in hovering insects (15° in hummingbirds). Despite the wealth of data available for many insects, relatively few experiments report the time variation of α during a stroke. They include wind tunnel experiments of a tethered locust and a tethered fly, and free hovering flight of a fruit fly.[4]
Because they are relatively easy to measure, the wing-tip trajectories have been reported more frequently. For example, selecting only flight sequences that produced enough lift to support a weight, will show that the wing tip follows an elliptical shape. Noncrossing shapes were also reported for other insects. Regardless of their exact shapes, the plugging-down motion indicates that insects may use aerodynamic drag in addition to lift to support its weight.[4]
Hovering
| Wing beats[10] | |
|---|---|
| Honeybee | 250 beats/s |
| Housefly | 190 beats/s |
| Bumblebee | 130 beats/s |
| Syrphid hoverfly | 120 beats/s |
| Hornet | 100 beats/s |
| Horsefly | 96 beats/s |
| Hummingbird hawkmoth | 85 beats/s |
| Aeschnid dragonfly | 38 beats/s |
| Scorpion fly | 28 beats/s |
| Damselfly | 16 beats/s |
| Large white butterfly | 12 beats/s |
| Wing speed[10] | |
|---|---|
| Aeschnid dragonfly | 15.6 mph (25.1 km/h) |
| Hornet | 12.8 mph (20.6 km/h) |
| Hummingbird moth | 11.1 mph (17.9 km/h) |
| Horsefly | 8.8 mph (14.2 km/h) |
| Syrphid hoverfly | 7.8 mph (12.6 km/h) |
| Bumblebee | 6.4 mph (10.3 km/h) |
| Honeybee | 5.7 mph (9.2 km/h) |
| Housefly | 4.4 mph (7.1 km/h) |
| Damselfly | 3.3 mph (5.3 km/h) |
| Scorpion fly | 1.1 mph (1.8 km/h) |
Many insects can hover, or stay in one spot in the air, doing so by beating their wings rapidly. The ability to do so, though, is complex; requiring the use of sideways stabilization as well as the lift necessary to overcome the force of gravity. The lifting force is caused by the downward stroke of the wings. As the wings push down on the surrounding air, the result reaction force of the air on the wings force the insect up. The wings of most insects are evolved so that, during the upward stroke, the force on the wing is small. Due to the fact that the upbeat and downbeat force the insect down and up respectively, the insect oscillates and winds up staying in the same position.[6]
The distance the insect falls between wingbeats depends on how rapidly its wings are beating. If the insect flaps its wings at a slow rate, the time interval during which the lifting force is zero is longer, and therefore the insect falls farther than if its wings were beating rapidly. One can calculate the wingbeat frequency necessary for the insect to maintain a given stability in its amplitude. To simplify the calculations, one must assume that the lifting force is at a finite constant value while the wings are moving down and that it is zero while the wings are moving up. During the time interval Δt of the upward wingbeat, the insect drops a distance h under the influence of gravity.[6]
The upward stroke then restores the insect to its original position. Typically, it may be required that the vertical position of the insect change by no more than 0.1 mm (i.e., h=0.1 mm). The maximum allowable time for free fall is then [6]
Since the up movements and the down movements of the wings are about equal in duration, the period T for a complete up-and-down wing is twice Δr, that is,[6]
The frequency of the beats, f, meaning the number of wingbeats per second, is represented by the equation:[6]
In the examples used the frequency used is 110 beats/s, which is the typical frequency found in insects. Butterflies have a much slower frequency with about 10 beats/s, which means that they can't hover. Other insects may be able to produce a frequency of 1000 beats/s. To restore the insect to its original vertical position, the average upward force during the downward stroke, Fav, must be equal to twice the weight of the insect. Note that since the upward force on the insect body is applied only for half the time, the average upward force on the insect is simply its weight.[6]
Power input
One can now compute the power required to maintain hovering by, considering again an insect with mass m 0.1 g, average force, Fav, applied by the two wings during the downward stroke is two times the weight. Because the pressure applied by the wings is uniformly distributed over the total wing area, that means one can assume the force generated by each wing acts through a single point at the midsection of the wings. During the downward stroke, the center of the wings traverses a vertical distance d.[6] The total work done by the insect during each downward stroke is the product of force and distance; that is,
If the wings swing through the beat at an angle of 70°, then in the case presented for the insect with 1 cm long wings, d is 0.57 cm. Therefore, the work done during each stroke by the two wings is:[6]
After, the energy has to go somewhere; here, in the example used, the mass of the insect has to be raised 0.1 mm during each downstroke. The energy E required for this task is:[6]
This is a negligible fraction of the total energy expended which clearly, most of the energy is expended in other processes. A more detailed analysis of the problem shows that the work done by the wings is converted primarily into kinetic energy of the air that is accelerated by the downward stroke of the wings. The power is the amount of work done in 1 s; in the insect used as an example, makes 110 downward strokes per second. Therefore, its power output P is, strokes per second, and that means its power output P is:[6]
Power output
In the calculation of the power used in hovering, the examples used neglected the kinetic energy of the moving wings. The wings of insects, light as they are, have a finite mass; therefore, as they move they possess kinetic energy. Because the wings are in rotary motion, the maximum kinetic energy during each wing stroke is:[6]
Here I is the moment of inertia of the wing and ωmax is the maximum angular velocity during the wing stroke. To obtain the moment of inertia for the wing, we will assume that the wing can be approximated by a thin rod pivoted at one end. The moment of inertia for the wing is then:[6]
Where l is the length of the wing (1 cm) and m is the mass of two wings, which may be typically 10−3 g. The maximum angular velocity, ωmax, can be calculated from the maximum linear velocity, νmax, at the center of the wing:[6]
During each stroke the center of the wings moves with an average linear velocity νav given by the distance d traversed by the center of the wing divided by the duration Δt of the wing stroke. From our previous example, d=0.57 cm and Δt=4.5×10−3 s. Therefore:[6]
The velocity of the wings is zero both at the beginning and at the end of the wing stroke, meaning the maximum linear velocity is higher than the average velocity. If we assume that the velocity varies sinusoidally along the wing path, the maximum velocity is twice as high as the average velocity. Therefore, the maximum angular velocity is:[6]
And the kinetic energy therefore is:[6]
Since there are two wing strokes (the upstroke and downstroke) in each cycle of the wing movement, the kinetic energy is 2×43=86 erg. This is about as much energy as is consumed in hovering itself.[6]
Elasticity

Insects gain kinetic energy, provided by the muscles, when they accelerate. When the wings begin to decelerate toward the end of the stroke, this energy must dissipate. During the downstroke, the kinetic energy is dissipated by the muscles themselves and is converted into heat (this heat is sometimes used to maintain core body temperature). Some insects are able to utilize the kinetic energy in the upward movement of the wings to aid in their flight. The wing joints of these insects contain a pad of elastic, rubber-like protein called resilin. During the upstroke of the wing, the resilin is stretched. The kinetic energy of the wing is converted into potential energy in the stretched resilin, which stores the energy much like a spring. When the wing moves down, this energy is released and aids in the downstroke.[6]
Using a few simplifying assumptions, we can calculate the amount of energy stored in the stretched resilin. Although the resilin is bent into a complex shape, the example given shows the calculation as a straight rod of area A and length. Furthermore, we will assume that throughout the stretch the resilin obeys Hooke's law. This is not strictly true as the resilin is stretched by a considerable amount and therefore both the area and Young’s modulus change in the process of stretching. The energy E stored in the stretched resilin is:[6]
Here Y is the Young’s modulus for resilin, which has been measured to be 1.8×107 dyn/cm2. Typically, in an insect the size of a bee the volume of the resilin may be equivalent to a cylinder 2×10−2 cm long and 4×10−4 cm2 in area. In the example given, the length of the resilin rod is increased by 50% when stretched. That is, Δℓ is 10−2 cm. Therefore, in this case the energy stored in the resilin of each wing is:[6]
The stored energy in the two wings is 36 erg, which is comparable to the kinetic energy in the upstroke of the wings. Experiments show that as much as 80% of the kinetic energy of the wing may be stored in the resilin.[6]
Wing coupling

Some four-winged insect orders, such as the Lepidoptera, have developed a wide variety of morphological wing coupling mechanisms in the imago which render these taxa as "functionally dipterous".[11] All, but the most basal forms, exhibit this wing-coupling.[12]:4266
The mechanisms are of three different types - jugal, frenulo-retinacular and amplexiform.[13]
The more primitive groups have an enlarged lobe-like area near the basal posterior margin, i.e. at the base of the forewing, called jugum, that folds under the hindwing in flight.[12][14]
Other groups have a frenulum on the hindwing that hooks under a retinaculum on the forewing.[14]
In the butterflies (except the male of one species of hesperiid) and in the Bombycoidea (except the Sphingidae), there is no arrangement of frenulum and retinaculum to couple the wings. Instead, an enlarged humeral area of the hindwing is broadly overlapped by the forewing. Despite the absence of a specific mechanical connection, the wings overlap and operate in phase. The power stroke of the forewing pushes down the hindwing in unison. This type of coupling is a variation of frenate type but where the frenulum and retinaculum are completely lost.[11][15]
Biochemistry
The biochemistry of insect flight has been a focus of considerable study. While many insects use carbohydrates and lipids as the energy source for flight, many beetles and flies use the amino acid proline as their energy source.[16] Some species also use a combination of sources and moths such as Manduca sexta use carbohydrates for pre-flight warm-up.[17]
Insects that beat their wings less than one hundred times a second use synchronous muscle. A Synchronous muscle is a type of muscle that contracts once for every nerve impulse, which is more efficient for fast flight.[18] Insects that beat their wings more rapidly, such as the bumblebee, use asynchronous muscle; this is a type of muscle that contracts more than once per nerve impulse. This is achieved by the muscle being stimulated to contract again by a release in tension in the muscle, which can happen more rapidly than through simple nerve stimulation alone.[19]
Evolution and adaptation

Sometime in the Carboniferous Period, some 350 million years ago, when there were only two major land masses, insects began flying. How and why insect wings developed is not well understood, largely due to the scarcity of appropriate fossils from the period of their development in the Lower Carboniferous. Three main theories on the origins of insect flight are that wings developed from paranotal lobes, extensions of the thoracic terga; that they are modifications of movable abdominal gills as found on aquatic naiads of mayflies; or that they developed from thoracic protrusions used as radiators.[20]
Paranotal hypothesis
The paranotal hypothesis suggests that the insect's wings developed from paranotal lobes, a preadaptation found in insect fossils that is believed to have assisted stabilization while hopping or falling. In favor of this hypothesis is the tendency of most insects, when startled while climbing on branches, to escape by dropping to the ground. Such lobes would have served as parachutes and enable the insect to land more softly. The theory suggests that these lobes gradually grew larger and in a later stage developed a joint with the thorax. Even later would appear the muscles to move these crude wings. This model implies a progressive increase in the effectiveness of the wings, starting with parachuting, then gliding and finally active flight. Still, lack of substantial fossil evidence of the development of the wing joints and muscles poses a major difficulty to the theory, as does the seemingly spontaneous development of articulation and venation, and it has been largely rejected by experts in the field.[20]
Epicoxal hypothesis
Some entomologists have suggested that a possible origin for insect wings might have been movable abdominal gills found in many aquatic insects, such as on naiads of mayflies. According to this theory these tracheal gills, which started their way as exits of the respiratory system and over time were modified into locomotive purposes, eventually developed into wings. The tracheal gills are equipped with little winglets that perpetually vibrate and have their own tiny straight muscles.[20]
Endite-exite hypothesis
The hypothesis with perhaps the strongest evidence is that which stems from the adaptation of endites and exites, appendages on the respective inner and outer aspects of the primitive arthropod limb. This was advanced by Trueman based on a study by Goldschmidt in 1945 on Drosophila melanogaster, in which a pod variation displayed a mutation transforming normal wings to what was interpreted as a triple-jointed leg arrangement with some additional appendages but lacking the tarsus, where the wing's costal surface normally would be. This mutation was reinterpreted as strong evidence for a dorsal exite and endite fusion, rather than a leg, with the appendages fitting in much better with this hypothesis. The innervation, articulation and musculature required for the evolution of wings are already present in podomeres.[21]
Other hypotheses
Suggestions have been made that wings may have evolved initially for sailing on the surface of water as seen in some stoneflies.[22] An alternative idea is that it drives from directed aerial gliding descent—a preflight phenomena found in some apterygote, a wingless sister taxa to the winged insects.[23]
See also
Notes
- 1 2 3 4 Chapman, A. D. (2006). Numbers of living species in Australia and the World. Canberra: Australian Biological Resources Study. pp. 60pp. ISBN 978-0-642-56850-2.
- ↑ March 2013 - Bristol Naturalists' Society Photography has revealed that both sexes unlock the pair of wings on each side and move them independently enabling hovering flight, very reminiscent of the way dragonflies fly.
- ↑ Smith D.S. (1965). "Flight muscles of insects". Scientific American. 212 (6): 76–88. doi:10.1038/scientificamerican0665-76.
- 1 2 3 4 5 6 7 8 9 10 11 Wang, Z. Jane (2005). "DISSECTING INSECT FLIGHT" (pdf). Annual Review of Fluid Mechanics. Annual Reviews. 37: 183–210. Bibcode:2005AnRFM..37..183W. doi:10.1146/annurev.fluid.36.050802.121940.
- 1 2 3 4 5 6 7 Sane, Sanjay P. (2003). "The aerodynamics of insect flight" (PDF). The Journal of Experimental Biology. 206 (23): 4191–4208. PMID 14581590. doi:10.1242/jeb.00663.
- 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 Davidovits, Paul (2008). Physics in Biology and Medicine. Academic Press. pp. 78–79. ISBN 978-0-12-369411-9.
- ↑ "Catching the Wake". Scientific American. June 28, 1999. Retrieved March 31, 2011.
- ↑ Weisberger, Mindy (19 February 2016). "Swim Like a Butterfly? Sea Snail 'Flies' Through Water". Scientific American. Retrieved 20 February 2016.
- ↑ Murphy, D.; Adhikari, D.; Webster, D.; Yen, J. (2016). "Underwater flight by the planktonic sea butterfly". Journal of Experimental Biology. 219: 535–543.
- 1 2 "Insect Wings in General". Aerodynamics of Insects. Cislunar Aerospace. 1997. Retrieved March 28, 2011.
- 1 2 Dudley, Robert. (2002).
- 1 2 Stocks, Ian.(2008). Sec.Wing Coupling in Capinera (Ed) (2008) "Encyc. Entom.", Vol 4.
- ↑ Scoble (1995) Subsec. "Wing coupling". Pp 56-60.
- 1 2 Powell, Jerry A. Ch. Lepidoptera (pp. 631-664) in Resh, & Cardé (Eds). "Encyclopedia of Insects". 2003.
- ↑ Gorb, S. (2001) Ch 4.1.5 "Inter-locking of body parts". Pp 46-50.
- ↑ I.P. Woiwod, D.R. Reynolds and C.D. Thomas(Eds) 2001. Insect Movement: Mechanisms and Consequences. CAB International.
- ↑ Joos, B. (1987). "Carbohydrate use in the flight muscles of Manduca sexta during pre-flight warm-up". Journal of Experimental Biology. 133: 317–327.
- ↑ "Definition of Synchronous muscle in the Entomologists' glossary". Department of Entomology, North Carolina State University. Retrieved 2011-03-21.
- ↑ "Definition of Asynchronous muscle in the Entomologists' glossary". Department of Entomology, North Carolina State University. Retrieved 2011-03-21.
- 1 2 3 Grimaldi, David; Engel, Michael S. (2005). Evolution of the Insects. New York: Cambridge University Press.
- ↑ Trueman JWH (1990), Comment: evolution of insect wings: a limb exite plus endite model Canadian Journal of Zoology
- ↑ Thomas, Adrian L. R.; Norberg, R. Åke (1996). "Skimming the surface — the origin of flight in insects?". Trends in Ecology & Evolution. 11 (5): 187–188. doi:10.1016/0169-5347(96)30022-0.
- ↑ Yanoviak, SP; Kaspari, M; Dudley, R (2009). "Gliding hexapods and the origins of insect aerial behaviour". Biol Lett. 5 (4): 510–2. PMC 2781901
 . PMID 19324632. doi:10.1098/rsbl.2009.0029.
. PMID 19324632. doi:10.1098/rsbl.2009.0029.
References
- Camper, M.A., "An Insect’s Role in the Development of Micro Air Vehicles," Colorado State University, 2003.
- Chapman, R.F. (1998) The Insects : Structure and Function. 4th Edition. Cambridge University Press.
- Dickinson, MH; Lehmann, FO; Sane, SP (1999). "Wing rotation and the aerodynamic basis of insect flight". Science. 284 (5422): 1954–60. PMID 10373107. doi:10.1126/science.284.5422.1954.
- Ellington, Charles P.; Van Den Berg, Coen; Willmott, Alexander P.; Thomas, Adrian L. R. (1996). "Leading-edge vortices in insect flight". Nature. 384 (6610): 626–630. Bibcode:1996Natur.384..626E. doi:10.1038/384626a0.
- Ellington, C. P. (1984). "The Aerodynamics of Hovering Insect Flight. I. The Quasi-Steady Analysis". Philosophical Transactions of the Royal Society B: Biological Sciences. 305 (1122): 1–15. Bibcode:1984RSPTB.305....1E. doi:10.1098/rstb.1984.0049.
- Ellington, CP (1999). "The novel aerodynamics of insect flight: Applications to micro-air vehicles" (PDF). The Journal of Experimental Biology. 202 (Pt 23): 3439–48. PMID 10562527.
- Grasmeyer, J.M. and Keennon, M.T., "Development of the Black Widow Micro Air Vehicle," AIAA Paper No. 2001-0127, 2001.
- Haj-Hariri, H., "Unsteady Aerodynamics of Flapping Wings," University of Virginia, 2001.
- Lewin, G. C.; Haj-Hariri, H. (2003). "Modelling thrust generation of a two-dimensional heaving airfoil in a viscous flow". Journal of Fluid Mechanics. 492: 339–362. Bibcode:2003JFM...492..339L. doi:10.1017/S0022112003005743.
- Lighthill, M. J. (2006). "On the Weis-Fogh mechanism of lift generation". Journal of Fluid Mechanics. 60: 1. Bibcode:1973JFM....60....1L. doi:10.1017/S0022112073000017.
- Platzer, Max F., Department Chairman, "Aerodynamics and Aeroelasticity: Flapping-Wing Propulsion." Last revised 2005, Retrieved 1 November 2005, from http://www.aa.nps.navy.mil/programs/aero/propulsion/
- Polhamus, E.C., "A Concept of the Vortex Lift of Sharp-Edge Delta Wings Based on a Leading-Edge-Suction Analogy," Langley Research Center, 1966.
- Pringle, J.W.S., "Insect flight," Oxford Biology Readers, Vol. 52, 1975.
- Sane, SP (2003). "The aerodynamics of insect flight". The Journal of Experimental Biology. 206 (Pt 23): 4191–208. PMID 14581590. doi:10.1242/jeb.00663.
- Savage, S.B.; Newman, B.G.; Wong, D.T.M. (1979). "The role of vortices and unsteady effects during the hovering flight of dragon flies" (PDF). The Journal of Experimental Biology. 83 (1): 59–77.
- Smyth, T., Jr. 1985. Muscle systems, pp. 227–286. In M.S. Blum [ed.], Fundamentals of insect physiology. John Wiley and Sons, New York.
- Sotavalta, O (1953). "Recordings of high wing-stroke and thoracic vibration frequency in some midges". Biol. Bull. Woods Hole. 104: 439–444. doi:10.2307/1538496.
- Van Den Berg, C.; Ellington, C. P. (1997). "The vortex wake of a 'hovering' model hawkmoth". Philosophical Transactions of the Royal Society B: Biological Sciences. 352 (1351): 317–328. PMC 1691928
 . doi:10.1098/rstb.1997.0023.
. doi:10.1098/rstb.1997.0023. - Walker, J.A. (September 2002). "Rotational lift: something difference or more of the same?" (PDF). The Journal of Experimental Biology. 205 (Pt 24): 3783–3792. PMID 12432002.
- Walker, P.B., "Growth of circulation about a wing and an apparatus for measuring fluid motion," Reports and Mem., Aeronaut. Res. Com. no. 1402, 1931.
- Zbikowski, R. (2002). "On aerodynamic modelling of an insect-like flapping wing in hover for micro air vehicles". Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences. 360 (1791): 273–290. Bibcode:2002RSPTA.360..273Z. doi:10.1098/rsta.2001.0930.
External links
| Wikimedia Commons has media related to Insect flight. |
- An Insect’s Role In The Development Of Micro Air Vehicles
- Insect-like Flapping-Wing Micro Air Vehicles
- The Novel Aerodynamics Of Insect Flight: Applications To Micro-Air Vehicles
- The aerodynamics of insect flight
- Flight muscles
- Flow visualization of butterfly aerodynamic mechanisms
- The Flight Of The Bumblebee
- Insect Flight
- T. J. Dean (2003-05-01). "Chapter 1 — Fastest Flyer". Book of Insect Records. University of Florida.