Catalan solid
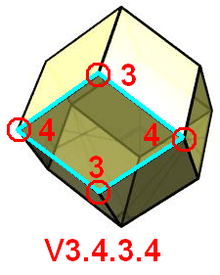

In mathematics, a Catalan solid, or Archimedean dual, is a dual polyhedron to an Archimedean solid. The Catalan solids are named for the Belgian mathematician, Eugène Catalan, who first described them in 1865.
The Catalan solids are all convex. They are face-transitive but not vertex-transitive. This is because the dual Archimedean solids are vertex-transitive and not face-transitive. Note that unlike Platonic solids and Archimedean solids, the faces of Catalan solids are not regular polygons. However, the vertex figures of Catalan solids are regular, and they have constant dihedral angles. Being face-transitive, Catalan solids are isohedra.
Additionally, two of the Catalan solids are edge-transitive: the rhombic dodecahedron and the rhombic triacontahedron. These are the duals of the two quasi-regular Archimedean solids.
Just as prisms and antiprisms are generally not considered Archimedean solids, so bipyramids and trapezohedra are generally not considered Catalan solids, despite being face-transitive.
Two of the Catalan solids are chiral: the pentagonal icositetrahedron and the pentagonal hexecontahedron, dual to the chiral snub cube and snub dodecahedron. These each come in two enantiomorphs. Not counting the enantiomorphs, bipyramids, and trapezohedra, there are a total of 13 Catalan solids.
Symmetry
The Catalan solids, along with their dual Archimedean solids, can be grouped by their symmetry: tetrahedral, octahedral, and icosahedral. There are 6 forms per symmetry, while the self-symmetric tetrahedral group only has three unique forms and two of those are duplicated with octahedral symmetry.
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Archimedean | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
 |
 |
 |
 |
 |
 | |||||
| Catalans | ||||||||||
 |
 |
 |
 |
 |
 | |||||
List
See also
- List of uniform tilings Shows dual uniform polygonal tilings similar to the Catalan solids
- Conway polyhedron notation A notational construction process
- Archimedean solid
- Johnson solid
References
- Eugène Catalan Mémoire sur la Théorie des Polyèdres. J. l'École Polytechnique (Paris) 41, 1-71, 1865.
- Alan Holden Shapes, Space, and Symmetry. New York: Dover, 1991.
- Wenninger, Magnus (1983), Dual Models, Cambridge University Press, ISBN 978-0-521-54325-5, MR 730208 (The thirteen semiregular convex polyhedra and their duals)
- Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 0-486-23729-X. (Section 3-9)
- Anthony Pugh (1976). Polyhedra: A visual approach. California: University of California Press Berkeley. ISBN 0-520-03056-7. Chapter 4: Duals of the Archimedean polyhedra, prisma and antiprisms
External links
| Wikimedia Commons has media related to Catalan solids. |
- Weisstein, Eric Wolfgang. "Catalan Solids". MathWorld.
- Weisstein, Eric Wolfgang. "Isohedron". MathWorld.
- Olshevsky, George. "Catalan". Glossary for Hyperspace. Archived from the original on 4 February 2007.
- Archimedean duals – at Virtual Reality Polyhedra
- Interactive Catalan Solid in Java