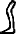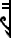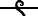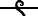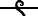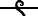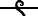Ancient Egyptian units of measurement

The ancient Egyptian units of measurement are those used by the dynasties of ancient Egypt prior to its incorporation in the Roman Empire and general adoption of Roman, Greek, and Byzantine units of measurement. The units of length seem to have originally been anthropic, based on various parts of the human body, although these were standardized using cubit rods, strands of rope, and official measures maintained at some temples.

Following Alexander the Great's conquest of Persia and subsequent death, his bodyguard and successor Ptolemy assumed control in Egypt, partially reforming its measurements, introducing some new units and hellenized names for others.

Length

Egyptian Circle Egyptian units of length are attested from the Early Dynastic Period, when the Palermo stone recorded the level of the Nile River. During the reign of Pharaoh Djer, the height of the Nile was recorded as 6 cubits and 1 palm (about 3.217 m or 10 ft 6.7 in).^[1] A 3rd-dynasty diagram shows how to construct an elliptical vault using simple measures along an arc. The ostracon depicting this diagram was found near the Step Pyramid of Saqqara. A curve is divided into five sections and the height of the curve is given in cubits, palms, and digits in each of the sections.^{[2] [3]}

At some point, lengths were standardized by cubit rods. Examples have been found in the tombs of officials, noting lengths up to remen. Royal cubits were used for land measures such as roads and fields. Fourteen rods, including one double-cubit rod, were described and compared by Lepsius.^[4] Two examples are known from the Saqqara tomb of Maya, the treasurer of Tutankhamun. Another was found in the tomb of Kha (TT8) in Thebes. These cubits are about 52.5 cm (20.7 in) long and are divided into palms and hands: each palm is divided into four fingers from left to right and the fingers are further subdivided into ro from right to left. The rules are also divided into hands^[5] so that for example one foot is given as three hands and fifteen fingers and also as four palms and sixteen fingers.^{[1][3][6][7][8][5]}

Cubit rod from the Turin Museum.

Surveying and itinerant measurement were undertaken using rods, poles, and knotted cords of rope. A scene in the tomb of Menna in Thebes shows surveyors measuring a plot of land using rope with knots tied at regular intervals. Similar scenes can be found in the tombs of Amenhotep-Sesi, Khaemhat and Djeserkareseneb. The balls of rope are also shown in New Kingdom statues of officials such as Senenmut, Amenemhet-Surer, and Penanhor.^[2]

Units of Length^[1][2]

Names					Equivalents
English	Egyptian		Coptic		Palms	Digits	Metric[9]
Digit[10] Finger[11] Fingerbreadth[10] Tebā[12]	[lower-alpha 1]	ḏbꜥ	ⲧⲏⲏⲃⲉ[14][15]	tēēbe	¼	1	1.875 cm
Palm[10] Hand[16] Shesep[17]	[lower-alpha 2]	šsp	ϣⲟⲡ[18][15] ϣⲟⲟⲡ[18] ϣⲱⲡ[18] ϣⲁⲡ[18]	shop shoop shōp shap	1	4	7.5 cm
Hand[19] Handsbreadth[17]	[lower-alpha 3]	ḏrt	ϩⲱϩϥ[20][21]	hōhf	1¼	5	9.38 cm
Fist[17]	[lower-alpha 4]	ḫfꜥ[17] ꜣmm[13]	ϭⲁϫⲙⲏ[22] ϫⲁⲙⲏ[22]	qajmē jamē	1½	6	11.25 cm
Double Handbreadth[13]	[lower-alpha 5]	šspwy			2	8	15 cm[13]
Small Span[17] Pedj-Sheser Shat Nedjes[17] Little Shat[11]		pḏ šsr šꜣt nḏs[17]	ⲣⲧⲱ[23][21] ⲉⲣⲧⲱ[24]	rtō ertō	3	12	22.5 cm
Great Span[17] Half-Cubit[13] Pedj-Aa Shat Aa[17] Great Shat[11]	[lower-alpha 6]	pḏ ꜥꜣ[17][13] šꜣt ꜥꜣ[17]			3½	14	26 cm
Foot Djeser[17] Ser[12] Bent Arm[17]		ḏsr			4	16	30 cm
Shoulder Remen[17] Upper Arm[17]		rmn			5	20	37.5 cm
Small Cubit[19] Short Cubit[17] Meh Nedjes[17]		mḥ nḏs mḥ šsr	ⲙⲁϩⲉ[25][15] ⲙⲉϩⲓ[26]	mahe mehi	6	24	45 cm
Cubit Royal Cubit[17] Sacred Cubit[16] Meh Nesut[10] Meh Nisut[17] Mahi Ell[25]	[lower-alpha 7]	mḥ			7	28	52.3 cm[10] 52.5 cm[16]
Pole Nebiu[27]		nbiw			8	32	60 cm
Rod Rod of Cord Stick of Rope[19] Khet[10] Schoinion[28]	[lower-alpha 8]	ḫt	ϩⲱⲧⲉ[30] ϩⲱϯ[30]	hōte hōti	100 cubits[10]		52.5 m[28]
Schoenus[13] River-Measure League[13] Ater[10] Iter[19] or Iteru[13]	[lower-alpha 9]	i͗trw	ϣϥⲱ[31] ϣⲃⲱ[31]	shfō shvō	20,000 cubits[10]		10.5 km[10]

The digit was also subdivided into smaller fractions of ½, ⅓, ¼, and  ¹⁄₁₆.^[32] Minor units include the Middle Kingdom reed of 2 royal cubits,^{[lower-alpha 10]} the Ptolemaic xylon (Greek: ξύλον, lit. "timber") of three royal cubits,^[33][34] the Ptolemaic fathom (Greek: ὀργυιά, orgyiá; Egyptian: ḥpt; Coptic: ϩⲡⲟⲧ, hpot) of four lesser cubits,^[35] and the kalamos of six royal cubits.^[16]

Area

Records of land area also date to the Early Dynastic Period. The Palermo stone records grants of land expressed in terms of kha and setat. Mathematical papyri also include units of land area in their problems. For example, several problems in the Moscow Mathematical Papyrus give the area of rectangular plots of land in terms of setat and the ratio of the sides and then require the scribe to solve for their exact lengths.^[1]

The setat was the basic unit of land measure and may originally have varied in size across Egypt's nomes.^[19] Later, it was equal to one square khet, where a khet measured 100 cubits. The setat could be divided into strips one khet long and ten cubit wide (a kha).^[2][1][36]

During the Old Kingdom:

Units of Area

Names					Equivalents[37]
English	Egyptian		Coptic		Setat	Square Cubits	Metric
Sa[19] Eighth		zꜣ			1⁄800	12½	3.4456 m²
Heseb Fourth Account Unit[19]		ḥsb			1⁄400	25	6.8913 m²
Remen Half Shoulder[19]		rmn			1⁄200	50	13.783 m²
Ta Khet[37] Cubit[38] Cubit of Land[38] Land Cubit[13] Ground Cubit[38] Cubit Strip[38] Land Unit[19]	[lower-alpha 11]	tꜣ ḫt mḥ mḥ itn	ϫⲓⲥⲉ[39][21]	jise	1⁄100	100[37]	27.565 m²
Kha Thousand[19]		ḫꜣ			1⁄10	1000	275.65 m²
Setat[37] Setjat[37] Aroura[37] Square Khet[37]	[lower-alpha 12]	sṯꜣ[28] sṯꜣt[37]	ⲥⲱⲧ[40][21] ⲥⲧⲉⲓⲱϩⲉ[41][21]	sōt steiōhe	1	10,000	2756.5 m²

During the Middle and New Kingdom, the "eighth", "fourth", "half", and "thousand" units were taken to refer to the setat rather than the cubit strip:

Sa Eighth	[lower-alpha 13]	sꜣ			⅛	1250	345 m²
Heseb Fourth	[lower-alpha 14]	hsb r-fdw			¼	2500	689 m²
Gs Remen Half	[lower-alpha 15]	gs	ⲣⲉⲣⲙⲏ[21]	rermē	½	5000	1378 m²
Kha Thousand	[lower-alpha 16]	ḫꜣ ḫꜣ tꜣ			10	100,000	2.76 ha

During the Ptolemaic period, the cubit strip square was surveyed using a length of 96 cubits rather than 100, although the aroura was still figured to compose 2756.25 m².^[16] A 36 sq. cubit area was known as a kalamos and a 144 sq. cubit area as a hamma.^[16] The uncommon bikos may have been 1½ hammata or another name for the cubit strip.^[16] The Coptic shipa (ϣⲓⲡⲁ) was a land unit of uncertain value, possibly derived from Nubia.^[42]

Volume

A bronze capacity measure inscribed with the cartouches of the birth and throne names of Amenhotep III of the 18th Dynasty

Units of volume appear in the mathematical papyri. For example, computing the volume of a circular granary in RMP 42 involves cubic cubits, khar, heqats, and quadruple heqats.^[1][8] RMP 80 divides heqats of grain into smaller henu.

Problem 80 on the Rhind Mathematical Papyrus: As for vessels (debeh) used in measuring grain by the functionaries of the granary: done into henu, 1 hekat makes 10; 1/2 makes 5; 1/4 makes 2½; etc.^[1][8]

Units of Volume^[1][2]

Names			Equivalents
English	Egyptian		Heqats	Ro	Metric
Ro		r	1⁄320	1	0.015 L
Dja		dja	1⁄16	20[43]	0.30 L
Jar Hinu		hnw	1⁄10	32	0.48 L
Barrel Heqat Hekat		hqt	1	320	4.8 L
Double Barrel Double Heqat Double Hekat		hqty	2	640	9.6 L
Quadruple Heqat (MK)[44] Oipe[45] (NK)[44]		hqt-fdw jpt[19] ipt[44]	4	1280	19.2 L
Sack Khar		khar	20 (MK) 16 (NK)[46]	6400 (MK) 5120 (NK)	96.5 L (MK) 76.8 L (NK)[46]
Deny Cubic cubit		deny	30	9600	144 L

The oipe was also formerly romanized as the apet.^[47]

Weight

Green glazed faience weight discovered at Abydos, inscribed for the high steward Aabeni during the late Middle Kingdom

Serpentine weight of 10 daric, inscribed for Taharqa during the 25th Dynasty

Weights were measured in terms of deben. This unit would have been equivalent to 13.6 grams in the Old Kingdom and Middle Kingdom. During the New Kingdom however it was equivalent to 91 grams. For smaller amounts the qedet (1/10 of a deben) and the shematy (1/12 of a deben) were used.^[2][8]

Units of Weight^[2]

Names			Equivalents
English	Egyptian		Debens	Metric
Piece Shematy		shȝts	1⁄12
Qedet Kedet Kite		qdt	1⁄10
Deben		dbn	1	13.6 g (OK & MK) 91 g (NK)

The qedet or kedet is also often known as the kite, from the Coptic form of the same name (ⲕⲓⲧⲉ or ⲕⲓϯ).^[48] In 19th-century sources, the deben and qedet are often mistakenly transliterated as the uten and kat respectively, although this was corrected by the 20th century.^[49]

Time

The former annual flooding of the Nile organized prehistoric and ancient Egypt into three seasons: Akhet ("Flood"), Peret ("Growth"), and Shemu or Shomu ("Low Water" or "Harvest").^[50][51][52]

The Egyptian civil calendar in place by Dynasty V^[53] followed regnal eras resetting with the ascension of each new pharaoh.^[54] It was based on the solar year and apparently initiated during a heliacal rising of Sirius following a recognition of its rough correlation with the onset of the Nile flood.^[55] It followed none of these consistently, however. Its year was divided into 3 seasons, 12 months, 36 decans, or 360 days with another 5 epagomenal days^[56]—celebrated as the birthdays of five major gods^[57] but feared for their ill luck^[58]—added "upon the year". The Egyptian months were originally simply numbered within each season^[59] but, in later sources, they acquired names from the year's major festivals^[60] and the three decans of each one were distinguished as "first", "middle", and "last".^[61] It has been suggested that during the Nineteenth Dynasty and the Twentieth Dynasty the last two days of each decan were usually treated as a kind of weekend for the royal craftsmen, with royal artisans free from work..^[62] This scheme lacked any provision for leap year intercalation until the introduction of the Alexandrian calendar by Augustus in the 20s BC, causing it to slowly move through the Sothic cycle against the solar, Sothic, and Julian years.^[1][3][63] Dates were typically given in a YMD format.^[54]

The civil calendar was apparently preceded by an observational lunar calendar which was eventually made lunisolar^{[lower-alpha 17]}</ref> and fixed to the civil calendar, probably in 357 BC.^[65] The months of these calendars were known as "temple months"^[66] and used for liturgical purposes until the closing of Egypt's pagan temples under Theodosius I^[67] in the AD 390s and the subsequent suppression of individual worship by his successors.^[68]

Smaller units of time were vague approximations for most of Egyptian history. Hours—known by a variant of the word for "stars"^[69]—were initially only demarcated at night and varied in length. They were measured using decan stars and by water clocks. Equal 24-part divisions of the day were only introduced in 127 BC. Division of these hours into 60 equal minutes is attested in Ptolemy's 2nd-century works.

Units of Time^[1][2]

Name			Days
English	Egyptian
hour	[lower-alpha 18]	wnwt	variable
day	[lower-alpha 19]	sw	1
decan decade week	[lower-alpha 20]	"ten-day" sw mḏ[79]	10
month	[lower-alpha 21]	ꜣbd	30
season		ı͗trw[lower-alpha 22]	120
year	[lower-alpha 23]	rnpt	365    365¼

See also

• Egyptian hieroglyphics and Transliteration of Ancient Egyptian
• Ancient Egyptian mathematics and technology
• Egyptian calendar and astronomy
• Ancient Mesopotamian, Hebrew, Persian, Greek, Roman, and Byzantine units of measurement
• Modern Egyptian units of measurement & Metric system

Notes

References

Citations

Bibliography

• Bagnall, Roger Shaler (2009), "Practical Help: Chronology, Geography, Measures, Currency, Names, Prosopography, and Technical Vocabulary", The Oxford Handbook of Papyrology, Oxford: Oxford University Press, pp. 179–196 .
• Clagett, Marshall (1995), Ancient Egyptian Science: A Source Book, Vol. II: Calendars, Clocks, and Astronomy, Memoirs of the APS, No. 214, Philadelphia: American Philosophical Society .
• Clagett, Marshall (1999), Ancient Egyptian Science: A Source Book, Vol. III: Ancient Egyptian Mathematics, Memoirs of the APS, Vol. 232, Philadelphia: American Philosophical Society, ISBN 978-0-87169-232-0 .
• Crum, Walter Ewing (1939), A Coptic Dictionary, Oxford: Clarendon Press, p. 210 .
• Jauhiainen, Heidi (2009), Do Not Celebrate Your Feast without Your Neighbors: A Study of References to Feasts and Festivals in Non-Literary Documents from Ramesside Period Deir el-Medina (PDF), Publications of the Institute for Asian and African Studies, No. 10, Helsinki: University of Helsinki .
• Lepsius, Karl Richard (1865), Die Alt-Aegyptische Elle und Ihre Eintheilung, Berlin: Dümmler . (in German)
• Parker, Richard Anthony (1950), The Calendars of Ancient Egypt (PDF), Studies in Ancient Oriental Civilization, No. 26, Chicago: University of Chicago Press .
• Spalinger, Anthony (January 1995), "Some Remarks on the Epagomenal Days in Ancient Egypt", Journal of Near Eastern Studies, Vol. 54, No. 1, pp. 33–47 .
• Tetley, M. Christine (2014), The Reconstructed Chronology of the Egyptian Kings, Vol. I .
• Weigall, Arthur Edward Pearse Brome (1908), Weights and Balances (PDF), Catalogue Général des Antiquités Égyptiennes du Musée du Caire, No. 31271-31670, Cairo: Imprimerie de l'Institut Français d'Archéologie Orientale .
• Winlock, Herbert Eustis (1940), "The Origin of the Ancient Egyptian Calendar", Proceedings of the American Philosophical Society, No. 83, New York: Metropolitan Museum of Art, pp. 447–464 .

External links

• Egyptian ceremonial ruler showing fingers, palms, hands, fists, feet, remen
• Cubit divided into fingers and hands
• Modern replica of Egyptian ruler
• Measuring length in Ancient Egypt Page by Digitalegypt (University College London).
• Irrational numbers and pyramids Article by Gay Robins and C.C.D. Shute
• Introduction to Egyptian Mathematics, with photographs of Maya's cubit rod from the Louvre and land surveying scenes from the tomb of Menna.