5
| ||||
|---|---|---|---|---|
|
[[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] [[{{#expr: (floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}} (number)|{{#switch:{{{1}}}|-1={{#ifexpr:(floor({{{number}}} div 10)) = 0|-1|←}}|10=→|#default={{#expr:(floor({{{number}}} div {{{factor}}})) * {{{factor}}}+({{{1}}}*{{{factor}}} div 10)}}}}]] | ||||
| Cardinal | five | |||
| Ordinal |
5th (fifth) | |||
| Numeral system | quinary | |||
| Factorization | prime | |||
| Prime | Yes | |||
| Divisors | 1, 5 | |||
| Roman numeral | V | |||
| Roman numeral (unicode) | Ⅴ, ⅴ | |||
| Greek prefix | penta-/pent- | |||
| Latin prefix | quinque-/quinqu-/quint- | |||
| Binary | 1012 | |||
| Ternary | 123 | |||
| Quaternary | 114 | |||
| Quinary | 105 | |||
| Senary | 56 | |||
| Octal | 58 | |||
| Duodecimal | 512 | |||
| Hexadecimal | 516 | |||
| Vigesimal | 520 | |||
| Base 36 | 536 | |||
| Greek | ε (or Ε) | |||
| Arabi & Kurdish | ٥ | |||
| Persian | ۵ | |||
| Urdu | ۵ | |||
| Ge'ez | ፭ | |||
| Bengali | ৫ | |||
| Kannada | ೫ | |||
| Punjabi | ੫ | |||
| Chinese numeral | 五,伍 | |||
| Korean | 다섯,오 | |||
| Devanāgarī | ५ (panch) | |||
| Hebrew | ה (Hey) | |||
| Khmer | ៥ | |||
| Telugu | ౫ | |||
| Malayalam | ൫ | |||
| Tamil | ௫ | |||
5 (five /ˈfaɪv/) is a number, numeral, and glyph. It is the natural number following 4 and preceding 6.
In mathematics
Five is the third prime number. Because it can be written as 221 + 1, five is classified as a Fermat prime; therefore a regular polygon with 5 sides (a regular pentagon) is constructible with compass and unmarked straightedge. 5 is the third Sophie Germain prime, the first safe prime, the third Catalan number, and the third Mersenne prime exponent. Five is the first Wilson prime and the third factorial prime, also an alternating factorial. Five is the first good prime.[1] It is an Eisenstein prime with no imaginary part and real part of the form 3n − 1. It is also the only number that is part of more than one pair of twin primes. Five is a congruent number.[2]
Five is conjectured to be the only odd untouchable number and if this is the case then five will be the only odd prime number that is not the base of an aliquot tree.
Five is also the only prime that is the sum of two consecutive primes, namely 2 and 3.
The number 5 is the fifth Fibonacci number, being 2 plus 3. 5 is also a Pell number and a Markov number, appearing in solutions to the Markov Diophantine equation: (1, 2, 5), (1, 5, 13), (2, 5, 29), (5, 13, 194), (5, 29, 433), ... (![]() A030452 lists Markov numbers that appear in solutions where one of the other two terms is 5). Whereas 5 is unique in the Fibonacci sequence, in the Perrin sequence 5 is both the fifth and sixth Perrin numbers.
A030452 lists Markov numbers that appear in solutions where one of the other two terms is 5). Whereas 5 is unique in the Fibonacci sequence, in the Perrin sequence 5 is both the fifth and sixth Perrin numbers.
5 is the length of the hypotenuse of the smallest integer-sided right triangle.
In bases 10 and 20, 5 is a 1-automorphic number.
Five is the second Sierpinski number of the first kind, and can be written as S2=(22)+1
While polynomial equations of degree 4 and below can be solved with radicals, equations of degree 5 and higher cannot generally be so solved. This is the Abel–Ruffini theorem. This is related to the fact that the symmetric group Sn is a solvable group for n ≤ 4 and not solvable for n ≥ 5.
While all graphs with 4 or fewer vertices are planar, there exists a graph with 5 vertices which is not planar: K5, the complete graph with 5 vertices.
There are five Platonic solids.[3]
A polygon with five sides is a pentagon. Figurate numbers representing pentagons (including five) are called pentagonal numbers. Five is also a square pyramidal number.
Five is the only prime number to end in the digit 5 because all other numbers written with a 5 in the ones place under the decimal system are multiples of five. As a consequence of this, 5 is in base 10 a 1-automorphic number.
Vulgar fractions with 5 or 2 in the denominator do not yield infinite decimal expansions, unlike expansions with all other prime denominators, because they are prime factors of ten, the base. When written in the decimal system, all multiples of 5 will end in either 5 or 0.
There are five Exceptional Lie groups.
List of basic calculations
| Multiplication | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 5 × x | 5 | 10 | 15 | 20 | 25 | 30 | 35 | 40 | 45 | 50 | 55 | 60 | 65 | 70 | 75 | 80 | 85 | 90 | 95 | 100 |
| Division | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 5 ÷ x | 5 | 2.5 | 1.6 | 1.25 | 1 | 0.83 | 0.714285 | 0.625 | 0.5 | 0.5 | 0.45 | 0.416 | 0.384615 | 0.3571428 | 0.3 | |
| x ÷ 5 | 0.2 | 0.4 | 0.6 | 0.8 | 1 | 1.2 | 1.4 | 1.6 | 1.8 | 2 | 2.2 | 2.4 | 2.6 | 2.8 | 3 |
| Exponentiation | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 5x | 5 | 25 | 125 | 625 | 3125 | 15625 | 78125 | 390625 | 1953125 | 9765625 | 48828125 | 244140625 | 1220703125 | 6103515625 | 30517578125 | |
| x5 | 1 | 32 | 243 | 1024 | 3125 | 7776 | 16807 | 32768 | 59049 | 100000 | 161051 | 248832 | 371293 | 537824 | 759375 |
Evolution of the glyph
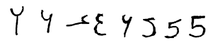
The evolution of the modern Western glyph for the numeral five cannot be traced back to the Indian system as for the numbers 1 to 4. The Kushana and Gupta empires in what is now India had among themselves several different glyphs which bear no resemblance to the modern glyph. The Nagari and Punjabi took these glyphs and all came up with glyphs that are similar to a lowercase "h" rotated 180°. The Ghubar Arabs transformed the glyph in several different ways, producing glyphs that were more similar to the numbers 4 or 3 than to the number 5.[4]
It was from those characters that Europeans finally came up with the modern 5, though from purely graphical evidence, it would be much easier to conclude that the modern symbol came from the Khmer. The Khmer glyph develops from the Kushana/Ândhra/Gupta numeral, its shape looking like the modern version with an extended swirled 'tail' [5]
While the shape of the 5 character has an ascender in most modern typefaces, in typefaces with text figures the character usually has a descender, as, for example, in ![]() .
.
Science
- The atomic number of boron.
- The number of appendages on most starfish, which exhibit pentamerism.
- The most destructive known hurricanes rate as Category 5 on the Saffir–Simpson hurricane wind scale.
- The most destructive known tornadoes rate an F-5 on the Fujita scale or EF-5 on the Enhanced Fujita scale.
Astronomy
- Messier object M5, a magnitude 7.0 globular cluster in the constellation Serpens.
- The New General Catalogue object NGC 5, a magnitude 13 spiral galaxy in the constellation Andromeda.
- The Roman numeral V stands for dwarfs (main sequence stars) in the Yerkes spectral classification scheme.
- The Roman numeral V (usually) stands for the fifth-discovered satellite of a planet or minor planet (e.g. Jupiter V).
- There are five Lagrangian points in a two-body system.
Biology
- Perception is conceived to occur through five senses.
- Almost all amphibians, reptiles, and mammals which have fingers or toes have five of them on each extremity.[6]
Computing
- 5 is the ASCII code of the Enquiry character, which is abbreviated to ENQ.
Religion and culture
Hinduism
- God shiva has five faces and His Mantra is also called Panchakshari (Five Worded) mantra.
- Goddess Saraswati, goddess of knowledge and intellectual is associated with Panchami or 5 number.
- There are five elements in this universe Dharti, Agni, Jal, Vayu evam Akash means Earth, Fire, Water, Air and Space respectively.
- Most sacred tree in Hinduism has 5 leaves in every leaf stunt.
- Most of the flowers have 5 petals in them.
Christian
- There are traditionally Five Wounds of Jesus Christ in Christianity: the Scourging at the Pillar, the Crowning with Thorns, the wounds in Christ's hands, the wounds in Christ's feet, and the Side Wound of Christ.
Discordianism
- In Discordianism, 5 is seen as a very important number. This is demonstrated in the Law of Fives, as well as in the Pentabarf, which contains five rules.
- Each page of the Principia Discordia—the primary religious document in Discordianism—is labeled with five digits.
Islamic
- The Five Pillars of Islam
- Muslims pray to Allah five times a day
- In Islam, particularly Shia Islam, the Panjetan or the Five Holy Purified Ones are the members of Muhammad's family: Muhammad, Ali, Fatimah, Hasan, and Husayn and is often symbolically represented by an image of the Khamsa.
Jewish
- The Torah contains five books—Genesis, Exodus, Leviticus, Numbers, and Deuteronomy—which are collectively called the Five Books of Moses, the Pentateuch (Greek for "five containers," referring to the scroll cases in which the books were kept), or Humash (חומש, Hebrew for "fifth").
- The book of Psalms is arranged into five books, paralleling the Five Books of Moses.
- The Khamsa, an ancient symbol shaped like a hand with four fingers and one thumb, is used as a protective amulet by Jews; that same symbol is also very popular in Arabic culture, known to protect from envy and the evil eye.
Sikh
- The five sacred Sikh symbols prescribed by Guru Gobind Singh are commonly known as panj kakars or the 'Five Ks' because they start with letter K representing kakka (ਕ) in the Punjabi language's Gurmukhi script. They are: kesh (unshorn hair), kangha (the comb), kara (the steel bracelet), kachhehra (the soldiers shorts), and kirpan (the sword) (in Gurmukhi: ਕੇਸ, ਕੰਘਾ, ਕੜਾ, ਕਛਹਰਾ, ਕਿਰਪਾਨ). Also, there are five deadly evils: kam (lust), krodh (anger), moh (attachment), lobh (greed), and ankhar (ego).
Daoism
Other religions and cultures
- According to ancient Greek philosophers such as Aristotle, the universe is made up of five classical elements: water, earth, air, fire, and ether. This concept was later adopted by Medieval alchemists and more recently by practitioners of Neo-Pagan religions such as Wicca.
- The pentagram, or five-pointed star, bears religious significance in various faiths including Baha'i, Christianity, Freemasonry, Satanism, Taoism, Thelema, and Wicca.
- In Cantonese, "five" sounds like the word "not" (character: 唔). When five appears in front of a lucky number, e.g. "58", the result is considered unlucky.
- In East Asian tradition, there are five elements: (water, fire, earth, wood, and metal). The Japanese names for the days of the week, Tuesday through Saturday, come from these elements via the identification of the elements with the five planets visible with the naked eye. Also, the traditional Japanese calendar has a five-day weekly cycle that can be still observed in printed mixed calendars combining Western, Chinese-Buddhist, and Japanese names for each weekday.
- Members of The Nation of Gods and Earths, a primarily African American religious organization, call themselves the "Five-Percenters" because they believe that only 5% of mankind is truly enlightened.
Art, entertainment, and media
Events
- November 5 is now known as Guy Fawkes Night or Guy Fawkes Day in the UK.
Fictional entities
- James the Red Engine, a fictional character numbered 5.
- Johnny 5 is the lead character in the film Short Circuit (1986)
- Number Five is a character in Lorien Legacies
- Sankara Stones, five magical rocks in Indiana Jones and the Temple of Doom that are sought by the Thuggees for evil purposes
- The Mach Five (マッハ号 Mahha-gō?), the racing car Speed Racer (Go Mifune in the Japanese version) drives in the anime series of the same name (known as "Mach Go! Go! Go!" in Japan)
- In the works of J.R.R. Tolkien, five wizards (Saruman, Gandalf, Radagast, Alatar and Pallando) are sent to Middle-Earth to aid against the threat of the Dark Lord Sauron
- In the A Song of Ice and Fire series, the War of the Five Kings is fought between different claimants to the Iron Throne of Westeros, as well to the thrones of the individual regions of Westeros (Joffrey Baratheon, Stannis Baratheon, Renly Baratheon, Robb Stark and Balon Greyjoy)
Films
- Towards the end of the film Monty Python and the Holy Grail (1975), the character of King Arthur repeatedly confuses the number five with the number three.
- Five Go Mad in Dorset (1982) was the first of the long-running series of Comic Strip Presents... television comedy films
- The Fifth Element (1997), a science fiction film
- Fast Five (2011), the fifth installment of the Fast and Furious film series.
- V for Vendetta (2005), produced by Warner Bros., directed by James McTeigue, and adapted from Alan Moore's graphic novel V for Vendetta prominently features number 5 and Roman Numeral V; the story is based on the historical event in which a group of men attempted to destroy Parliament on November 5, 1605
Music
Groups
- Five (band), a U.K. Boy band
- The Five (composers), 19th-century Russian composers
- 5 Seconds of Summer, pop band that originated in Sydney, Australia
- Five Americans, American rock band active 1965–1969
- Five Finger Death Punch, American heavy metal band from Las Vegas, Nevada. Active 2005-present
- Five Man Electrical Band, Canadian rock group billed (and active) as the Five Man Electrical Band, 1969 – 1975
- Maroon 5, American pop rock band that originated in Los Angeles, California
- MC5, American punk rock band
- Pentatonix, a Grammy-winning a cappella group originated in Arlington, Texas
- The 5th Dimension, American pop vocal group, active 1977–present
- The Dave Clark Five, a.k.a. DC5, an English pop rock group comprising Dave Clark, Lenny Davidson, Rick Huxley, Denis Payton, and Mike Smith; active 1958–1970
- The Jackson 5, American pop rock group featuring various members of the Jackson family; they were billed (and active) as The Jackson 5, 1966–1975
- We Five: American folk rock group active 1965–1967 and 1968–1977
- Grandmaster Flash and the Furious Five: American rap group, 1970-80's
- Fifth Harmony, an American girl group.
Other uses
- A perfect fifth is the most consonant harmony, and is the basis for most western tuning systems.
- Modern musical notation uses a musical staff made of five horizontal lines.
- In harmonics – the fifth partial (or 4th overtone) of a fundamental has a frequency ratio of 5:1 to the frequency of that fundamental. This ratio corresponds to the interval of 2 octaves plus a pure major third. Thus, the interval of 5:4 is the interval of the pure third. A major triad chord when played in just intonation (most often the case in a cappella vocal ensemble singing), will contain such a pure major third.
- The number of completed, numbered piano concertos of Ludwig van Beethoven, Sergei Prokofiev, and Camille Saint-Saëns.
- Using the Latin root, five musicians are called a quintet.
- A scale with five notes per octave is called a pentatonic scale.
- Five is the lowest possible number that can be the top number of a time signature with an asymmetric meter.
Television
- Stations
- Channel 5, a television channel that broadcasts in the United Kingdom
- TV5 (Former TV station ABC 5) (DWET-TV channel 5 In Metro Manila) a television network in the Philippines.
- Series
- Babylon 5, a science fiction television series created, produced and largely written by J. Michael Straczynski
- The number 5 features in the television series Battlestar Galactica in regards to the Final Five cylons and the Temple of Five
- Hi-5 (Australian TV series), a television series from Australia
- Hi-5 (British TV series), a television show from the United Kingdom
- Hi-5 Philippines (Filipino TV series) a tevevsion show from the Philippines
- Odyssey 5, a 2002 science fiction television series
- The Five: Fox News Channel roundtable current events television show, premiered 2011. So named for its panel of five commentators.
- Yes! Pretty Cure 5 is a 2007 anime series which follows the adventures of Nozomi and her friends. It is also followed by the 2008 sequel Yes! Pretty Cure 5 GoGo!
Literature
- The Famous Five is a series of children's books by British writer Enid Blyton
- The Power of Five is a series of children's books by British writer and screenwriter Anthony Horowitz
- The Fall of Five is a book written under the collective pseudonym Pittacus Lore in the series Lorien Legacies
- The Book of Five Rings is a text on kenjutsu and the martial arts in general, written by the swordsman Miyamoto Musashi circa 1645
- Slaughterhouse-Five is a book by Kurt Vonnegut about World War II
Sports
- The Olympic Games have five interlocked rings as their symbol, representing the number of inhabited continents represented by the Olympians (Europe, Asia, Africa, Australia and Oceania, and the Americas).
- In baseball scorekeeping, the number 5 represents the third baseman's position.
- In basketball:
- The number 5 is used to represent the position of center.
- Each team has five players on the court at a given time. Thus, the phrase "five on five" is commonly used to describe standard competitive basketball.
- The "5-second rule" refers to several related rules designed to promote continuous play. In all cases, violation of the rule results in a turnover.
- Five-a-side football is a variation of association football in which each team fields five players.
- In hockey, the area between the goaltender's legs is known as the five-hole.
- In most rugby leagues, the starting left wing wears this number. An exception is the European Super League, which uses static squad numbering.
- In rugby union, the starting lock forward wears number 5, and usually jumps at number 4 in the line-out.
Technology
- 5 is the most common number of gears for automobiles with manual transmission.
- In radio communication, the term "Five by five" is used to indicate perfect signal strength and clarity.
- On almost all devices with a numeric keypad such as telephones, computers, etc., the 5 key has a raised dot or raised bar to make dialing easier. Persons who are blind or have low vision find it useful to be able to feel the keys of a telephone. All other numbers can be found with their relative position around the 5 button (on computer keyboards, the 5 key of the numpad has the raised dot or bar, but the 5 key that shifts with % does not).
- On most telephones, the 5 key is associated with the letters J, K, and L, but on some of the BlackBerry phones, it is the key for G and H.
- The Pentium, coined by Intel Corporation, is a fifth-generation x86 architecture microprocessor.
- The resin identification code used in recycling to identify polypropylene.
- A pentamer is an oligomer composed of five subunits.
Miscellaneous fields


Five can refer to:
- "Give me five" is a common phrase used preceding a High five.
- An informal term for the British Security Service, MI5.
- Five babies born at one time are quintuplets. The most famous set of quintuplets were the Dionne quintuplets born in the 1930s.
- In the United States legal system, the Fifth Amendment to the United States Constitution can be referred to in court as "pleading the fifth", absolving the defendant from self-incrimination.
- Pentameter is verse with five repeating feet per line; iambic pentameter was the most popular form in Shakespeare.
- Quintessence, meaning 'fifth element', refers to the elusive fifth element that completes the basic four elements (water, fire, air, and earth)
- The designation of an Interstate Highway (Interstate 5) that runs from San Diego, California to Blaine, Washington. In addition, all major north-south Interstate Highways in the United States end in 5.
- The five basic tastes are sweet, salty, sour, bitter, and umami.
- In the computer game Riven, 5 is considered a holy number, and is a recurring theme throughout the game, appearing in hundreds of places, from the number of islands in the game to the number of bolts on pieces of machinery.
- The Garden of Cyrus (1658) by Sir Thomas Browne is a Pythagorean discourse based upon the number 5.
- The holy number of Discordianism, as dictated by the Law of Fives.
- The number of Justices on the Supreme Court of the United States necessary to render a majority decision.
- The number of dots in a quincunx.
- The number of permanent members with veto power on the United Nations Security Council.
- The number of sides and the number of angles in a pentagon.
- The number of points in a pentagram.
- The number of Korotkoff sounds when measuring blood pressure
- The drink Five Alive is named for its five ingredients. The drink punch derives its name after the Sanskrit पञ्च (pañc) for having five ingredients.
- The Keating Five were five United States Senators accused of corruption in 1989.
- The Inferior Five: Merryman, Awkwardman, The Blimp, White Feather, and Dumb Bunny. DC Comics parody superhero team.
- No. 5 is the name of the iconic fragrance created by Coco Chanel.
- The Committee of Five was delegated to draft the United States Declaration of Independence.
- The 5th U.S. President was James Monroe.
- The five-second rule is a commonly used rule of thumb for dropped food.
- 555 95472, usually referred to simply as 5, is a minor male character in the comic strip Peanuts.
- Number Five/#00.05 is a character from the comic book series The Umbrella Academy
See also
References
- ↑ "Sloane's A028388 : Good primes". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-01.
- ↑ "Sloane's A003273 : Congruent numbers". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-01.
- ↑ Bryan Bunch, The Kingdom of Infinite Number. New York: W. H. Freeman & Company (2000): 61
- ↑ Georges Ifrah, The Universal History of Numbers: From Prehistory to the Invention of the Computer transl. David Bellos et al. London: The Harvill Press (1998): 394, Fig. 24.65
- ↑ Ifrah, Georges (1998). The universal history of numbers : from prehistory to the invention of the computer. translated from the French by David Bellos ... [et al.] London: Harvill Press. ISBN 978-1-86046-324-2.
- ↑ Kisia, S. M. (2010), Vertebrates: Structures and Functions, Biological Systems in Vertebrates, CRC Press, p. 106, ISBN 9781439840528,
The typical limb of tetrapods is the pentadactyl limb (Gr. penta, five) that has five toes. Tetrapods evolved from an ancestor that had limbs with five toes. ... Even though the number of digits in different vertebrates may vary from five, vertebrates develop from an embryonic five-digit stage.
- Wells, D. The Penguin Dictionary of Curious and Interesting Numbers London: Penguin Group. (1987): 58–67
External links
 Media related to 5 (number) at Wikimedia Commons
Media related to 5 (number) at Wikimedia Commons The dictionary definition of five at Wiktionary
The dictionary definition of five at Wiktionary- The Number 5
- The Positive Integer 5
- Prime curiosities: 5
