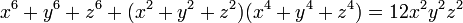Wiman's sextic

In mathematics, Wiman's sextic is a degree 6 plane curve with four nodes studied by Anders Wiman (1896). It is given by the equation (in homogeneous coordinates)

x^6+y^6+z^6 + (x^2+y^2+z^2)(x^4+y^4+z^4)=12 x^2y^2z^2

Its normalization is a genus 6 curve with automorphism group isomorphic to the symmetric group S₅.

References

Inoue, Naoki; Kato, Fumiharu (2005), "On the geometry of Wiman's sextic", Journal of Mathematics of Kyoto University 45 (4): 743–757, ISSN 0023-608X, MR 2226628
Wiman, A. (1896), "Zur Theorie der endlichen Gruppen von birationalen Transformationen in der Ebene", Mathematische Annalen 48: 195–240, doi:10.1007/BF01446342

This article is issued from Wikipedia - version of the Friday, September 26, 2014. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.