Weighing matrix
In mathematics, a weighing matrix W of order n and weight w is an n × n (0,1,-1)-matrix such that  , where
, where  is the transpose of
is the transpose of 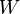 and
and  is the identity matrix of order
is the identity matrix of order  .
.
For convenience, a weighing matrix of order n and weight w is often denoted by W(n,w). A W(n,n) is a Hadamard matrix and a W(n,n-1) is equivalent to a conference matrix.
Properties
Some properties are immediate from the definition. If W is a W(n,w), then:
- The rows of W are pairwise orthogonal (that is, every pair of rows you pick from W will be orthogonal). Similarly, the columns are pairwise orthogonal.
- Each row and each column of W has exactly w non-zero elements.
-
 , since the definition means that
, since the definition means that  , where
, where  is the inverse of
is the inverse of 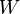 .
. -
 where
where  is the determinant of
is the determinant of 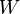 .
.
Examples
Note that when weighing matrices are displayed, the symbol  is used to represent -1. Here are two examples:
is used to represent -1. Here are two examples:
This is a W(2,2):
This is a W(7,4):
Equivalence
Two weighing matrices are considered to be equivalent if one can be obtained from the other by a series of permutations and negations of the rows and columns of the matrix. The classification of weighing matrices is complete for cases where w ≤ 5 as well as all cases where n ≤ 15 are also completed.[1] However, very little has been done beyond this with exception to classifying circulant weighing matrices.[2][3]
Open Questions
There are many open questions about weighing matrices. The main question about weighing matrices is their existence: for which values of n and w does there exist a W(n,w)? A great deal about this is unknown. An equally important but often overlooked question about weighing matrices is their enumeration: for a given n and w, how many W(n,w)'s are there?
References
- ↑ M. Harada, A. Munemasa, On the classification of weighing matrices and self-orthogonal codes, 2011, http://arxiv.org/abs/1011.5382.
- ↑ Ang, Miin Huey, et al. "Study of proper circulant weighing matrices with weight 9." Discrete Mathematics 308.13 (2008): 2802-2809.
- ↑ Arasu, K. T., et al. "Determination of all possible orders of weight 16 circulant weighing matrices." Finite Fields and Their Applications 12.4 (2006): 498-538.

