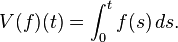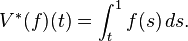Volterra operator

In mathematics, in the area of functional analysis and operator theory, the Volterra operator, named after Vito Volterra, represents the operation of indefinite integration, viewed as a bounded linear operator on the space L²(0,1) of complex-valued square integrable functions on the interval (0,1). It is the operator corresponding to the Volterra integral equations.

Definition

The Volterra operator, V, may be defined for a function f ∈ L²(0,1) and a value t ∈ (0,1), as

Properties

• V is a bounded linear operator between Hilbert spaces, with Hermitian adjoint

• V is a Hilbert–Schmidt operator, hence in particular is compact.^[1]
• V has no eigenvalues and therefore, by the spectral theory of compact operators, its spectrum σ(V) = {0}.^[1]
• V is a quasinilpotent operator (that is, the spectral radius, ρ(V), is zero), but it is not nilpotent.
• The operator norm of V is exactly ||V|| = ²⁄_π.^[1]

References