Virial mass

In astronomy, the virial mass has different meanings depending on the context.

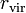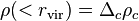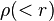In the context of dark matter halos of galaxies or galaxy clusters, virial mass refers to the mass within the virial radius $r_{\rm vir}$ , a radius within which a spherical "top hat" density perturbation destined to become a galaxy is collapsing. 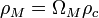This radius is defined as where $\rho(<r_{\rm vir}) = \Delta_c \rho_c$ where $\rho(<r)$ is the halo's average density within that radius, and $\rho_c$ is the critical density of the universe.^[1] (Sometimes in the definition, $\rho_c$ is replaced with the mean density of matter, $\rho_M = \Omega_M \rho_c$ , where, at the present day, $\Omega_M \simeq 0.27$ according to data fitted to the Lambda-CDM model.) The virial mass is the mass within this radius and hence is a reasonable measure of the total mass inside a dark matter halo, because beyond that radius the halo blends into the background matter in the universe. This definition is not universal, however, as the exact value of 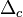$\Delta_c$ depends on the cosmology – in practice, to improve communication, it is sometimes simply assumed that 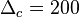$\Delta_c = 200$ and hence this is sometimes denoted as 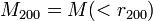$M_{200} = M(<r_{200})$ , or the total mass contained within the virial radius.

In other contexts, it may refer to the mass inferred from the rotation curve or velocity dispersion of a bound collection of stars, assuming the virial theorem applies.

References

↑ White, M (3 February 2001). "The mass of a halo". A&A 367 (1): 27–32. Bibcode:2001A&A...367...27W. doi:10.1051/0004-6361:20000357. Retrieved 30 November 2014.

This article is issued from Wikipedia - version of the Monday, October 05, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.