Varignon's theorem (mechanics)
The Varignon Theorem is a theorem by French mathematician Pierre Varignon (1654-1722), published in 1687 in his book Projet d' unè nouvelle mèchanique. The theorem states that the moment of a resultant of two concurrent forces about any point is equal to the algebraic sum of the moments of its components about the same point. In other words, "If many coplanar forces are acting on a body, then algebraic sum of moments of all the forces about a point in the plane of the forces is equal to the moment of their resultant about the same point."[1]
Proof
For a set n of vectors 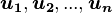 that concurs at point O in space. The resultant is:
that concurs at point O in space. The resultant is:

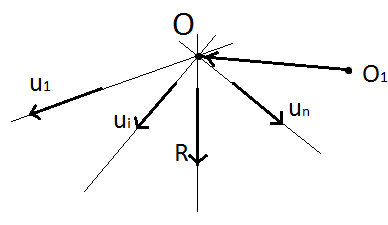
The moment of each vector is:
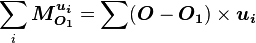
In terms of summary, taking out the common factor 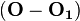 , and considering the expression of R, results:
, and considering the expression of R, results:
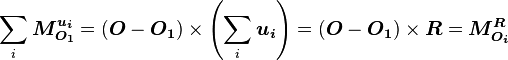
References
- ↑ I. C. Jong, B. G. Rogers (1991). "Engineering Mechanics: Statics".
External links
- Varirgnon's Theorem at TheFreeDictionary.com
- Proof. Mechanics of Solids. S.S. Bhavikatti. (page 19)