Turnaround (music)

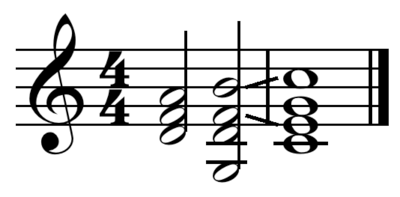
This article is about the musical technique. For other music-related uses, see Turnaround (disambiguation).
ii-V7-I turnaround in C  Play 

In jazz, a turnaround is a passage at the end of a section which leads to the next section. This next section is most often the repetition of the previous section or the entire piece or song.[1]

The turnaround may lead back to this section either harmonically, as a chord progression, or melodically.

Typical turnarounds

I-vi7-ii-V7 turnaround in C[2]  Play .
III-VI-II-V turnaround often appears in the bridge of jazz standards.[3]  Play 
Tadd Dameron turnaround with resolution.  Play 

Typical turnarounds in jazz include:

Turnarounds typically begin with the tonic (I) and end on the dominant (V7), the next section starting on the tonic (I). They may also end on ♭II7 (dominant substitute).[6] Thus when used in a twelve bar blues pattern, the twelfth bar may end on the dominant.[1] All of the chords in a turnaround may be seventh chords.

Harmonic alternatives

Sometimes, especially in blues music, musicians will take chords which are normally minor chords and make them major. The most popular example is the I - VI - ii - V - (I) progression; normally, the vi chord would be a minor chord (min, -7, -6, -(♭6), etc.) but here the major third allows for a more interesting modulation. Take the example in C major: C - A - d min - G (dom) . The third of the VI chord (in this case, C♯) allows for chromatic movement from C (the root of I) to C♯ (the third of VI) to D (the root of ii).

Similar chromaticism and harmonic interest can be achieved by the use of a secondary dominant, which is also useful for turnarounds. The simplest example is V7/V - V7 - I, instead of ii - V - I. Another popular turnaround which may be considered as a secondary dominant analysis is ii - ♭V/V (or ♭II) - I, which is a variation on the standard ii - V - I turnaround. In jazz parlance, use of the ♭II instead of the V is known as tritone substitution. Using ♭V/V instead of V allows for a smooth chromatic descent. Again, let's examine C major; the original turnaround would be d min - G (dom) - C, while the modified would be d min - D♭ - C . The obvious chromatic movement is thorough; it is apparent in the roots (D - D♭ - C), thirds (F - F - E; F is often used as a pedal tone), and fifths (A - A♭ - G).

While in that particular example the ♭V/V can be considered a Neapolitan chord, the more typical functional analysis in the context of the jazz idiom is that it is not a "secondary dominant" (♭V7/V) at all, but ♭II7, a substitute dominant[7] (tritone substitution). Harmonically, ♭II7 functions exactly as V7/I does, because the two chords enharmonically contain the same tritone, which is the critical harmonic element in the resolution from dominant to tonic. The half step downward motion of the roots of those chords, as seen in ii - ♭II7 - I, forms the familiar "line cliché", arriving satisfyingly at the tonic.

NB: "Secondary dominant" refers to the functional dominant of the key's dominant or another non-tonic chord, while "substitute dominant" refers to an alternative functional dominant of the key's tonic. The extending of dominants to secondaries (or beyond) is a practice which remains firmly inside the circle of fifths, while the substitution of dominants replaces that cycle with one of minor-second intervals.

I-vi-ii-V may be transformed through various chord substitutions. For example, the vi and ii chords may be substituted with dominant chords, giving I-VI7-II7-V or C-A7-D7-G,[8] the Ragtime progression. The tritone substitution may be applied to the vi and V chords, giving C-E♭7-D7-D♭7, or to every chord but the I, giving C-E♭7-A♭M7-D♭7.[9]

See also

References

  1. 1 2 Randel, Don Michael (2002). The Harvard Concise Dictionary of Music and Musicians. ISBN 0-674-00978-9. p.693
  2. 1 2 Boyd, Bill (1997). Jazz Chord Progressions, p.43. ISBN 0-7935-7038-7.
  3. ↑ Boyd, Bill (1997). Jazz Chord Progressions, p.56. ISBN 0-7935-7038-7.
  4. ↑ Boyd (1997), p.86.
  5. ↑ Boyd (1997), p.90.
  6. ↑ Coker, et al (1982). Patterns for Jazz: A Theory Text for Jazz Composition and Improvisation, p.118. ISBN 0-89898-703-2.
  7. ↑ , . Harmony 4 course book, . Berklee College of Music.
  8. ↑ Boyd (1997), p.44.
  9. ↑ Boyd (1997), p.46-47.
This article is issued from Wikipedia - version of the Monday, November 16, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.