Trillium theorem

In Euclidean geometry, the trillium theorem – (from Russian: лемма о трезубце,^[1]^[2] literally 'lemma about trident', Russian: теорема трилистника,^[3] literally 'theorem of trillium' or 'theorem of trefoil') is a statement about properties of inscribed and circumscribed circles and their relations.

Theorem

Trillium theorem

Let $ABC$ be an arbitrary triangle. Let $I$ be its incenter and let $D$ be the point where line $BI$ (the angle bisector of $∠ ABC$ ) crosses the circumcircle of $ABC$ . Then, the theorem states that $D$ is equidistant from $A$ , $C$ , and $I$ . Equivalently:

The circle through $A$ , $C$ , and $I$ has its center at $D$ . In particular, this implies that the center of this circle lies on the circumcircle.^[4]^[5]
The three triangles $AID$ , $CID$ , and $ACD$ are isosceles, with $D$ as their apex.

A fourth point, the excenter of $ABC$ relative to $B$ , also lies at the same distance from $D$ , diametrally opposite from $I$ .^[6]^[2]

Proof

By the inscribed angle theorem,

|\angle ABI| = |\angle DCA|, \quad |\angle CBI| = |\angle DAC|.

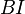Since $BI$ is angle bisector,

\begin{align} & |\angle DCA| = |\angle DAC| \\[6pt] \Rightarrow {} & |AD| = |CD|, \text{ and } |\angle DIA| = 180^\circ - |\angle AIB| \\[6pt] = {} & 180^\circ - (180^\circ - |\angle IAB| - |\angle IBA|) \\[6pt] = {} & |\angle IAB| + |\angle IBA|= |\angle IAC| + |\angle CAD| \\[6pt] = {} & |\angle IAD| \\[6pt] \Rightarrow {} & |AD| = |DI|. \end{align}

Application to triangle reconstruction

This theorem can be used to reconstruct a triangle starting from the locations only of one vertex, the incenter, and the circumcenter of the triangle. For, let $B$ be the given vertex, $I$ be the incenter, and $O$ be the circumcenter. This information allows the successive construction of:

the circumcircle of the given triangle, as the circle with center $O$ and radius $OB$ ,
point $D$ as the intersection of the circumcircle with line $BI$ ,
the circle of the trillium theorem, with center $D$ and radius $DI$ , and
vertices $A$ and $C$ as the intersection points of the two circles.^[7]

However, for some triples of points $B$ , $I$ , and $O$ , this construction may fail, either because line $IB$ is tangent to the circumcircle or because the two circles do not have two crossing points. It may also produce a triangle for which the given point $I$ is an excenter rather than the incenter. In these cases, there can be no triangle having $B$ as vertex, $I$ as incenter, and $O$ as circumcenter.^[8]

Other triangle reconstruction problems, such as the reconstruction of a triangle from a vertex, incenter, and center of its nine-point circle, can be solved by reducing the problem to the case of a vertex, incenter, and circumcenter.^[8]

Generalization

Let $I$ and $J$ be any two of the four points given by the incenter and the three excenters of a triangle $ABC$ . Then $I$ and $J$ are collinear with one of the three triangle vertices. The circle with $IJ$ as diameter passes through the other two vertices and is centered on the circumcircle of $ABC$ . When one of $I$ or $J$ is the incenter, this is the trillium theorem, with line $IJ$ as the (internal) angle bisector of one of the triangle's angles. However, it is also true when $I$ and $J$ are both excenters; in this case, line $IJ$ is the external angle bisector of one of the triangle's angles.^[9]

References

↑ Р. Н. Карасёв, В. Л. Дольников, И. И. Богданов, А. В. Акопян. Задачи для школьного математического кружка (PDF). Problem 1.2. p. 4.
1 2 "6. Лемма о трезубце" (PDF). СУНЦ МГУ им. М. В. Ломоносова - школа им. А.Н. Колмогорова. 2014-10-29.
↑ И. А. Кушнир. "Это открытие - золотой ключ Леонарда Эйлера" (PDF). Ф7 (Теорема трилистника), page 34; proof on page 36.
↑ Morris, Richard (1928), "Circles through notable points of the triangle", The Mathematics Teacher 21 (2): 63–71, JSTOR 27951001 . See in particular the discussion on p. 65 of circles $BIC$ , $CIA$ , $AIB$ , and their centers.
↑ Bogomolny, Alexander, "A Property of Circle Through the Incenter", Cut-the-Knot, retrieved 2016-01-26 .
↑ Bogomolny, Alexander, "Midpoints of the Lines Joining In- and Excenters", Cut-the-Knot, retrieved 2016-01-26 .
↑ Aref, M. N.; Wernick, William (1968), Problems and Solutions in Euclidean Geometry, Dover Books on Mathematics, Dover Publications, Inc., 3.3(i), p. 68, ISBN 9780486477206 .
1 2 Yiu, Paul (2012), "Conic construction of a triangle from its incenter, nine-point center, and a vertex" (PDF), Journal for Geometry and Graphics 16 (2): 171–183, MR 3088369
↑ Chou, Shang-Ching; Gao, Xiao-Shan; Zhang, Jingzhong (1994), Machine Proofs in Geometry: Automated Production of Readable Proofs for Geometry Theorems, Series on applied mathematics 6, World Scientific, Examples 6.145 and 6.146, pp. 328–329, ISBN 9789810215842 .

External links

Weisstein, Eric W., "Incenter-Excenter Circle", MathWorld.

This article is issued from Wikipedia - version of the Wednesday, January 27, 2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.

Trillium theorem

Theorem

Proof

Application to triangle reconstruction

Generalization

See also

References

External links