Trilinear polarity

In geometry, trilinear polarity is a certain correspondence between the points in the plane of a triangle not lying on the sides of the triangle and lines in the plane of the triangle not passing through the vertices of the triangle. "Although it is called a polarity, it is not really a polarity at all, for poles of concurrent lines are not collinear lines."[1] It was Poncelet (1788–1867), a French engineer and mathematician, who introduced the idea of the trilinear polar of a point in 1865.[1][2]

Definitions

Diagram illustrating the definition of the trilinear polar of a point.

Let ABC be a plane triangle and let P be any point in the plane of the triangle not lying on the sides of the triangle. Briefly, the trilinear polar of P is the axis of perspectivity of the cevian triangle of P and the triangle ABC.

In detail, let the line AP, BP, CP meet the sideliness BC, CA, AB at D, E, F respectively. Triangle DEF is the cevian triangle of P with reference to triangle ABC. Let the pairs of line (BC, EF), (CA, FD), (DE, AB) intersect at X, Y, Z respectively. By Desargues' theorem the points X, Y, Z are collinear. The line of collinearity is the axis of perspectivity of triangle ABC and triangle DEF. The line XYZ is the trilinear polar of the point P.[1]

The points X, Y, Z can also be obtained as the harmonic conjugates of D, E, F with respect to the pairs of points (B,C), (C, A), (A, B) respectively. Poncelet used this idea to define the concept of trilinear polars.[1]

If the line L is the trilinear polar of the point P with respect to the reference triangle ABC then P is called the trilinear pole of the line L with respect to the reference triangle ABC.

Trilinear equation

Let the trilinear coordinates of the point P be (p : q : r). Then the trilinear equation of the trilinear polar of P is[3]

x / p + y / q + z / r = 0.

Construction of the trilinear pole

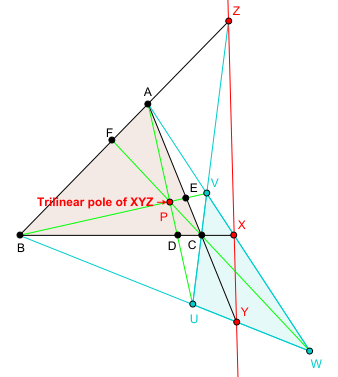
Diagram illustrating the construction of the trilinear pole of a given line XYZ

Let the line L meet the sides BC, CA, AB of triangle ABC at X, Y, Z respectively. Let the pairs of lines (BY, CZ), (CZ, AX), (AX, BY) meet at U, V, W. Triangles ABC and UVW are in perspective and let P be the center of perspectivity. P is the trilinear pole of the line L.

Some trilinear polars

Some of the trilinear polars are well known.[4]

Poles of pencils of lines

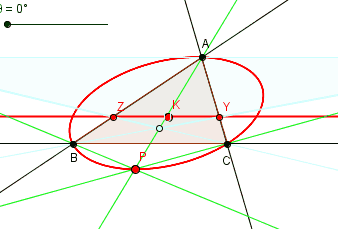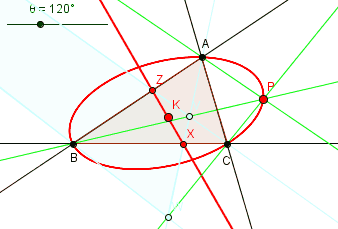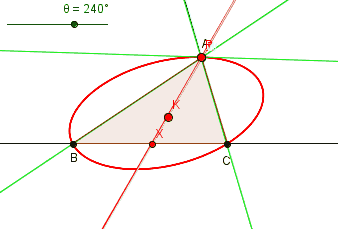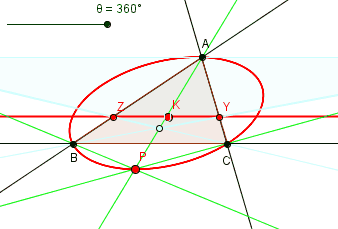
Animation illustrating the fact that the locus of the trilinear poles of a pencil of lines passing through a fixed point K is a circumconic of the reference triangle.

Let P with trilinear coordinates ( X : Y : Z ) be the pole of a line passing through a fixed point K with trilinear coordinates ( x0 : y0 : z0 ). Equation of the line is

Since this passes through K,

Thus the locus of P is

This is a circumconic of the triangle of reference ABC. Thus the locus of the poles of a pencil of lines passing through a fixed point is a circumconic of the triangle of reference.

References

  1. 1 2 3 4 Coxeter, H.S.M. (1993). The Real Projective Plane. Springer. pp. 102–103. ISBN 9780387978895.
  2. ↑ Coxeter, H.S.M. (2003). Projective Geometry. Springer. p. 29. ISBN 9780387406237.
  3. ↑ Weisstein, Eric W. "Trilinear Polar". MathWorld—A Wolfram Web Resource. Retrieved 31 July 2012.
  4. ↑ Weisstein, Eric W. "Trilinear Pole". MathWorld—A Wolfram Web Resource. Retrieved 8 August 2012.

External links

This article is issued from Wikipedia - version of the Wednesday, February 03, 2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.