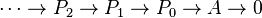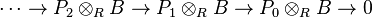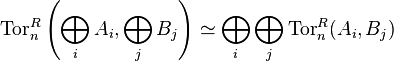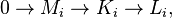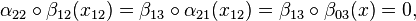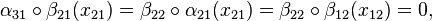Tor functor
In homological algebra, the Tor functors are the derived functors of the tensor product functor. They were first defined in generality to express the Künneth theorem and universal coefficient theorem in algebraic topology.
Specifically, suppose R is a ring, and denote by R-Mod the category of left R-modules and by Mod-R the category of right R-modules (if R is commutative, the two categories coincide). Pick a fixed module B in R-Mod. For A in Mod-R, set T(A) = A ⊗R B. Then T is a right exact functor from Mod-R to the category of abelian groups Ab (in the case when R is commutative, it is a right exact functor from Mod-R to Mod-R) and its left derived functors LnT are defined. We set
i.e., we take a projective resolution
then remove the A term and tensor the projective resolution with B to get the complex
(note that A ⊗R B does not appear and the last arrow is just the zero map) and take the homology of this complex.
Properties
- For every n ≥ 1, TorR
n is an additive functor from Mod-R × R-Mod to Ab. In the case when R is commutative, we have additive functors from Mod-R × Mod-R to Mod-R.
- As is true for every family of derived functors, every short exact sequence 0 → K → L → M → 0 induces a long exact sequence of the form
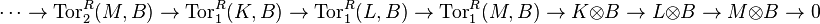 .
.
- If R is commutative and r in R is not a zero divisor then
- from which the terminology Tor (that is, Torsion) comes: see torsion subgroup.
- TorZ
n(A, B) = 0 for all n ≥ 2. The reason: every abelian group A has a free resolution of length 1, since subgroups of free abelian groups are free abelian. So in this important special case, the higher Tor functors vanish. In addition, TorZ
1(Z/kZ, A) = Ker(f) where f: A → A represents "multiplication by k".
- Furthermore, every free module has a free resolution of length zero, so by the argument above, if F is a free R-module, then TorR
n(F, B) = 0 for all n ≥ 1.
- The Tor functors preserve filtered colimits and arbitrary direct sums: there is a natural isomorphism
- From the classification of finitely generated abelian groups, we know that every finitely generated abelian group is the direct sum of copies of Z and Zk. This together with the previous three points allows us to compute TorZ
1(A, B) whenever A is finitely generated.
- A module M in Mod-R is flat if and only if TorR
1(M, -) = 0. In this case, we even have TorR
n(M, -) = 0 for all n ≥ 1 . In fact, to compute TorR
n(A, B), one may use a flat resolution of A or B, instead of a projective resolution (note that a projective resolution is automatically a flat resolution, but the converse isn't true, so allowing flat resolutions is more flexible).
- Symmetry: if R is commutative, then there is a natural isomorphism TorR
n(L
1, L
2) ≅ TorR
n(L
2, L
1). Here is the idea for abelian groups (i.e., the case R = Z and n = 1). Fix a free resolution of L
i as follows
- so that M
i and K
i are free abelian groups. This gives rise to a double-complex with exact rows and columns

- Start with x ∈ TorZ
1(L
1, L
2), so β03(x) ∈ Ker(β13). Let x
12 ∈ M
1 ⊗ K
2 be such that α12(x
12) = β03(x). Then
- i.e., β12(x
12) ∈ Ker(α22). By exactness of the second row, this means that β12(x
12) = α21(x
21) for some unique x
21 ∈ K
1 ⊗ M
2. Then
- i.e., β21(x
21) ∈ Ker(α31). By exactness of the bottom row, this means that β21(x
21) = α30(y) for some unique y ∈ TorZ
1(L
2, L
1).
- Upon checking that y is uniquely determined by x (not dependent on the choice of x
12), this defines a function TorZ
n(L
1, L
2) → TorZ
n(L
2, L
1), taking x to y, which is a group homomorphism. One may check that this map has an inverse, namely the function TorZ
n(L
2, L
1) → TorZ
n(L
1, L
2) defined in a similarly manner. One can also check that the function does not depend on the choice of free resolutions.
See also
References
- Weibel, Charles A. (1994), An introduction to homological algebra, Cambridge Studies in Advanced Mathematics 38, Cambridge University Press, ISBN 978-0-521-55987-4, OCLC 36131259, MR 1269324