Taylor state

In plasma physics, a Taylor state is the minimum energy state of a plasma satisfying the constraint of conserving magnetic helicity.[1]

Derivation

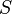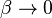Consider a closed, simply-connected, flux-conserving, perfectly conducting surface S surrounding a plasma with negligible thermal energy (\beta \rightarrow 0).

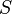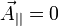Since \vec{B}\cdot\vec{ds}=0 on S. This implies that \vec{A}_{||}=0.

As discussed above, the plasma would relax towards a minimum energy state while conserving its magnetic helicity. Since the boundary is perfectly conducting, there cannot be any change in the associated flux. This implies 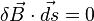\delta \vec{B}\cdot\vec{ds}=0 and \delta\vec{A}_{||}=0 on S.

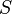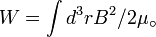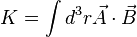We formulate a variational problem of minimizing the plasma energy W=\int d^3rB^2/2\mu_\circ while conserving magnetic helicity K=\int d^3r\vec{A}\cdot\vec{B}.

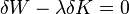The variational problem is \delta W -\lambda \delta K = 0.

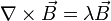After some algebra this leads to the following constraint for the minimum energy state \nabla \times \vec{B} = \lambda \vec{B}.

See also

References

  1. ↑ Paul M. Bellan (2000). Spheromaks: A Practical Application of Magnetohydrodynamic dynamos and plasma self-organization. pp. 71–79. ISBN 1-86094-141-9.


This article is issued from Wikipedia - version of the Thursday, April 30, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.