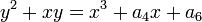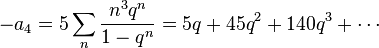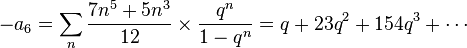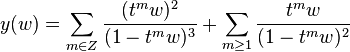Tate curve

In mathematics, the Tate curve is a curve defined over the ring of formal power series $\mathbb {Z} [[q]]$ with integer coefficients. Over the open subscheme where q is invertible, the Tate curve is an elliptic curve. The Tate curve can also be defined for q as an element of a complete field of norm less than 1, in which case the formal power series converge.

The Tate curve was introduced by John Tate (1995) in a 1959 manuscript originally titled "Rational Points on Elliptic Curves Over Complete Fields"; he did not publish his results until many years later, and his work first appeared in Roquette (1970).

Definition

The Tate curve is the projective plane curve over the ring Z''q'' of formal power series with integer coefficients given (in an affine open subset of the projective plane) by the equation

y^{2}+xy=x^{3}+a_{4}x+a_{6}

where

-a_{4}=5\sum _{n}{\frac {n^{3}q^{n}}{1-q^{n}}}=5q+45q^{2}+140q^{3}+\cdots

-a_{6}=\sum _{n}{\frac {7n^{5}+5n^{3}}{12}}\times {\frac {q^{n}}{1-q^{n}}}=q+23q^{2}+154q^{3}+\cdots

are power series with integer coefficients.^[1]

The Tate curve over a complete field

Suppose that the field k is complete with respect to some absolute value | |, and q is a non-zero element of the field k with |q|<1. Then the series above all converge, and define an elliptic curve over k. If in addition q is non-zero then there is an isomorphism of groups from k^*/q^Z to this elliptic curve, taking w to (x(w),y(w)) for w not a power of q, where

x(w)=-y(w)-y(w^{-1})

y(w)=\sum _{m\in Z}{\frac {(t^{m}w)^{2}}{(1-t^{m}w)^{3}}}+\sum _{m\geq 1}{\frac {t^{m}w}{(1-t^{m}w)^{2}}}

and taking powers of q to the point at infinity of the elliptic curve. The series x(w) and y(w) are not formal power series in w.

Intuitive example

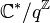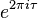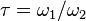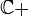In the case of the curve over the complete field, $k^{*}/q^{\mathbb {Z} }$ , the easiest case to visualize is $\mathbb {C} ^{*}/q^{\mathbb {Z} }$ , where $q$ is the discrete subgroup generated by one multiplicative period $e^{2\pi i\tau }$ , where the period $\tau =\omega _{1}/\omega _{2}$ . Note that $\mathbb {C} ^{*}$ is isomorphic to $\mathbb {C} +/\mathbb {Z} +$ , where $\mathbb {C} +$ is the complex numbers under addition.

To see why the Tate Curve morally corresponds to a torus when the field is C with the usual norm, $q$ is already singly periodic; modding out by q's integral powers you are modding out $\mathbb {C}$ by $\mathbb {Z} ^{2}$ , which is a torus. In other words, we have an annulus, and we glue inner and outer edges.

But the annulus does not correspond to the circle minus a point: the annulus is the set of complex numbers between two consecutive powers of q; say all complex numbers with magnitude between 1 and q. That gives us two circles, i.e., the inner and outer edges of an annulus.

The image of the torus given here is a bunch of inlaid circles getting narrower and narrower as they approach the origin.

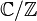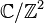This is slightly different from the usual method beginning with a flat sheet of paper, $\mathbb {C}$ , and gluing together the sides to make a cylinder $\mathbb {C} /\mathbb {Z}$ , and then gluing together the edges of the cylinder to make a torus, $\mathbb {C} /\mathbb {Z} ^{2}$ .

This is slightly oversimplified. The Tate curve is really a curve over a formal power series ring rather than a curve over C. Intuitively, it's a family of curves depending on a formal parameter (when that formal parameter is zero it degenerates to a pinched torus, and when it's nonzero it's a torus).

Properties

The j-invariant of the Tate curve is given by a power series in q with leading term q⁻¹.^[2] Over a p-adic local field, therefore, j is non-integral and the Tate curve has semistable reduction of multiplicative type. Conversely, every semistable elliptic curve over a local field is isomorphic to a Tate curve (up to quadratic twist).^[3]

References

↑ Manin & Panchishkin (2007) p.220
↑ Silverman (1994) p.423
↑ Manin & Panchiskin (2007) p.300

Lang, Serge (1987), Elliptic functions, Graduate Texts in Mathematics 112 (2nd ed.), Berlin, New York: Springer-Verlag, ISBN 978-0-387-96508-6, MR 890960, Zbl 0615.14018
Manin, Yu. I.; Panchishkin, A. A. (2007). Introduction to Modern Number Theory. Encyclopaedia of Mathematical Sciences 49 (Second ed.). ISBN 978-3-540-20364-3. ISSN 0938-0396. Zbl 1079.11002.
Robert, Alain (1973), Elliptic curves, Lecture Notes in Mathematics 326, Berlin, New York: Springer-Verlag, doi:10.1007/978-3-540-46916-2, ISBN 978-3-540-06309-4, MR 0352107, Zbl 0256.14013
Roquette, Peter (1970), Analytic theory of elliptic functions over local fields, Hamburger Mathematische Einzelschriften (N.F.), Heft 1, Göttingen: Vandenhoeck & Ruprecht, MR 0260753, Zbl 0194.52002
Silverman, Joseph H. (1994). Advanced Topics in the Arithmetic of Elliptic Curves. Graduate Texts in Mathematics 151. Springer-Verlag. ISBN 0-387-94328-5. Zbl 0911.14015.
Tate, John (1995) [1959], "A review of non-Archimedean elliptic functions" (PDF), in Coates, John; Yau, Shing-Tung, Elliptic curves, modular forms, & Fermat's last theorem (Hong Kong, 1993), Series in Number Theory I, Int. Press, Cambridge, MA, pp. 162–184, ISBN 978-1-57146-026-4, MR 1363501

This article is issued from Wikipedia - version of the Monday, December 14, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.