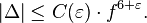Szpiro's conjecture

In number theory, Szpiro's conjecture concerns a relationship between the conductor and the discriminant of an elliptic curve. In a general form, it is equivalent to the well-known abc conjecture. It is named for Lucien Szpiro who formulated it in the 1980s.

The conjecture states that: given ε > 0, there exists a constant C(ε) such that for any elliptic curve E defined over Q with minimal discriminant Δ and conductor f, we have

\vert\Delta\vert \leq C(\varepsilon ) \cdot f^{6+\varepsilon }. \,

The modified Szpiro conjecture states that: given ε > 0, there exists a constant C(ε) such that for any elliptic curve E defined over Q with invariants c₄, c₆ and conductor f (see Tate's algorithm#Notation), we have

\max\{\vert c_4\vert^3,\vert c_6\vert^2\} \leq C(\varepsilon )\cdot f^{6+\varepsilon }. \,

References

Lang, S. (1997), Survey of Diophantine geometry, Berlin: Springer-Verlag, p. 51, ISBN 3-540-61223-8, Zbl 0869.11051
Szpiro, L. (1981), "Seminaire sur les pinceaux des courbes de genre au moins deux", Astérisque 86 (3): 44–78, Zbl 0463.00009
Szpiro, L. (1987), "Présentation de la théorie d'Arakelov", Contemp. Math. 67: 279–293, doi:10.1090/conm/067/902599, Zbl 0634.14012

This article is issued from Wikipedia - version of the Sunday, January 10, 2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.