Symplectic basis
In linear algebra, a standard symplectic basis is a basis 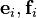 of a symplectic vector space, which is a vector space with a nondegenerate alternating bilinear form
of a symplectic vector space, which is a vector space with a nondegenerate alternating bilinear form  , such that
, such that 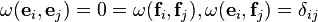 . A symplectic basis of a symplectic vector space always exists; it can be constructed by a procedure similar to the Gram–Schmidt process.[1] The existence of the basis implies in particular that the dimension of a symplectic vector space is even if it is finite.
. A symplectic basis of a symplectic vector space always exists; it can be constructed by a procedure similar to the Gram–Schmidt process.[1] The existence of the basis implies in particular that the dimension of a symplectic vector space is even if it is finite.
See also
Notes
- ↑ Maurice de Gosson: Symplectic Geometry and Quantum Mechanics (2006), p.7 and pp. 12–13
References
- da Silva, A.C., Lectures on Symplectic Geometry, Springer (2001). ISBN 3-540-42195-5.
- Maurice de Gosson: Symplectic Geometry and Quantum Mechanics (2006) Birkhäuser Verlag, Basel ISBN 978-3-7643-7574-4.
This article is issued from Wikipedia - version of the Thursday, September 24, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.