Structurable algebra

In abstract algebra, a structurable algebra is a certain kind of unital involutive non-associative algebra over a field. For example, all Jordan algebras are structurable algebras (with the trivial involution), as is any alternative algebra with involution, or any central simple algebra with involution. An involution here means a linear anti-homomorphism whose square is the identity.[1]

Assume A is a unital non-associative algebra over a field, and 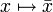x \mapsto \bar{x} is an involution. If we define V_{x,y}z:=(x\bar{y})z+(z\bar{y})x-(z\bar{x})y, and [x,y]=xy-yx, then we say A is a structurable algebra if: [2]

[V_{x,y} , V_{z,w}] = V_{V_{x,y}z,w} - V_{z,V_{y,x}w}.

Structurable algebras were introduced by Allison in 1978.[3] The Kantor–Koecher–Tits construction produces a Lie algebra from any Jordan algebra, and this construction can be generalized so that a Lie algebra can be produced from an structurable algebra. Moreover, Allison proved over fields of characteristic zero that a structurable algebra is central simple if and only if the corresponding Lie algebra is central simple.[1]

Another example of a structurable algebra is a 56-dimensional non-associative algebra originally studied by Brown in 1963, which can be constructed out of an Albert algebra.[4] When the base field is algebraically closed over characteristic not 2 or 3, the automorphism group of such an algebra has identity component equal to the simply connected exceptional algebraic group of type E6.[5]

References

  1. 1 2 R.D Schafer (1985). "On Structurable algebras". Journal of Algebra 92. pp. 400–412.
  2. ↑ Skip Garibaldi (2001). "Structurable Algebras and Groups of Type E_6 and E_7". Journal of Algebra 236. pp. 651–691.
  3. ↑ Garibaldi, p.658
  4. ↑ R. B. Brown (1963). "A new type of nonassociative algebra" 50. Proc. Nat. Acad. Sci. U.S. A. pp. 947–949.
  5. ↑ Garibaldi, p.660
This article is issued from Wikipedia - version of the Friday, March 13, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.