Specific activity
Specific activity is the activity per quantity of a radionuclide and is a physical property of that radionuclide.[1][2]
Activity is a quantity related to radioactivity. The SI unit of activity is the becquerel (Bq), equal to one reciprocal second.[3]
Since the probability of radioactive decay for a given radionuclide is a fixed physical quantity (with some slight exceptions, see Changing decay rates), the number of decays that occur in a given time of a specific number of atoms of that radionuclide is also a fixed physical quantity (if there are large enough numbers of atoms to ignore statistical fluctuations).
Thus, specific activity is defined as the activity per quantity of atoms of a particular radionuclide. It is usually given in units of Bq/g, but another commonly used unit of activity is the curie (Ci) allowing the definition of specific activity in Ci/g.
Half-life
Experimentally-measured specific activity can be used to calculate the half-life of a radionuclide.
Half-life (T1/2) is defined as the length of time for half of a given quantity of radioactive atoms to undergo radioactive decay: Or more generally: Starting with N0, atoms of an element, the number of atoms, N, remaining after time, t, is given by:
The natural log of both sides
The derivative with respect to time, t
Multiplying both sides by N
Yields
dN/dt represents the decay rate of atoms. The negative sign shows that the rate is negative, so the number of atoms is decreasing with time. Rearranging terms:
Example: half-life of Rb-87
One gram of rubidium-87 and a radioactivity count rate that, after taking solid angle effects into account, is consistent with a decay rate of 3200 decays per second corresponds to a specific activity of 3.2×106 Bq/kg. Rubidium's atomic weight is 87, so one gram is one 87th of a mole, or N=6.9×1021 atoms. Plugging in the numbers:
Formulation
Radioactivity is expressed as the decay rate of a particular radionuclide with decay constant λ and the number of atoms N:
Mass of the radionuclide is given by
where m is mass number of the radionuclide and NA is Avogadro's constant.
Specific radioactivity a is defined as radioactivity per unit mass of the radionuclide:
In addition, decay constant λ is related to the half-life T1/2 by the following equation:
Thus, specific radioactivity can also be described by
This equation is simplified by
When the unit of half-life converts a year
For example, specific radioactivity of radium 226 with a half-life of 1600 years is obtained by
This value derived from radium 226 was defined as unit of radioactivity known as Curie (Ci).
Another two examples are specific radioactivity of thorium 232 and specific radioactivity of potassium 40:
Applications
The specific activity of radionuclides is particularly relevant when it comes to select them for production for therapeutic pharmaceuticals, as well as for immunoassays or other diagnostic procedures, or assessing radioactivity in certain environments, among several other biomedical applications.[4][5][6][7][8][9]
References
- ↑ Breeman, Wouter A. P.; Jong, Marion; Visser, Theo J.; Erion, Jack L.; Krenning, Eric P. (2003). "Optimising conditions for radiolabelling of DOTA-peptides with 90Y, 111In and 177Lu at high specific activities". European Journal of Nuclear Medicine and Molecular Imaging 30 (6): 917–920. doi:10.1007/s00259-003-1142-0. ISSN 1619-7070.
- ↑ de Goeij, J. J. M.; Bonardi, M. L. (2005). "How do we define the concepts specific activity, radioactive concentration, carrier, carrier-free and no-carrier-added?". Journal of Radioanalytical and Nuclear Chemistry 263 (1): 13–18. doi:10.1007/s10967-005-0004-6. ISSN 0236-5731.
- ↑ "SI units for ionizing radiation: becquerel". Resolutions of the 15th CGPM (Resolution 8). 1975. Retrieved 3 July 2015.
- ↑ Duursma, E. K. "Specific activity of radionuclides sorbed by marine sediments in relation to the stable element composition." Radioactive contamination of the marine environment (1973): 57-71.
- ↑ Wessels, Barry W. (1984). "Radionuclide selection and model absorbed dose calculations for radiolabeled tumor associated antibodies". Medical Physics 11 (5): 638. doi:10.1118/1.595559. ISSN 0094-2405.
- ↑ I. Weeks, I. Beheshti, F. McCapra, A. K. Campbell & J. S. Woodhead (August 1983). "Acridinium esters as high-specific-activity labels in immunoassay". Clinical chemistry 29 (8): 1474–1479. PMID 6191885.
- ↑ Neves, M; Kling, A; Lambrecht, R.M (2002). "Radionuclide production for therapeutic radiopharmaceuticals". Applied Radiation and Isotopes 57 (5): 657–664. doi:10.1016/S0969-8043(02)00180-X. ISSN 0969-8043.
- ↑ Mausner, Leonard F. (1993). "Selection of radionuclides for radioimmunotherapy". Medical Physics 20 (2): 503. doi:10.1118/1.597045. ISSN 0094-2405.
- ↑ Murray, A. S.; Marten, R.; Johnston, A.; Martin, P. (1987). "Analysis for naturally occuring radionuclides at environmental concentrations by gamma spectrometry". Journal of Radioanalytical and Nuclear Chemistry Articles 115 (2): 263–288. doi:10.1007/BF02037443. ISSN 0236-5731.
Further Reading
- Fetter, Steve; Cheng, E.T; Mann, F.M (1990). "Long-term radioactive waste from fusion reactors: Part II". Fusion Engineering and Design 13 (2): 239–246. doi:10.1016/0920-3796(90)90104-E. ISSN 0920-3796.
- Holland, Jason P.; Sheh, Yiauchung; Lewis, Jason S. (2009). "Standardized methods for the production of high specific-activity zirconium-89". Nuclear Medicine and Biology 36 (7): 729–739. doi:10.1016/j.nucmedbio.2009.05.007. ISSN 0969-8051.
- McCarthy, Deborah W.; Shefer, Ruth E.; Klinkowstein, Robert E.; Bass, Laura A.; Margeneau, William H.; Cutler, Cathy S.; Anderson, Carolyn J.; Welch, Michael J. (1997). "Efficient production of high specific activity 64Cu using a biomedical cyclotron". Nuclear Medicine and Biology 24 (1): 35–43. doi:10.1016/S0969-8051(96)00157-6. ISSN 0969-8051.


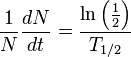



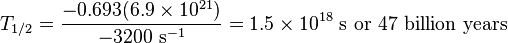
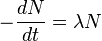
![\frac{N}{N_A} [\text{mol}] \times {m} [\text {g } \text{mol}^{-1}]](../I/m/2e90a2ae46f1aa08a5af26f1bb0d21c3.png)
![a [\text {Bq/g}] = \frac{\lambda N}{M N/N_A} = \frac{\lambda N_A}{M}](../I/m/9c9bb1d2a4ef3955c79cfb8db510d35b.png)
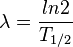
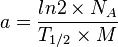
![a [\text {Bq/g}] \simeq \frac{4.17\times 10^{23} [\text{mol}^{-1}] }{T_{1/2} [s]\times M [\text {g } \text{mol}^{-1}]}](../I/m/c25fc4b9ab3c6e81e4c3754d3058761a.png)
![a [\text {Bq/g}] = \frac{ln2 \times {N_A}}{T_{1/2} [s] \times {M [\text {g } \text{mol}^{-1}]}} = \frac{ln2 \times {N_A}}{T_{1/2}[year] \times365\times24\times60\times60 \times M [\text {g } \text{mol}^{-1}]} \simeq \frac{1.32\times 10^{16} [\text{mol}^{-1}] }{T_{1/2}[year] \times M [\text {g } \text{mol}^{-1}]}](../I/m/5b8c1d42d57787dd21382a52d2eade7d.png)
![a_{Ra}[\text {Bq/g}] = \frac{1.32\times 10^{16} [\text{mol}^{-1}] }{1600[year] \times 226 [\text {g } \text{mol}^{-1}] } \simeq {3.7} \times 10^{10} [\text {Bq/g}]](../I/m/93b2e7189030db79277171f841f09de7.png)
![a_{Th}[\text {Bq/g}] = \frac{1.32\times 10^{16} [\text{mol}^{-1}] }{1.405\times 10^{10}[year] \times 232 [\text {g } \text{mol}^{-1}] } \simeq {4.059} \times 10^{3} [\text {Bq/g}]](../I/m/69415342e950ae81d9dc259a5341981b.png)
![a_{K}[\text {Bq/g}] = \frac{1.32\times 10^{16} [\text{mol}^{-1}] }{1.251\times 10^{9}[year] \times 40 [\text {g } \text{mol}^{-1}] } \simeq {2.63789} \times 10^{5} [\text {Bq/g}]](../I/m/0cf849485059544ec4b076c2974ac0f1.png)