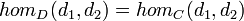Skeleton (category theory)
In mathematics, a skeleton of a category is a subcategory which, roughly speaking, does not contain any extraneous isomorphisms. In a certain sense, the skeleton of a category is the "smallest" equivalent category which captures all "categorical properties". In fact, two categories are equivalent if and only if they have isomorphic skeletons. A category is called skeletal if isomorphic objects are necessarily identical.
Definition
A skeleton of a category C is a full, isomorphism-dense subcategory D in which no two distinct objects are isomorphic. In detail, a skeleton of C is a category D such that:
- Every object of D is an object of C.
- (Fullness) For every pair of objects d1 and d2 of D, the morphisms in D are precisely the morphisms in C, i.e.
- For every object d of D, the D-identity on d is the C-identity on d.
- The composition law in D is the restriction of the composition law in C to the morphisms in D.
- (Isomorphism-dense) Every C-object is isomorphic to some D-object.
- No two distinct D-objects are isomorphic.
Existence and uniqueness
It is a basic fact that every small category has a skeleton; more generally, every accessible category has a skeleton. (This is equivalent to the axiom of choice.) Also, although a category may have many distinct skeletons, any two skeletons are isomorphic as categories, so up to isomorphism of categories, the skeleton of a category is unique.
The importance of skeletons comes from the fact that they are (up to isomorphism of categories), canonical representatives of the equivalence classes of categories under the equivalence relation of equivalence of categories. This follows from the fact that any skeleton of a category C is equivalent to C, and that two categories are equivalent if and only if they have isomorphic skeletons.
Examples
- The category Set of all sets has the subcategory of all cardinal numbers as a skeleton.
- The category K-Vect of all vector spaces over a fixed field
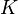 has the subcategory consisting of all powers
has the subcategory consisting of all powers  , where n is any cardinal number, as a skeleton; the maps
, where n is any cardinal number, as a skeleton; the maps  are exactly the n×m matrices with entries in K.
are exactly the n×m matrices with entries in K. - FinSet, the category of all finite sets has FinOrd, the category of all finite ordinal numbers, as a skeleton.
- The category of all well-ordered sets has the subcategory of all ordinal numbers as a skeleton.
- A preorder, i.e. a small category such that for every pair of objects
 , the set
, the set  either has one element or is empty, has a partially ordered set as a skeleton.
either has one element or is empty, has a partially ordered set as a skeleton.
See also
References
- Adámek, Jiří, Herrlich, Horst, & Strecker, George E. (1990). Abstract and Concrete Categories. Originally publ. John Wiley & Sons. ISBN 0-471-60922-6. (now free on-line edition)
- Robert Goldblatt (1984). Topoi, the Categorial Analysis of Logic (Studies in logic and the foundations of mathematics, 98). North-Holland. Reprinted 2006 by Dover Publications.