Shannon–Weaver model

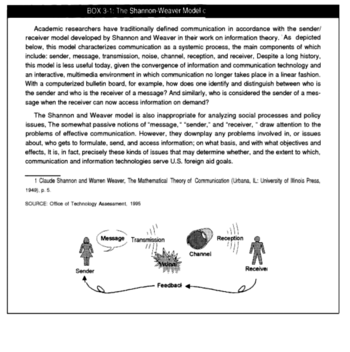
The Shannon–Weaver model as portrayed in a report from the United States Office of Technology Assessment^[1]

The Shannon–Weaver model of communication has been called the "mother of all models."^[2] Social Scientists use the term to refer to an integrated model of the concepts of information source, message, transmitter, signal, channel, noise, receiver, information destination, probability of error, encoding, decoding, information rate, channel capacity, etc. However, this is misleading, as the related fundamental ideas were developed by Shannon alone.^[3]

In 1948 Claude Elwood Shannon published A Mathematical Theory of Communication article in two parts in the July and October numbers of the Bell System Technical Journal.^[4] In this fundamental work he used tools in probability theory, developed by Norbert Wiener, which were in their nascent stages of being applied to communication theory at that time. Shannon developed information entropy as a measure for the uncertainty in a message while essentially inventing what became known as the dominant form of information theory.

The book co-authored with Warren Weaver, The Mathematical Theory of Communication, reprints Shannon's 1948 article and Weaver's popularization of it, which is accessible to the non-specialist.^[5] In short, Weaver reprinted Shannon's two-part paper, wrote a 28 page introduction for a 144 pages book, and changed the title from "A mathematical theory..." to "The mathematical theory...". Shannon's concepts were also popularized, subject to his own proofreading, in John Robinson Pierce's Symbols, Signals, and Noise, a popular introduction for non-specialists.^[6]

The term Shannon–Weaver model was widely adopted in social science fields such as education, communication sciences, organizational analysis, psychology, etc. At the same time, it has been subject to much criticism in the social sciences, as it is supposedly "inappropriate to represent social processes" ^[1] and "misleading misrepresentation of the nature of human communication", citing its simplicity and inability to consider context.^[7] In engineering, mathematics, physics, and biology Shannon's theory is used more literally and is referred to as Shannon theory, or information theory.^[8] This means that everywhere outside of the social sciences, there is no Shannon–Weaver model, but simply Shannon's information theory and that it is a misinterpretation to attribute the information theoretic channel logic to Weaver as well.^[3]

References