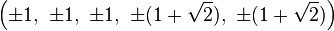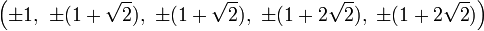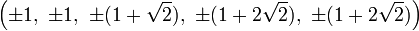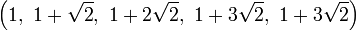Runcinated 5-cubes

Orthogonal projections in B₅ Coxeter plane
5-cube	Runcinated 5-cube	Runcinated 5-orthoplex
Runcitruncated 5-cube	Runcicantellated 5-cube	Runcicantitruncated 5-cube
Runcitruncated 5-orthoplex	Runcicantellated 5-orthoplex	Runcicantitruncated 5-orthoplex

In five-dimensional geometry, a runcinated 5-cube is a convex uniform 5-polytope that is a runcination (a 3rd order truncation) of the regular 5-cube.

There are unique 8 degrees of runcinations of the 5-cube, along with permutations of truncations, and cantellations. Four are more simply constructed relative to the 5-orthoplex.

Runcinated 5-cube

Runcinated 5-cube
Type	Uniform 5-polytope
Schläfli symbol	t_0,3{4,3,3,3}
Coxeter diagram
4-faces	202
Cells	1240
Faces	2160
Edges	1440
Vertices	320
Vertex figure	3-3 duoprism
Coxeter group	B₅ [4,3,3,3]
Properties	convex

Alternate names

Small prismated penteract (Acronym: span) (Jonathan Bowers)

Coordinates

The Cartesian coordinates of the vertices of a runcinated 5-cube having edge length 2 are all permutations of:

\left(\pm1,\ \pm1,\ \pm1,\ \pm(1+\sqrt{2}),\ \pm(1+\sqrt{2})\right)

Images

orthographic projections
Coxeter plane	B₅	B₄ / D₅	B₃ / D₄ / A₂
Graph
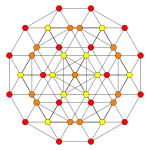Dihedral symmetry	[10]	[8]	[6]
Coxeter plane	B₂	A₃
Graph
Dihedral symmetry	[4]	[4]

Runcitruncated 5-cube

Runcitruncated 5-cube
Type	uniform polyteron
Schläfli symbol	t_0,1,3{4,3,3,3}
Coxeter-Dynkin diagrams
4-faces	202
Cells	1560
Faces	3760
Edges	3360
Vertices	960
Vertex figure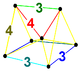
Coxeter groups	B₅, [3,3,3,4]
Properties	convex

Alternate names

Runcitruncated penteract
Prismatotruncated penteract (Acronym: pattin) (Jonathan Bowers)

Construction and coordinates

The Cartesian coordinates of the vertices of a runcitruncated 5-cube having edge length 2 are all permutations of:

\left(\pm1,\ \pm(1+\sqrt{2}),\ \pm(1+\sqrt{2}),\ \pm(1+2\sqrt{2}),\ \pm(1+2\sqrt{2})\right)

Images

orthographic projections
Coxeter plane	B₅	B₄ / D₅	B₃ / D₄ / A₂
Graph
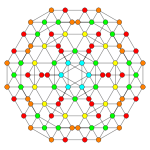Dihedral symmetry	[10]	[8]	[6]
Coxeter plane	B₂	A₃
Graph
Dihedral symmetry	[4]	[4]

Runcicantellated 5-cube

Runcicantellated 5-cube
Type	Uniform 5-polytope
Schläfli symbol	t_0,2,3{4,3,3,3}
Coxeter-Dynkin diagram
4-faces	202
Cells	1240
Faces	2960
Edges	2880
Vertices	960
Vertex figure
Coxeter group	B₅ [4,3,3,3]
Properties	convex

Alternate names

Runcicantellated penteract
Prismatorhombated penteract (Acronym: prin) (Jonathan Bowers)

Coordinates

The Cartesian coordinates of the vertices of a runcicantellated 5-cube having edge length 2 are all permutations of:

\left(\pm1,\ \pm1,\ \pm(1+\sqrt{2}),\ \pm(1+2\sqrt{2}),\ \pm(1+2\sqrt{2})\right)

Images

orthographic projections
Coxeter plane	B₅	B₄ / D₅	B₃ / D₄ / A₂
Graph
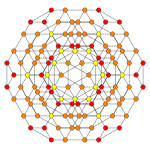Dihedral symmetry	[10]	[8]	[6]
Coxeter plane	B₂	A₃
Graph
Dihedral symmetry	[4]	[4]

Runcicantitruncated 5-cube

Runcicantitruncated 5-cube
Type	Uniform 5-polytope
Schläfli symbol	t_0,1,2,3{4,3,3,3}
Coxeter-Dynkin diagram
4-faces	202
Cells	1560
Faces	4240
Edges	4800
Vertices	1920
Vertex figure	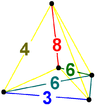Irregular 5-cell
Coxeter group	B₅ [4,3,3,3]
Properties	convex, isogonal

Alternate names

Runcicantitruncated penteract
Biruncicantitruncated 16-cell / Biruncicantitruncated pentacross
great prismated penteract (gippin) (Jonathan Bowers)

Coordinates

The Cartesian coordinates of the vertices of a runcicantitruncated 5-cube having an edge length of 2 are given by all permutations of coordinates and sign of:

\left(1,\ 1+\sqrt{2},\ 1+2\sqrt{2},\ 1+3\sqrt{2},\ 1+3\sqrt{2}\right)

Images

orthographic projections
Coxeter plane	B₅	B₄ / D₅	B₃ / D₄ / A₂
Graph
Dihedral symmetry	[10]	[8]	[6]
Coxeter plane	B₂	A₃
Graph
Dihedral symmetry	[4]	[4]

Related polytopes

These polytopes are a part of a set of 31 uniform polytera generated from the regular 5-cube or 5-orthoplex.

β₅	t₁β₅	t₂γ₅	t₁γ₅	γ₅	t_0,1β₅	t_0,2β₅	t_1,2β₅
t_0,3β₅	t_1,3γ₅	t_1,2γ₅	t_0,4γ₅	t_0,3γ₅	t_0,2γ₅	t_0,1γ₅	t_0,1,2β₅
t_0,1,3β₅	t_0,2,3β₅	t_1,2,3γ₅	t_0,1,4β₅	t_0,2,4γ₅	t_0,2,3γ₅	t_0,1,4γ₅	t_0,1,3γ₅
t_0,1,2γ₅	t_0,1,2,3β₅	t_0,1,2,4β₅	t_0,1,3,4γ₅	t_0,1,2,4γ₅	t_0,1,2,3γ₅	t_0,1,2,3,4γ₅

References

H.S.M. Coxeter:
- H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6
  - (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10]
  - (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591]
  - (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
Norman Johnson Uniform Polytopes, Manuscript (1991)
- N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D.
Richard Klitzing, 5D, uniform polytopes (polytera) o3x3o3o4x - span, o3x3o3x4x - pattin, o3x3x3o4x - prin, o3x3x3x4x - gippin

External links

Glossary for hyperspace, George Olshevsky.
Polytopes of Various Dimensions, Jonathan Bowers
- Runcinated uniform polytera (spid), Jonathan Bowers
Multi-dimensional Glossary

Fundamental convex regular and uniform polytopes in dimensions 2–10
Family	A_n	B_n	I₂(p) / D_n	E₆ / E₇ / E₈ / F₄ / G₂	H_n
Regular polygon	Triangle	Square	p-gon	Hexagon	Pentagon
Uniform polyhedron	Tetrahedron	Octahedron • Cube	Demicube		Dodecahedron • Icosahedron
Uniform 4-polytope	5-cell	16-cell • Tesseract	Demitesseract	24-cell	120-cell • 600-cell
Uniform 5-polytope	5-simplex	5-orthoplex • 5-cube	5-demicube
Uniform 6-polytope	6-simplex	6-orthoplex • 6-cube	6-demicube	1₂₂ • 2₂₁
Uniform 7-polytope	7-simplex	7-orthoplex • 7-cube	7-demicube	1₃₂ • 2₃₁ • 3₂₁
Uniform 8-polytope	8-simplex	8-orthoplex • 8-cube	8-demicube	1₄₂ • 2₄₁ • 4₂₁
Uniform 9-polytope	9-simplex	9-orthoplex • 9-cube	9-demicube
Uniform 10-polytope	10-simplex	10-orthoplex • 10-cube	10-demicube
Uniform n-polytope	n-simplex	n-orthoplex • n-cube	n-demicube	1_k2 • 2_k1 • k₂₁	n-pentagonal polytope
Topics: Polytope families • Regular polytope • List of regular polytopes and compounds

This article is issued from Wikipedia - version of the Thursday, April 30, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.