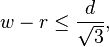Reuleaux triangle

The Reuleaux triangle is a constant width curve based on an equilateral triangle. All points on a side are equidistant from the opposite vertex.
Reuleaux triangle shaped water valve cover in San Francisco

A Reuleaux triangle [ʁœlo] is a shape formed from the intersection of three circular disks, each having its center on the boundary of the other two. It is a curve of constant width, the simplest and best known such curve other than the circle itself.[1] Constant width means that the separation of every two parallel supporting lines is the same, independent of their orientation. Because all its diameters are the same, the Reuleaux triangle is one answer to the question "Other than a circle, what shape can a manhole cover be made so that it cannot fall down through the hole?"[2]

Reuleaux triangles have also been called spherical triangles, but that term more properly refers to triangles on the curved surface of a sphere. They are named after Franz Reuleaux,[3] a 19th-century German engineer who pioneered the study of machines for translating one type of motion into another, and who used Reuleaux triangles in his designs.[4] However, these shapes were known before his time, for instance by the designers of Gothic church windows, by Leonardo da Vinci, who used it for a map projection, and by Leonhard Euler in his study of constant-width shapes. Other applications of the Reuleaux triangle include giving the shape to guitar picks, pencils, and drill bits for drilling square holes, as well as in graphic design in the shapes of some signs and corporate logos.

Among constant-width shapes with a given width, the Reuleaux triangle has the minimum area and the sharpest possible angle (120°) at its corners. By several numerical measures it is the farthest from being centrally symmetric. It provides the largest constant-width shape avoiding the points of an integer lattice, and is closely related to the shape of the quadrilateral maximizing the ratio of perimeter to diameter. It can perform a complete rotation within a square while at all times touching all four sides of the square, and has the smallest possible area of shapes with this property. However, although it covers most of the square in this rotation process, it fails to cover a small fraction of the square's area, near its corners. Because of this property of rotating within a square, the Reuleaux triangle is also sometimes known as the Reuleaux rotor.[5]

The Reuleaux triangle is the first of a sequence of Reuleaux polygons, curves of constant width formed from regular polygons with an odd number of sides. Some of these curves have been used as the shapes of coins. The Reuleaux triangle can also be generalized into three dimensions in multiple ways: the Reuleaux tetrahedron (the intersection of four spheres whose centers lie on a regular tetrahedron) does not have constant width, but can be modified by rounding its edges to form the Meissner tetrahedron, which does. Alternatively, the surface of revolution of the Reuleaux triangle also has constant width.

Construction

To construct a Reuleaux triangle

The Reuleaux triangle may be constructed either directly from three circles, or by rounding the sides of an equilateral triangle.[6]

The three-circle construction may be performed with a compass alone, not even needing a straightedge. By the Mohr–Mascheroni theorem the same is true more generally of any compass-and-straightedge construction,[7] but the construction for the Reuleaux triangle is particularly simple. The first step is to mark two arbitrary points of the plane (which will eventually become vertices of the triangle), and use the compass to draw a circle centered at one of the marked points, through the other marked point. Next, one draws a second circle, of the same radius, centered at the other marked point and passing through the first marked point. Finally, one draws a third circle, again of the same radius, with its center at one of the two crossing points of the two previous circles, passing through both marked points.[8] The central region in the resulting arrangement of three circles will be a Reuleaux triangle.[6]

Alternatively, a Reuleaux triangle may be constructed from an equilateral triangle T by drawing three arcs of circles, each centered at one vertex of T and connecting the other two vertices.[9] Or, equivalently, it may be constructed as the intersection of three disks centered at the vertices of T, with radius equal to the side length of T.[10]

Mathematical properties

Parallel supporting lines of a Reuleaux triangle

The most basic property of the Reuleaux triangle is that it has constant width, meaning that for every pair of parallel supporting lines (two lines of the same slope that both touch the shape without crossing through it) the two lines have the same Euclidean distance from each other, regardless of the orientation of these lines.[9] In any pair of parallel supporting lines, one of the two lines will necessarily touch the triangle at one of its vertices. The other supporting line may touch the triangle at any point on the opposite arc, and their distance (the width of the Reuleaux triangle) equals the radius of this arc.[11]

The first mathematician to discover the existence of curves of constant width, and to observe that the Reuleaux triangle has constant width, may have been Leonhard Euler.[5] In a paper that he presented in 1771 and published in 1781 entitled De curvis triangularibus, Euler studied curvilinear triangles as well as the curves of constant width, which he called orbiforms.[12][13]

Extremal measures

By many different measures, the Reuleaux triangle is one of the most extreme curves of constant width.

By the Blaschke–Lebesgue theorem, the Reuleaux triangle has the smallest possible area of any curve of given constant width. This area is

\frac{1}{2}(\pi - \sqrt3)s^2 \approx 0.70477s^2,

where s is the constant width. One method for deriving this area formula is to partition the Reuleaux triangle into an inner equilateral triangle and three curvilinear regions between this inner triangle and the arcs forming the Reuleaux triangle, and then add the areas of these four sets. At the other extreme, the curve of constant width that has the maximum possible area is a circular disk, which has area \pi s^2 / 4\approx 0.78540s^2.[14]

The angles made by each pair of arcs at the corners of a Reuleaux triangle are all equal to 120°. This is the sharpest possible angle at any vertex of any curve of constant width.[9] Additionally, among the curves of constant width, the Reuleaux triangle is the one with both the largest and the smallest inscribed equilateral triangles.[15] The largest equilateral triangle inscribed in a Reuleaux triangle is the one connecting its three corners, and the smallest one is the one connecting the three midpoints of its sides. The subset of the Reuleaux triangle consisting of points belonging to three or more diameters is the interior of the larger of these two triangles; it has a larger area than the set of three-diameter points of any other curve of constant width.[16]

Centrally symmetric shapes inside and outside a Reuleaux triangle, used to measure its asymmetry

Although the Reuleaux triangle has sixfold dihedral symmetry, the same as an equilateral triangle, it does not have central symmetry. The Reuleaux triangle is the least symmetric curve of constant width according to two different measures of central asymmetry, the Kovner–Besicovitch measure (ratio of area to the largest centrally symmetric shape enclosed by the curve) and the Estermann measure (ratio of area to the smallest centrally symmetric shape enclosing the curve). For the Reuleaux triangle, the two centrally symmetric shapes that determine the measures of asymmetry are both hexagonal, although the inner one has curved sides.[17] The Reuleaux triangle has diameters that split its area more unevenly than any other curve of constant width. That is, the maximum ratio of areas on either side of a diameter, another measure of asymmetry, is bigger for the Reuleaux triangle than for other curves of constant width.[18]

Among all shapes of constant width that avoid all points of an integer lattice, the one with the largest width is a Reuleaux triangle. It has one of its axes of symmetry parallel to the coordinate axes on a half-integer line. Its width, approximately 1.545, is the root of a degree-6 polynomial with integer coefficients.[17][19][20]

Just as it is possible for a circle to be surrounded by six congruent circles that touch it, it is also possible to arrange seven congruent Reuleaux triangles so that they all make contact with a central Reuleaux triangle of the same size. This is the maximum number possible for any curve of constant width.[21]

An equidiagonal kite that maximizes the ratio of perimeter to diameter, inscribed in a Reuleaux triangle

Among all quadrilaterals, the shape that has the greatest ratio of its perimeter to its diameter is an equidiagonal kite that can be inscribed into a Reuleaux triangle.[22]

Other measures

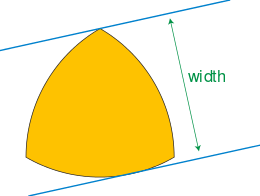
By Barbier's theorem all curves of constant width including the Reuleaux triangle have equal perimeter. In particular this perimeter equals the perimeter of the circle with the same width, which is \pi s.[23][24][9]

The radii of the largest inscribed circle of a Reuleaux triangle with width s, and of the circumscribed circle of the same triangle, are

\displaystyle\left(1-\frac{1}{\sqrt 3}\right)s\approx 0.42265s and \displaystyle\frac{s}{\sqrt 3}\approx 0.57735s

respectively; the sum of these radii equals the width of the Reuleaux triangle. More generally, for every curve of constant width, the largest inscribed circle and the smallest circumscribed circle are concentric, and their radii sum to the constant width of the curve.[25]

Unsolved problem in mathematics:
How densely can Reuleaux triangles be packed in the plane?
(more unsolved problems in mathematics)

The optimal packing density of the Reuleaux triangle in the plane remains unproven, but is conjectured to be

\frac{2(\pi-\sqrt 3)}{\sqrt{15}+\sqrt{7}-\sqrt{12}} \approx 0.922888,

which is the density of one possible double lattice packing for these shapes. The best proven upper bound on the packing density is approximately 0.947275.[26] It has also been conjectured, but not proven, that the Reuleaux triangles have the highest packing density of any curve of constant width.[27]

Rotation within a square

Rotation of a Reuleaux triangle within a square, showing also the curve traced by the center of the triangle

Any curve of constant width can form a rotor within a square, a shape that can perform a complete rotation while staying within the square and at all times touching all four sides of the square. However, the Reuleaux triangle is the rotor with the minimum possible area.[9] As it rotates, its axis does not stay fixed at a single point, but instead follows a curve formed by the pieces of four ellipses.[28] Because of its 120° angles, the rotating Reuleaux triangle cannot reach some points near the sharper angles at the square's vertices, but rather covers a shape with slightly rounded corners, also formed by elliptical arcs.[9]

Reuleaux triangle in a square, with ellipse governing the path of motion of the triangle center
Ellipse followed by the center of a rotating Reuleaux triangle in a square
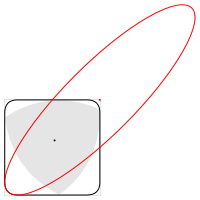
Reuleaux triangle in a square, with ellipse bounding the region swept by the triangle
Ellipse separating the corners of a square from the region swept by a rotating Reuleaux triangle

At any point during this rotation, two of the corners of the Reuleaux triangle touch two adjacent sides of the square, while the third corner of the triangle traces out a curve near the opposite vertex of the square. The shape traced out by the rotating Reuleaux triangle covers approximately 98.77% of the area of the square.[29]

As a counterexample

Reuleaux's original motivation for studying the Reuleaux triangle was as a counterexample, showing that three single-point contacts may not be enough to fix a planar object into a single position.[30]

The existence of Reuleaux polygons shows that diameter measurements alone cannot verify that an object has a circular cross-section.[31] Overlooking this fact may have played a role in the Space Shuttle Challenger disaster, as the roundness of sections of the rocket in that launch was tested only by measuring different diameters, and off-round shapes may cause unusually high stresses that could have been one of the factors causing the disaster.[9]

In connection with the inscribed square problem, Eggleston (1958) observed that the Reuleaux triangle provides an example of a constant-width shape in which no regular polygon with more than four sides can be inscribed, except the regular hexagon, and he described a small modification to this shape that preserves its constant width but also prevents regular hexagons from being inscribed in it. He generalized this result to three dimensions using a cylinder with the same shape as its cross section.[32]

Applications

Reaching into corners

Several types of machinery take the shape of the Reuleaux triangle, based on its property of being able to rotate within a square.

The Watts Brothers Tool Works square drill bit has the shape of a Reuleaux triangle, modified with concavities to form cutting surfaces. When mounted in a special chuck which allows for the bit not having a fixed centre of rotation, it can drill a hole that is nearly square.[33] Although patented by Henry Watts in 1914, similar drills invented by others were used earlier.[9] Other Reuleaux polygons are used to drill pentagonal, hexagonal, and octagonal holes.[9][33]

Panasonic's RULO robotic vacuum cleaner has its shape based on the Reuleaux triangle in order to ease cleaning up dust in the corners of rooms.[34][35]

Rolling cylinders

Comparison of a cylindrical and Reuleaux triangle roller

Another class of applications of the Reuleaux triangle involves cylindrical objects with a Reuleaux triangle cross section. Several pencils are manufactured in this shape, rather than the more traditional round or hexagonal barrels.[36] They are usually promoted as being more comfortable or encouraging proper grip, as well as being less likely to roll off tables (since the center of gravity moves up and down more than a rolling hexagon).

A Reuleaux triangle (along with all other curves of constant width) can roll but makes a poor wheel because it does not roll about a fixed center of rotation. An object on top of rollers that have Reuleaux triangle cross-sections would roll smoothly and flatly, but an axle attached to Reuleaux triangle wheels would bounce up and down three times per revolution.[9][37] This concept was used in a science fiction short story by Poul Anderson titled "Three-Cornered Wheel".[11] A bicycle with floating axles and a frame supported by the rim of its Reuleaux triangle shaped wheel was built and demonstrated in 2009 by Chinese inventor Guan Baihua, who was inspired by pencils with the same shape.[38]

Mechanism design

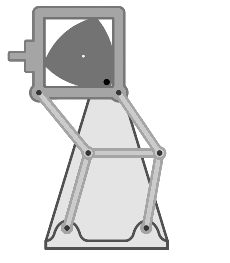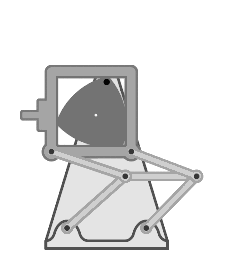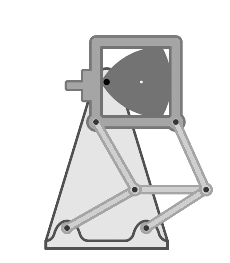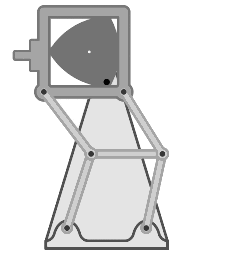
Reuleaux triangle based film advance mechanism in the Soviet Luch-2 8 mm film projector

Another class of applications of the Reuleaux triangle involves using it as a part of a mechanical linkage that can convert rotation around a fixed axis into reciprocating motion.[10] These mechanisms were studied by Franz Reuleaux. With the assistance of the Gustav Voigt company, Reuleaux built approximately 800 models of mechanisms, several of which involved the Reuleaux triangle.[39] Reuleaux used these models in his pioneering scientific investigations of their motion.[40] Although most of the Reuleaux–Voigt models have been lost, 219 of them have been collected at Cornell University, including nine based on the Reuleaux triangle.[39][41] However, the use of Reuleaux triangles in mechanism design predates the work of Reuleaux; for instance, some steam engines from as early as 1830 had a cam in the shape of a Reuleaux triangle.[42][43]

One application of this principle arises in a film projector. In this application, it is necessary to advance the film in a jerky, stepwise motion, in which each frame of film stops for a fraction of a second in front of the projector lens, and then much more quickly the film is moved to the next frame. This can be done using a mechanism in which the rotation of a Reuleaux triangle within a square is used to create a motion pattern for an actuator that pulls the film quickly to each new frame and then pauses the film's motion while the frame is projected.[44]

The rotor of the Wankel engine is shaped as a curvilinear triangle that is often cited as an example of a Reuleaux triangle.[9][43] However, its curved sides are somewhat flatter than those of a Reuleaux triangle and so it does not have constant width.[45]

Architecture

Reuleaux triangle shaped window of the Church of Our Lady, Bruges in Belgium
The Kölntriangle building has a Reuleaux triangle cross-section

In Gothic architecture, beginning in the late 13th century or early 14th century,[46] the Reuleaux triangle became one of several curvilinear forms frequently used for windows, window tracery, and other architectural decorations.[3] For instance, in English Gothic architecture, this shape was associated with the decorated period, both in its geometric style of 1250–1290 and continuing into its curvilinear style of 1290–1350.[46] In this context, the shape is more frequently called a spherical triangle,[46][47][48] but the more usual mathematical meaning of a spherical triangle is a triangle on the surface of a sphere (a shape also commonly used in architecture as a pendentive). In its use in Gothic church architecture, the three-cornered shape of the Reuleaux triangle may be seen both as a symbol of the Trinity,[49] and as "an act of opposition to the form of the circle".[50]

The Reuleaux triangle has also been used in other styles of architecture. For instance, Leonardo da Vinci sketched this shape as the plan for a fortification.[41] A modern high-rise building, the Kölntriangle in Cologne, Germany, was built with a Reuleaux triangle cross-section. Together with the circular shape of its core, this gives varied depths to the rooms of the building.[51]

Mapmaking

Another early application of the Reuleaux triangle, by Leonardo da Vinci circa 1514 (or possibly by one of his followers at his direction), was a world map in which the spherical surface of the earth was divided into eight octants, each flattened into the shape of a Reuleaux triangle.[52][53][54]

Leonardo da Vinci's world map in eight Reuleaux-triangle quadrants

Similar maps also based on the Reuleaux triangle were published by Oronce Finé in 1551 and by John Dee in 1580.[54]

Other objects

Three Reuleaux triangle shaped guitar picks

Many guitar picks employ the Reuleaux triangle, as its shape combines a sharp point to provide strong articulation, with a wide tip to produce a warm timbre. Because all three points of the shape are usable, it is easier to orient and wears less quickly compared to a pick with a single tip.[55]

The Submillimeter Array, with seven of its eight antennae arranged on an approximate Reuleaux triangle

Following a suggestion of Keto (1997),[56] the antennae of the Submillimeter Array, a radio-wave astronomical observatory on Mauna Kea in Hawaii, are arranged on four nested Reuleaux triangles.[57][58] Placing the antennae on a curve of constant width causes the observatory to have the same spatial resolution in all directions, and provides a circular observation beam. As the most asymmetric curve of constant width, the Reuleaux triangle leads to the most uniform coverage of the plane for the Fourier transform of the signal from the array.[56][58] The antennae may be moved from one Reuleaux triangle to another for different observations, according to the desired angular resolution of each observation.[57][58] The precise placement of the antennae on these Reuleaux triangles was optimized using a neural network. In some places the constructed observatory departs from the preferred Reuleaux triangle shape because that shape was not possible within the given site.[58]

Signs and logos

The shield shapes used for many signs and corporate logos feature rounded triangles, some of which are more specifically Reuleaux triangles.

The corporate logo of Petrofina (Fina), a Belgian oil company with major operations in Europe, North America and Africa, used a Reuleaux triangle with the Fina name from 1950 until Petrofina's merger with Total S.A. in 2000.[59][60] Another corporate logo framed in the Reuleaux triangle, the south-pointing compass of Bavaria Brewery, was part of a makeover by design company Total Identity that won the SAN 2010 Advertiser of the Year award.[61] The Reuleaux triangle is also used in the logo of Colorado School of Mines.[62]

In nature

The Reuleaux triangle as the central bubble in a mathematical model of a four-bubble planar soap bubble cluster

According to Plateau's laws, the circular arcs in two-dimensional soap bubble clusters meet at 120° angles, the same angle found at the corners of a Reuleaux triangle. Based on this fact, it is possible to construct clusters in which some of the bubbles take the form of a Reuleaux triangle.[63]

The shape was first isolated in crystal form in 2014 as Reuleaux triangle disks.[64] Basic bismuth nitrate disks with the Reuleaux triangle shape were formed from the hydrolysis and precipitation of bismuth nitrate in an ethanol–water system in the presence of 2,3-bis(2-pyridyl)pyrazine.

Generalizations

Triangular curves of constant width with smooth rather than sharp corners may be obtained as the locus of points at a fixed distance from the Reuleaux triangle.[65] Other generalizations of the Reuleaux triangle include surfaces in three dimensions, curves of constant width with more than three sides, and the Yanmouti sets which provide extreme examples of an inequality between width, diameter, and inradius.

Three-dimensional version

Four spheres intersect to form a Reuleaux tetrahedron.

The intersection of four spheres of radius s centered at the vertices of a regular tetrahedron with side length s is called the Reuleaux tetrahedron, but is not a surface of constant width.[66] It can, however, be made into a surface of constant width, called Meissner's tetrahedron, by replacing its edge arcs by curved surfaces, the surfaces of rotation of a circular arc. Alternatively, the surface of revolution of a Reuleaux triangle through one of its symmetry axes forms a surface of constant width, with minimum volume among all known surfaces of revolution of given constant width.[67]

Reuleaux polygons

Reuleaux polygons

The Reuleaux triangle can be generalized to regular polygons with an odd number of sides, yielding a Reuleaux polygon. These are the only shapes of constant width whose boundaries are formed by finitely many circular arcs of equal length.[68]

The constant width of these shapes allows their use as coins that can be used in coin-operated machines. For instance, the United Kingdom has made 20-pence and 50-pence coins in the shape of a Reuleaux heptagon.[9] The Canadian Loonie dollar coin uses another Reuleaux polygon with 11 sides.[69]

Similar methods can be used to enclose an arbitrary simple polygon within a curve of constant width, whose width equals the diameter of the given polygon. The resulting shape consists of circular arcs (at most as many as sides of the polygon), can be constructed algorithmically in linear time, and can be drawn with compass and straightedge.[70] Although the regular-polygon based Reuleaux polygons all have an odd number of circular-arc sides, it is possible to construct constant-width shapes based on irregular polygons that have an even number of sides.[71]

Yanmouti sets

The Yanmouti sets are defined as the convex hulls of an equilateral triangle together with three circular arcs, centered at the triangle vertices and spanning the same angle as the triangle, with equal radii that are at most equal to the side length of the triangle. Thus, when the radius is small enough, these sets degenerate to the equilateral triangle itself, but when the radius is as large as possible they equal the corresponding Reuleaux triangle. Every shape with width w, diameter d, and inradius r (the radius of the largest possible circle contained in the shape) obeys the inequality

w - r \le \frac{d}{\sqrt 3},

and this inequality becomes an equality for the Yanmouti sets, showing that it cannot be improved.[72]

Related figures

Triquetra interlaced to form a trefoil knot

In the classical presentation of a three-set Venn diagram as three overlapping circles, the central region (representing elements belonging to all three sets) takes the shape of a Reuleaux triangle.[3] The same three circles form one of the standard drawings of the Borromean rings, three mutually linked rings that cannot, however, be realized as geometric circles.[73] Parts of these same circles are used to form the triquetra, a figure of three overlapping semicircles (each two of which form a vesica piscis symbol) that again has a Reuleaux triangle at its center;[74] just as the three circles of the Venn diagram may be interlaced to form the Borromean rings, the three circular arcs of the triquetra may be interlaced to form a trefoil knot.[75]

Relatives of the Reuleaux triangle arise in the problem of finding the minimum perimeter shape that encloses a fixed amount of area and includes three specified points in the plane. For a wide range of choices of the area parameter, the optimal solution to this problem will be a curved triangle whose three sides are circular arcs with equal radii. In particular, when the three points are equidistant from each other and the area is that of the Reuleaux triangle, the Reuleaux triangle is the optimal enclosure.[76]

Circular triangles are triangles with circular-arc edges, including the Reuleaux triangle as well as other shapes. The Deltoid curve is another type of curvilinear triangle, but one in which the curves replacing each side of an equilateral triangle are concave rather than convex. It is not composed of circular arcs, but may be formed by rolling one circle within another of three times the radius.[77] Other planar shapes with three curved sides include the arbelos, which is formed from three semicircles with collinear endpoints,[78] and the Bézier triangle.[79]

The Reuleaux triangle may also be interpreted as the conformal image of a spherical triangle with 120° angles.[63] This spherical triangle is one of the Schwarz triangles (with parameters 3/2, 3/2, 3/2), triangles bounded by great-circle arcs on the surface of a sphere that can tile the sphere by reflection.[80]

References

  1. ↑ Gardner (2014) calls it the simplest, while Gruber (1983), p. 59 calls it "the most notorious".
  2. ↑ Klee, Victor (1971), "Shapes of the future", The Two-Year College Mathematics Journal 2 (2): 14–27, doi:10.2307/3026963.
  3. 1 2 3 Alsina, Claudi; Nelsen, Roger B. (2011), Icons of Mathematics: An Exploration of Twenty Key Images, Dolciani Mathematical Expositions 45, Mathematical Association of America, p. 155, ISBN 978-0-88385-352-8.
  4. ↑ Moon, F. C. (2007), The Machines of Leonardo Da Vinci and Franz Reuleaux: Kinematics of Machines from the Renaissance to the 20th Century, History of Mechanism and Machine Science 2, Springer, ISBN 978-1-4020-5598-0.
  5. 1 2 Bryant, John; Sangwin, Chris (2011), How Round Is Your Circle?: Where Engineering and Mathematics Meet, Princeton University Press, p. 190, ISBN 978-0-691-14992-9.
  6. 1 2 Hann, Michael (2014), Structure and Form in Design: Critical Ideas for Creative Practice, A&C Black, p. 34, ISBN 978-1-4725-8431-1.
  7. ↑ Hungerbühler, Norbert (1994), "A short elementary proof of the Mohr-Mascheroni theorem", American Mathematical Monthly 101 (8): 784–787, doi:10.2307/2974536, MR 1299166.
  8. ↑ This construction is briefly described by Maor & Jost (2014) and may be seen, for instance, in the video Fun with Reuleaux triangles by Alex Franke, August 21, 2011.
  9. 1 2 3 4 5 6 7 8 9 10 11 12 Gardner, Martin (2014), "Chapter 18: Curves of Constant Width", Knots and Borromean Rings, Rep-Tiles, and Eight Queens, The New Martin Gardner Mathematical Library 4, Cambridge University Press, pp. 223–245, ISBN 978-0-521-75613-6.
  10. 1 2 Klee, Victor; Wagon, S. (1991), Old and New Unsolved Problems in Plane Geometry and Number Theory, Dolciani mathematical expositions 11, Cambridge University Press, p. 21, ISBN 978-0-88385-315-3.
  11. 1 2 Maor, Eli; Jost, Eugen (2014), "46 The Reuleaux Triangle", Beautiful Geometry, Princeton University Press, pp. 154–156, ISBN 978-1-4008-4833-1.
  12. ↑ Reich, Karin (2007), "Euler's contribution to differential geometry and its reception", in Bradley, Robert E.; Sandifer, Ed, Leonhard Euler: Life, Work and Legacy, Studies in the History and Philosophy of Mathematics 5, Elsevier, pp. 479–502, doi:10.1016/S0928-2017(07)80026-0. See in particular section 1.4, "Orbiforms, 1781", pp. 484–485.
  13. ↑ Euler, Leonhard (1781), "De curvis triangularibus", Acta Academiae Scientarum Imperialis Petropolitinae (in Latin) 1778: 3–30. See in particular p. 7 for the definition of orbiforms.
  14. ↑ Gruber, Peter M. (1983), Convexity and its Applications, Birkhäuser, p. 67, ISBN 978-3-7643-1384-5
  15. ↑ Gruber (1983), p. 76
  16. ↑ Makeev, V. V. (2000), "An extremal property of the Reuleaux triangle", Zap. Nauchn. Sem. S.-Peterburg. Otdel. Mat. Inst. Steklov. (POMI) 267 (Geom. i Topol. 5): 152–155, 329, doi:10.1023/A:1021287302603, MR 1809823.
  17. 1 2 Finch, Steven R. (2003), "8.10 Reuleaux Triangle Constants" (PDF), Mathematical Constants, Encyclopedia of Mathematics and its Applications, Cambridge University Press, pp. 513–514, ISBN 978-0-521-81805-6.
  18. ↑ Groemer, H.; Wallen, L. J. (2001), "A measure of asymmetry for domains of constant width", Beiträge zur Algebra und Geometrie 42 (2): 517–521, MR 1865537.
  19. ↑ Gruber (1983), p. 78
  20. ↑ Sallee, G. T. (1969), "The maximal set of constant width in a lattice", Pacific Journal of Mathematics 28: 669–674, doi:10.2140/pjm.1969.28.669, MR 0240724.
  21. ↑ Fejes Tóth, L. (1967), "On the number of equal discs that can touch another of the same kind", Studia Scientiarum Mathematicarum Hungarica 2: 363–367, MR 0221388; Schopp, J. (1970), "Über die Newtonsche Zahl einer Scheibe konstanter Breite", Studia Scientiarum Mathematicarum Hungarica (in German) 5: 475–478, MR 0285983.
  22. ↑ Ball, D.G. (1973), "A generalisation of π", The Mathematical Gazette 57 (402): 298–303, doi:10.2307/3616052; Griffiths, David; Culpin, David (1975), "Pi-optimal polygons", The Mathematical Gazette 59 (409): 165–175, doi:10.2307/3617699.
  23. ↑ Lay, Steven R. (2007), Convex Sets and Their Applications, Dover, Theorem 11.11, pp. 81–82, ISBN 978-0-486-45803-8.
  24. ↑ Barbier, E. (1860), "Note sur le problème de l'aiguille et le jeu du joint couvert" (PDF), Journal de Mathématiques Pures et Appliquées, 2e série (in French) 5: 273–286. See in particular pp. 283–285.
  25. ↑ Lay (2007), Theorem 11.8, pp. 80–81.
  26. ↑ Blind, G.; Blind, R. (1983), "Eine Abschätzung für die Dichte der dichtesten Packung mit Reuleaux-Dreiecken", Studia Scientiarum Mathematicarum Hungarica (in German) 18 (2-4): 465–469, MR 787951. See also Blind, G.; Blind, R. (1987), "Reguläre Packungen mit Reuleaux-Dreiecken", Results in Mathematics (in German) 11 (1-2): 1–7, doi:10.1007/BF03323256, MR 880190.
  27. ↑ Resnikoff, Howard L. (2015), On Curves and Surfaces of Constant Width, arXiv:1504.06733.
  28. ↑ Gleiftner, Winfried; Zeitler, Herbert (May 2000), "The Reuleaux triangle and its center of mass", Results in Mathematics 37 (3-4): 335–344, doi:10.1007/bf03322004.
  29. ↑ Pickover, Clifford A. (2009), "Reuleaux Triangle", The Math Book: From Pythagoras to the 57th Dimension, 250 Milestones in the History of Mathematics, Sterling Publishing Company, p. 266, ISBN 978-1-4027-5796-9.
  30. ↑ Moon (2007), p. 239.
  31. ↑ Granovsky, V. A.; Siraya, T. N., "Metrological traceability and quality of industrial tests measurements", in Pavese, F.; Bär, M.; Filtz, J.-R.; Forbes, A. B.; Pendrill, L.; Shirono, K., Advanced Mathematical and Computational Tools in Metrology and Testing IX, World Scientific, pp. 194–201. See in particular p. 200.
  32. ↑ Eggleston, H. G. (1958), "Figures inscribed in convex sets", American Mathematical Monthly 65: 76–80, doi:10.2307/2308878, MR 0097768.
  33. 1 2 How to drill square hexagon octagon pentagon holes, Wilmerding, Pennsylvania: Watts Brothers Tool Works, 1950–1951 (27 page brochure).
  34. ↑ Mochizuki, Takashi (January 22, 2015), "Panasonic Rolls Out Triangular Robot Vacuum", Japan Real Time, Wall Street Journal.
  35. ↑ Coxworth, Ben (March 3, 2015), "Panasonic enters the robo-vac game, with the triangular Rulo", Gizmag.
  36. ↑ Gamber, Johnny (April 26, 2006), "Review of Staedtler Noris Ergosoft HB", Pencil Revolution, retrieved 2015-05-22.
  37. ↑ Masferrer León, Claudia; von Wuthenau Mayer, Sebastián (December 2005), "Reinventing the wheel: Non-circular wheels", The Mathematical Intelligencer 27 (4): 7–13, doi:10.1007/bf02985852.
  38. ↑ Dempster, Tyra (June 17, 2009), Chinese man reinvents the wheel, Reuters.
  39. 1 2 Moon, Francis C. (July 1999), The Reuleaux Collection of Kinematic Mechanisms at Cornell University, Cornell University Library.
  40. ↑ Sinclair, Nathalie; Higginson, William (2007), Mathematics and the Aesthetic: New Approaches to an Ancient Affinity, CMS Books in Mathematics, Springer, p. 81, ISBN 978-0-387-38145-9.
  41. 1 2 Moon (2007), p. 241.
  42. ↑ Moon (2007), p. 240
  43. 1 2 Peterson, Ivars (October 19, 1996), "Rolling with Reuleaux", MathTrek (ScienceNews). Reprinted in Peterson, Ivars (2002), Mathematical Treks: From Surreal Numbers to Magic Circles, MAA spectrum, Mathematical Association of America, pp. 141–144, ISBN 978-0-88385-537-9.
  44. ↑ Lay (2007), p. 83.
  45. ↑ Gruber (1983), p. 80. For a detailed calculation of the curvature of a circular arc approximating the optimal Wankel rotor shape, see Badr, O.; Naik, S.; O'Callaghan, P. W.; Probert, S. D. (1991), "Rotary Wankel engines as expansion devices in steam Rankine-cycle engines", Applied Energy 39 (1): 59–76, doi:10.1016/0306-2619(91)90063-4.
  46. 1 2 3 Hart, Stephen (2010), Medieval Church Window Tracery in England, Boydell & Brewer Ltd, pp. 63–64, ISBN 978-1-84383-533-2.
  47. ↑ Parker, John Henry (1850), A glossary of terms used in Grecian, Roman, Italian, and Gothic architecture 1 (5th ed.), London: David Rogue, p. 202.
  48. ↑ Burchett, E. S. (1876), Practical plane geometry, London and Glasgow: William Collins, Sons, and Co., Caption to Plate LV, Fig. 6.
  49. ↑ Durand, Guillaume (1906), The Symbolism of Churches and Church Ornaments: A Translation of the First Book of the Rationale Divinorum Officiorum (3rd ed.), Gibbings, p. lxxxviii.
  50. ↑ Frankl, Paul; Crossley, Paul (2000), Gothic Architecture, Pelican history of art 19, Yale University Press, p. 146, ISBN 978-0-300-08799-4.
  51. ↑ Architecture, Kölntriangle, retrieved 2015-05-23.
  52. ↑ Snyder, John P. (1997), Flattening the Earth: Two Thousand Years of Map Projections, University of Chicago Press, p. 40, ISBN 978-0-226-76747-5.
  53. ↑ Keuning, Johannes (January 1955), "The history of geographical map projections until 1600", Imago Mundi 12 (1): 1–24, doi:10.1080/03085695508592085, JSTOR 1150090.
  54. 1 2 Bower, David I. (February 2012), "The unusual projection for one of John Dee's maps of 1580" (PDF), The Cartographic Journal 49 (1): 55–61, doi:10.1179/1743277411y.0000000015.
  55. ↑ Hoover, Will (November 1995), Picks!: The Colorful Saga of Vintage Celluloid Guitar Plectrums, Backbeat Books, pp. 32–33, ISBN 978-0-87930-377-8.
  56. 1 2 Keto, Eric (1997), "The shapes of cross-correlation interferometers", The Astrophysical Journal 475 (2): 843–852, Bibcode:1997ApJ...475..843K, doi:10.1086/303545.
  57. 1 2 Blundell, Raymond (2007), "The submillimeter array" (PDF), Proc. 2007 IEEE/MTT-S International Microwave Symposium, doi:10.1109/mwsym.2007.380132.
  58. 1 2 3 4 Ho, Paul T. P.; Moran, James M.; Lo, Kwok Yung (2004), "The submillimeter array", The Astrophysical Journal 616 (1): L1–L6, Bibcode:2004ApJ...616L...1H, doi:10.1086/423245.
  59. ↑ Gwillian, Sam (May 16, 2015), Interesting Stuff: Curves of Constant Width, Newport City Radio.
  60. ↑ "Fina Logo History: from Petrofina to Fina", Total: Group Presentation (Total S.A.), archived from the original on December 26, 2012, retrieved 31 October 2015.
  61. ↑ Global: Bavaria, Fundamental Rebranding Operation at Bavaria, Total Identity, retrieved 2015-06-27.
  62. ↑ Fisher, Roland B. (Spring 2002), "M-blems: Explaining the logo" (PDF), Mines: the magazine of Colorado School of Mines 92 (2): 29.
  63. 1 2 Modes, Carl D.; Kamien, Randall D. (2013), "Spherical foams in flat space", Soft Matter (Royal Society of Chemistry) 9 (46): 11078–11084, arXiv:0810.5724, doi:10.1039/c3sm51585k.
  64. ↑ Ng, C. H. B.; Fan, W. Y. (2014), "Reuleaux triangle disks: New shape on the block", Journal of the American Chemical Society 136 (37): 12840–12843, doi:10.1021/ja506625y.
  65. ↑ Banchoff, Thomas; Giblin, Peter (1994), "On the geometry of piecewise circular curves", American Mathematical Monthly 101 (5): 403–416, doi:10.2307/2974900, MR 1272938.
  66. ↑ Weber, Christof (2009), What does this solid have to do with a ball? (PDF) Weber also has films of both types of Meissner body rotating.
  67. ↑ Campi, Stefano; Colesanti, Andrea; Gronchi, Paolo (1996), "Minimum problems for volumes of convex bodies", Partial Differential Equations and Applications: Collected Papers in Honor of Carlo Pucci, Lecture Notes in Pure and Applied Mathematics, no. 177, Marcel Dekker, pp. 43–55.
  68. ↑ Firey, W. J. (1960), "Isoperimetric ratios of Reuleaux polygons", Pacific Journal of Mathematics 10: 823–829, doi:10.2140/pjm.1960.10.823, MR 0113176.
  69. ↑ Chamberland, Marc (2015), Single Digits: In Praise of Small Numbers, Princeton University Press, pp. 104–105, ISBN 9781400865697.
  70. ↑ Chandru, V.; Venkataraman, R. (1991), "Circular hulls and orbiforms of simple polygons", Proceedings of the Second Annual ACM-SIAM Symposium on Discrete Algorithms (SODA '91), Philadelphia, PA, USA: Society for Industrial and Applied Mathematics, pp. 433–440, ISBN 0-89791-376-0.
  71. ↑ Peterson, Bruce B. (1973), "Intersection properties of curves of constant width", Illinois Journal of Mathematics 17: 411–420, MR 0320885.
  72. ↑ Hernández Cifre, M. A. (2000), "Is there a planar convex set with given width, diameter, and inradius?", American Mathematical Monthly 107 (10): 893–900, doi:10.2307/2695582, MR 1806918.
  73. ↑ Lindström, Bernt; Zetterström, Hans-Olov (1991), "Borromean circles are impossible", American Mathematical Monthly 98 (4): 340–341, doi:10.2307/2323803, JSTOR 2323803.
  74. ↑ Weisstein, Eric W., "Triquetra", MathWorld.
  75. ↑ Hoy, Jessica; Millett, Kenneth C. (2014), "A mathematical analysis of knotting and linking in Leonardo da Vinci's cartelle of the Accademia Vinciana" (PDF), Journal of Mathematics and the Arts.
  76. ↑ Courant, Richard; Robbins, Herbert (1996), What Is Mathematics? An Elementary Approach to Ideas and Methods (2nd ed.), Oxford University Press, pp. 378–379, ISBN 978-0-19-975487-8.
  77. ↑ Lockwood, E. H. (1961), "Chapter 8: The Deltoid", A Book of Curves, Cambridge University Press
  78. ↑ Mackay, J. S. (February 1884), "The shoemaker's knife", Proceedings of the Edinburgh Mathematical Society 3: 2, doi:10.1017/s0013091500037196.
  79. ↑ Bruijns, J. (1998), "Quadratic Bezier triangles as drawing primitives", Proceedings of the ACM SIGGRAPH/EUROGRAPHICS Workshop on Graphics Hardware (HWWS '98), New York, NY, USA: ACM, pp. 15–24, doi:10.1145/285305.285307, ISBN 1-58113-097-X.
  80. ↑ Wenninger, Magnus J. (2014), Spherical Models, Dover, p. 134, ISBN 978-0-486-14365-1.

External links

Wikimedia Commons has media related to Reuleaux triangles.
This article is issued from Wikipedia - version of the Saturday, January 02, 2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.