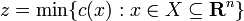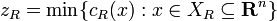Relaxation (approximation)

In mathematical optimization and related fields, relaxation is a modeling strategy. A relaxation is an approximation of a difficult problem by a nearby problem that is easier to solve. A solution of the relaxed problem provides information about the original problem.

For example, a linear programming relaxation of an integer programming problem removes the integrality constraint and so allows non-integer rational solutions. A Lagrangian relaxation of a complicated problem in combinatorial optimization penalizes violations of some constraints, allowing an easier relaxed problem to be solved. Relaxation techniques complement or supplement branch and bound algorithms of combinatorial optimization; linear programming and Lagrangian relaxations are used to obtain bounds in branch-and-bound algorithms for integer programming.[1]

The modeling strategy of relaxation should not be confused with iterative methods of relaxation, such as successive over-relaxation (SOR); iterative methods of relaxation are used in solving problems in differential equations, linear least-squares, and linear programming.[2][3][4] However, iterative methods of relaxation have been used to solve Lagrangian relaxations.[5]

Definition

A relaxation of the minimization problem

z = \min \{c(x) : x \in X \subseteq \mathbf{R}^{n}\}

is another minimization problem of the form

z_R = \min \{c_R(x) : x \in X_R \subseteq \mathbf{R}^{n}\}

with these two properties

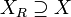
  1. X_R \supseteq X
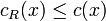  2. c_R(x) \leq c(x) for all x \in X.

The first property states that the original problem's feasible domain is a subset of the relaxed problem's feasible domain. The second property states that the original problem's objective-function is greater than or equal to the relaxed problem's objective-function.[1]

Properties

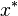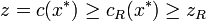If x^* is an optimal solution of the original problem, then x^* \in X \subseteq X_R and z = c(x^*) \geq c_R(x^*)\geq z_R. Therefore 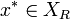x^* \in X_R provides an upper bound on z_R.

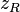If in addition to the previous assumptions, 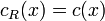c_R(x)=c(x), \forall x\in X, the following holds: If an optimal solution for the relaxed problem is feasible for the original problem, then it is optimal for the original problem.[1]

Some relaxation techniques

Notes

  1. 1 2 3 Geoffrion (1971)
  2. ↑ Murty, Katta G. (1983). "16 Iterative methods for linear inequalities and linear programs (especially 16.2 Relaxation methods, and 16.4 Sparsity-preserving iterative SOR algorithms for linear programming)". Linear programming. New York: John Wiley & Sons, Inc. pp. 453–464. ISBN 0-471-09725-X. MR 720547.
  3. ↑ Goffin, J.-L. (1980). "The relaxation method for solving systems of linear inequalities". Math. Oper. Res. 5 (3): 388–414. doi:10.1287/moor.5.3.388. JSTOR 3689446. MR 594854.
  4. ↑ Minoux, M. (1986). Mathematical programming: Theory and algorithms. Egon Balas (foreword) (Translated by Steven Vajda from the (1983 Paris: Dunod) French ed.). Chichester: A Wiley-Interscience Publication. John Wiley & Sons, Ltd. pp. xxviii+489. ISBN 0-471-90170-9. MR 868279. (2008 Second ed., in French: Programmation mathématique: Théorie et algorithmes. Editions Tec & Doc, Paris, 2008. xxx+711 pp. ISBN 978-2-7430-1000-3.. MR 2571910)
  5. ↑ Relaxation methods for finding feasible solutions to linear inequality systems arise in linear programming and in Lagrangian relaxation. Goffin (1980) and Minoux (1986)|loc=Section 4.3.7, pp. 120–123 cite Shmuel Agmon (1954), and Theodore Motzkin and Isaac Schoenberg (1954), and L. T. Gubin, Boris T. Polyak, and E. V. Raik (1969).

References

This article is issued from Wikipedia - version of the Saturday, April 12, 2014. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.