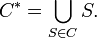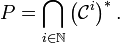Pseudo-arc

In general topology, the pseudo-arc is the simplest nondegenerate hereditarily indecomposable continuum. The pseudo-arc is an arc-like homogeneous continuum. R.H. Bing proved that, in a certain well-defined sense, most continua in Rⁿ, n ≥ 2, are homeomorphic to the pseudo-arc.

History

In 1920, Bronisław Knaster and Kazimierz Kuratowski asked whether a nondegenerate homogeneous continuum in the Euclidean plane R² must be a Jordan curve. In 1921, Stefan Mazurkiewicz asked whether a nondegenerate continuum in R² that is homeomorphic to each of its nondegenerate subcontinua must be an arc. In 1922, Knaster discovered the first example of a homogeneous hereditarily indecomposable continuum K, later named the pseudo-arc, giving a negative answer to the Mazurkiewicz question. In 1948, R.H. Bing proved that Knaster's continuum is homogeneous, i.e., for any two of its points there is a homeomorphism taking one to the other. Yet also in 1948, Edwin Moise showed that Knaster's continuum is homeomorphic to each of its non-degenerate subcontinua. Due to its resemblance to the fundamental property of the arc, namely, being homeomorphic to all its nondegenerate subcontinua, Moise called his example M a pseudo-arc.^[1] Bing's construction is a modification of Moise's construction of M, which he had first heard described in a lecture. In 1951, Bing proved that all hereditarily indecomposable arc-like continua are homeomorphic — this implies that Knaster's K, Moise's M, and Bing's B are all homeomorphic. Bing also proved that the pseudo-arc is typical among the continua in a Euclidean space of dimension at least 2 or an infinite-dimensional separable Hilbert space.^[2]

Construction

The following construction of the pseudo-arc follows (Wayne Lewis 1999).

Chains

At the heart of the definition of the pseudo-arc is the concept of a chain, which is defined as follows:

A chain is a finite collection of open sets

\mathcal{C}=\{C_1,C_2,\ldots,C_n\}

in a metric space such that

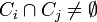C_i\cap C_j\ne\emptyset

if and only if

|i-j|\le1.

The elements of a chain are called its links, and a chain is called an ε-chain if each of its links has diameter less than ε.

While being the simplest of the type of spaces listed above, the pseudo-arc is actually very complex. The concept of a chain being crooked (defined below) is what endows the pseudo-arc with its complexity. Informally, it requires a chain to follow a certain recursive zig-zag pattern in another chain. To 'move' from the mth link of the larger chain to the nth, the smaller chain must first move in a crooked manner from the mth link to the (n-1)th link, then in a crooked manner to the (m+1)th link, and then finally to the nth link.

More formally:

Let

\mathcal{C}

and

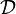\mathcal{D}

be chains such that

each link of 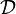$\mathcal{D}$ is a subset of a link of $\mathcal{C}$ , and
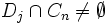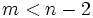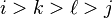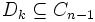for any indices i, j, m, and n with $D_i\cap C_m\ne\emptyset$ , $D_j\cap C_n\ne\emptyset$ , and $m<n-2$ , there exist indices $k$ and $\ell$ with $i<k<\ell<j$ (or $i>k>\ell>j$ ) and $D_k\subseteq C_{n-1}$ and $D_\ell\subseteq C_{m+1}.$

Then

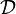\mathcal{D}

is crooked in

\mathcal{C}.

Pseudo-arc

For any collection C of sets, let $C^{*}$ denote the union of all of the elements of C. That is, let

C^*=\bigcup_{S\in C}S.

The pseudo-arc is defined as follows:

Let p and q be distinct points in the plane and

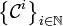\left\{\mathcal{C}^{i}\right\}_{i\in\mathbb{N}}

be a sequence of chains in the plane such that for each i,

the first link of 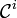$\mathcal{C}^i$ contains p and the last link contains q,
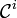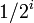the chain $\mathcal{C}^i$ is a $1/2^i$ -chain,
the closure of each link of 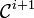$\mathcal{C}^{i+1}$ is a subset of some link of 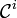$\mathcal{C}^i$ , and
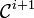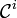the chain $\mathcal{C}^{i+1}$ is crooked in $\mathcal{C}^i$ .

Let

P=\bigcap_{i\in\mathbb{N}}\left(\mathcal{C}^i\right)^{*}.

Then P is a pseudo-arc.

Notes

↑ (George W. Henderson 1960) later showed that a decomposable continuum homeomorphic to all its nondenerate subcontinua must be an arc.
↑ The history of the discovery of the pseudo-arc is described in (Nadler 1992), pp 228–229.

References

R.H. Bing, A homogeneous indecomposable plane continuum, Duke Math. J., 15:3 (1948), 729–742
R.H. Bing, Concerning hereditarily indecomposable continua, Pacific J. Math., 1 (1951), 43–51
George W. Henderson, Proof that every compact decomposable continuum which is topologically equivalent to each of its nondegenerate subcontinua is an arc. Annals of Math., 72 (1960), 421–428
Bronisław Knaster, Un continu dont tout sous-continu est indécomposable. Fundamenta Mathematicae 3 (1922): pp. 247–286
Wayne Lewis, The Pseudo-Arc, Bol. Soc. Mat. Mexicana, 5 (1999), 25–77
Edwin Moise, An indecomposable plane continuum which is homeomorphic to each of its nondegenerate subcontinua, Trans. Amer. Math. Soc., 63, no. 3 (1948), 581–594
Sam B. Nadler, Jr, Continuum theory. An introduction. Pure and Applied Mathematics, Marcel Dekker (1992) ISBN 0-8247-8659-9

This article is issued from Wikipedia - version of the Monday, June 22, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.