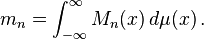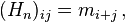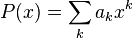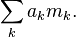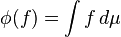Moment problem

In mathematics, a moment problem arises as the result of trying to invert the mapping that takes a measure μ to the sequences of moments

m_n = \int_{-\infty}^\infty x^n \,d\mu(x)\,.\,\!

More generally, one may consider

m_n = \int_{-\infty}^\infty M_n(x) \,d\mu(x)\,.\,\!

for an arbitrary sequence of functions M_n.

Introduction

In the classical setting, μ is a measure on the real line, and M is the sequence { xⁿ : n = 0, 1, 2, ... }. In this form the question appears in probability theory, asking whether there is a probability measure having specified mean, variance and so on, and whether it is unique.

There are three named classical moment problems: the Hamburger moment problem in which the support of μ is allowed to be the whole real line; the Stieltjes moment problem, for [0, +∞); and the Hausdorff moment problem for a bounded interval, which without loss of generality may be taken as [0, 1].

Existence

A sequence of numbers m_n is the sequence of moments of a measure μ if and only if a certain positivity condition is fulfilled; namely, the Hankel matrices H_n,

(H_n)_{ij} = m_{i+j}\,,\,\!

should be positive semi-definite. A condition of similar form is necessary and sufficient for the existence of a measure 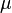$\mu$ supported on a given interval [a, b].

One way to prove these results is to consider the linear functional $\scriptstyle\varphi$ that sends a polynomial

P(x) = \sum_k a_k x^k \,\!

\sum_k a_k m_k.\,\!

If m_kn are the moments of some measure μ supported on [a, b], then evidently

φ(P) ≥ 0 for any polynomial P that is non-negative on [a, b].

(1)

Vice versa, if (1) holds, one can apply the M. Riesz extension theorem and extend 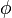$\phi$ to a functional on the space of continuous functions with compact support C₀([a, b]), so that

$\qquad \varphi(f) \ge 0\text{ for any } f \in C_0([a,b])$

(2)

such that ƒ ≥ 0 on [a, b].

By the Riesz representation theorem, (2) holds iff there exists a measure μ supported on [a, b], such that

\phi(f) = \int f \, d\mu\,\!

for every ƒ ∈ C₀([a, b]).

Thus the existence of the measure 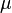$\mu$ is equivalent to (1). Using a representation theorem for positive polynomials on [a, b], one can reformulate (1) as a condition on Hankel matrices.

See Shohat & Tamarkin 1943 and Krein & Nudelman 1977 for more details.

Uniqueness (or determinacy)

The uniqueness of μ in the Hausdorff moment problem follows from the Weierstrass approximation theorem, which states that polynomials are dense under the uniform norm in the space of continuous functions on [0, 1]. For the problem on an infinite interval, uniqueness is a more delicate question; see Carleman's condition, Krein's condition and Akhiezer (1965).

Variations

An important variation is the truncated moment problem, which studies the properties of measures with fixed first k moments (for a finite k). Results on the truncated moment problem have numerous applications to extremal problems, optimisation and limit theorems in probability theory. See also: Chebyshev–Markov–Stieltjes inequalities and Krein & Nudelman 1977.

References

Shohat, James Alexander; Tamarkin, Jacob D. (1943). The Problem of Moments. New York: American mathematical society.
Akhiezer, Naum I. (1965). The classical moment problem and some related questions in analysis. New York: Hafner Publishing Co. (translated from the Russian by N. Kemmer)
Krein, M. G.; Nudelman, A. A. (1977). The Markov moment problem and extremal problems. Ideas and problems of P. L. Chebyshev and A. A. Markov and their further development. Translations of Mathematical Monographs, Vol. 50. American Mathematical Society, Providence, R.I. (Translated from the Russian by D. Louvish)

This article is issued from Wikipedia - version of the Wednesday, December 09, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.