Prakash Belkale
Prakash Belkale (born May 1973, Bangalore) is an Indian-American mathematician, specializing in algebraic geometry and representation theory.[1][2]
Education and career
Belkale received his Ph.D. in 1999 from the University of Chicago with thesis advisor Madhav Nori.[3]
in 2003 Belkale and Patrick Brosnan disproved Kontsevich's Spanning-Tree Conjecture (first published in 1997).[4]
Let G be a finite connected graph. The Kirchhoff polynomial of G is a certain homogeneous polynomial whose degree is equal to the first betti number of G. These polynomials appear in the study of electrical circuits and in the evaluation of Feynman amplitudes. Motivated by work of D. Kreimer and D. J. Broadhurst associating multiple zeta values to certain Feynman integrals, Kontsevich conjectured that the number of zeros of a Kirchhoff polynomial over the field with q elements is always a polynomial function of q. We show that this conjecture is false by relating the schemes defined by Kirchhoff polynomials to the representation spaces of matroids. Moreover, using Mnev's universality theorem, we show that these schemes essentially generate all arithmetic of schemes of finite type over the integers.[4]
Belkale works on enumerative algebraic geometry, quantum cohomology and moduli spaces of vector bundles on curves (conformal blocks and strange duality), and the Schubert calculus and its connections to intersection theory and representation theory. He is a professor at the University of North Carolina at Chapel Hill.
In 2010 he was an invited speaker at the International Congress of Mathematicians in Hyderabad and gave a talk The tangent space to an enumerative problem. In December 2014 he was elected a Fellow of the American Mathematical Society.
Selected publications
- "Local systems on
– 
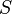for 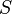a finite set". Compositio Mathematica 129 (1): 67–86. 2001. doi:10.1023/A:1013195625868. - "Invariant Theory of GL(n) and intersection theory of Grassmannians". Internat. Math. Research Notes 69: 3709–3721. 2004.
- with P. Brosnan: "Periods and Igusa Local Zeta functions". Int. Math. Res. Notices 49: 2655–2670. 2003.
- with S. Kumar: "Eigenvalue problem and a new product on cohomology of flag varieties". Invent. Math. 166: 185–228. 2006. doi:10.1007/s00222-006-0516-x.
- "The strange duality conjecture for generic curves". J. Amer. Math. Soc. 21: 235–258. 2008. doi:10.1090/S0894-0347-07-00569-3. MR 2350055.
- "Quantum generalization of the Horn conjecture" (PDF). J. Amer. Math. Soc. 21: 365–408. 2008. doi:10.1090/S0894-0347-07-00584-X.
- "The tangent space to an enumerative problem" (PDF). Proceedings of the International Congress of Mathematicians (Hyderabad, India). 2010.
- "Unitarity of the KZ/Hitchin connection on conformal blocks in genus 0 for arbitrary Lie algebras". J. Math. Pures Appl. 98: 367–398. 2012. doi:10.1016/j.matpur.2012.02.008. Arxiv
References
- ↑ Michel Brion (2011). Restriction de représentations et projection d'orbites coadjointes, d'après Belkale, Kumar et Ressayre. Bourbaki Seminar 1043.
- ↑ Christian Pauly (2008). La dualité étrange, d’après P. Belkale, A. Marian et D. Oprea. Bourbaki Seminar 994.
- ↑ Prakash Belkale at the Mathematics Genealogy Project
- 1 2 Belkale, Prakash; Brosnan, Patrick (2003). "Matroids, motives and a conjecture of Kontsevich". Duke Math. J. 116: 1–188. doi:10.1215/s0012-7094-03-11615-4.
External links
|