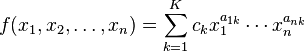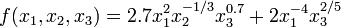Posynomial

A posynomial, also known as a posinomial in some literature, is a function of the form

f(x_1, x_2, \dots, x_n) = \sum_{k=1}^K c_k x_1^{a_{1k}} \cdots x_n^{a_{nk}}

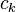where all the coordinates $x_i$ and coefficients $c_k$ are positive real numbers, and the exponents 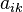$a_{ik}$ are real numbers. Posynomials are closed under addition, multiplication, and nonnegative scaling.

For example,

f(x_1, x_2, x_3) = 2.7 x_1^2x_2^{-1/3}x_3^{0.7} + 2x_1^{-4}x_3^{2/5}

is a posynomial.

Posynomials are not the same as polynomials in several independent variables. A polynomial's exponents must be non-negative integers, but its independent variables and coefficients can be arbitrary real numbers; on the other hand, a posynomial's exponents can be arbitrary real numbers, but its independent variables and coefficients must be positive real numbers. This terminology was introduced by Richard J. Duffin, Elmor L. Peterson, and Clarence Zener in their seminal book on Geometric programming.

References

Richard J. Duffin; Elmor L. Peterson; Clarence Zener (1967). Geometric Programming. John Wiley and Sons. p. 278. ISBN 0-471-22370-0.

Stephen P Boyd; Lieven Vandenberghe (2004). Convex optimization (pdf version). Cambridge University Press. ISBN 0-521-83378-7.

Harvir Singh Kasana; Krishna Dev Kumar (2004). Introductory operations research: theory and applications. Springer. ISBN 3-540-40138-5.

Weinstock, D.; Appelbaum, J. "Optimal solar field design of stationary collectors". J. of Solar Energy Engineering 126 (3): 898–905. doi:10.1115/1.1756137.

External links

S. Boyd, S. J. Kim, L. Vandenberghe, and A. Hassibi, A Tutorial on Geometric Programming

This article is issued from Wikipedia - version of the Friday, May 15, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.