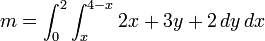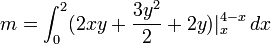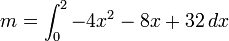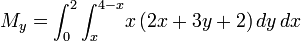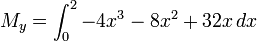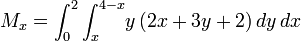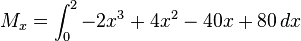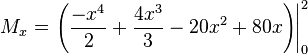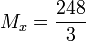Planar lamina

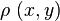In mathematics, a planar lamina is a closed set in a plane of mass $m$ and surface density $\rho\ (x,y)$ such that:

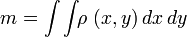m = \int\int_{}{}\rho\ (x,y)\,dx\,dy

, over the closed set.

The center of mass of the lamina is at the point

\left(\frac{M_y}{m},\frac{M_x}{m}\right)

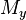where $M_y$ moment of the entire lamina about the x-axis and $M_x$ moment of the entire lamina about the y-axis.

M_y = \lim_{m,n \to \infty}\,\sum_{i=1}^{m}\,\sum_{j=1}^{n}\,x{_{ij}}^{*}\,\rho\ (x{_{ij}}^{*},y{_{ij}}^{*})\,\Delta\Alpha = \iint_{}{} x\, \rho\ (x,y)\,dx\,dy

, over the closed surface.

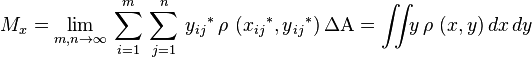M_x = \lim_{m,n \to \infty}\,\sum_{i=1}^{m}\,\sum_{j=1}^{n}\,y{_{ij}}^{*}\,\rho\ (x{_{ij}}^{*},y{_{ij}}^{*})\,\Delta\Alpha = \iint_{}{} y\, \rho\ (x,y)\,dx\,dy

, over the closed surface.

Example 1.

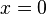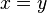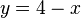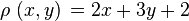Find the center of mass of a lamina with edges given by the lines $x=0$ , $x=y$ and $y=4-x$ , where the density is given as $\rho\ (x,y)\,=2x+3y+2$ .

m = \int_0^2{\int_x^{4-x}}_{}{}\,2x+3y+2\,dy\,dx

m=\int_0^2 (2xy+\frac{3y^2}{2}+2y)|_x^{4-x}\,dx

m=\int_0^2 -4x^2-8x+32\,dx

m= (\frac{-4x^3}{3}-4x^2+32x)|_0^2

m= \frac{112}{3}

M_y = \int_0^2{\int_x^{4-x}}{}{}x\,(2x+3y+2)\,dy\,dx

M_y=\int_0^2 (2x^2y+\frac{3xy^2}{2}+2xy)|_x^{4-x}\,dx

M_y=\int_0^2 -4x^3-8x^2+32x\,dx

M_y= (-x^4-\frac{8x^3}{3}+16x^2)|_0^2

M_y= \frac{80}{3}

M_x = \int_0^2{\int_x^{4-x}}{}{}y\,(2x+3y+2)\,dy\,dx

M_x = \int_0^2 (xy^2+y^3+y^2)|_x^{4-x}\,dx

M_x = \int_0^2 -2x^3+4x^2-40x+80\,dx

M_x= \left.\left(\frac{-x^4}{2}+\frac{4x^3}{3}-20x^2+80x\right)\right|_0^2

M_x= \frac{248}{3}

center of mass is at the point

\left(\frac{\frac{80}{3}}{\frac{112}{3}},\frac{\frac{248}{3}}{\frac{112}{3}}\right)=\left(\frac{5}{7},\frac{31}{14}\right)

Planar laminas can be used to determine moments of inertia, or center of mass.

This article is issued from Wikipedia - version of the Saturday, October 17, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.