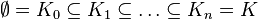Persistent homology
See homology for an introduction to the notation.
Persistent homology is a method for computing topological features of a space at different spatial resolutions. More persistent features are detected over a wide range of length and are deemed more likely to represent true features of the underlying space, rather than artifacts of sampling, noise, or particular choice of parameters.[1]
To find the persistent homology of a space, the space must first be represented as a simplicial complex. A distance function on the underlying space corresponds to a filtration of the simplicial complex, that is a nested sequence of increasing subsets.
Formally, consider a real-valued function on a simplicial complex  that is non-decreasing on increasing sequences of faces, so
that is non-decreasing on increasing sequences of faces, so  whenever
whenever 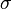 is a face of
is a face of  in
in 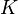 . Then for every
. Then for every  the sublevel set
the sublevel set ![K(a)=f^{-1}(-\infty, a]](../I/m/efcb3f958d48135975140771a61b6dd2.png) is a subcomplex of K, and the ordering of the values of
is a subcomplex of K, and the ordering of the values of  on the simplices in
on the simplices in 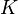 (which is in practice always finite) induces an ordering on the sublevel complexes that defines the filtration
(which is in practice always finite) induces an ordering on the sublevel complexes that defines the filtration
When 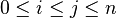 , the inclusion
, the inclusion  induces a homomorphism
induces a homomorphism  on the simplicial homology groups for each dimension
on the simplicial homology groups for each dimension  . The
. The 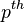 persistent homology groups are the images of these homomorphisms, and the
persistent homology groups are the images of these homomorphisms, and the 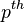 persistent Betti numbers
persistent Betti numbers  are the ranks of those groups.[2] Persistent Betti numbers for
are the ranks of those groups.[2] Persistent Betti numbers for  coincide with
the predecessor of persistence homology, i.e. the size function.[3]
coincide with
the predecessor of persistence homology, i.e. the size function.[3]
There are various software packages for computing persistence intervals of a finite filtration, such as javaPlex, Dionysus, Perseus, PHAT, DIPHA, Gudhi, and the phom and TDA R packages.
See also
References
- ↑ Carlsson, Gunnar (2009). "Topology and data". AMS Bulletin 46(2), 255–308.
- ↑ Edelsbrunner, H and Harer, J (2010). Computational Topology: An Introduction. American Mathematical Society.
- ↑ Verri, A, Uras, C, Frosini, P and Ferri, M (1993). On the use of size functions for shape analysis, Biological Cybernetics, 70, 99–107.