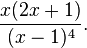Pentagonal pyramidal number
A pentagonal pyramidal number is a figurate number that represents the number of objects in a pyramid with a pentagonal base.[1] The nth pentagonal pyramidal number is equal to the sum of the first n pentagonal numbers.
The first few pentagonal pyramidal numbers are:
1, 6, 18, 40, 75, 126, 196, 288, 405, 550, 726, 936, 1183, 1470, 1800, 2176, 2601, 3078, 3610, 4200, 4851, 5566, 6348, 7200, 8125, 9126 (sequence A002411 in OEIS).
The formula for the nth pentagonal pyramidal number is[2]
so the nth pentagonal pyramidal number is the average of n2 and n3.[2] The nth pentagonal pyramidal number is also n times the nth triangular number.
The generating function for the pentagonal pyramidal numbers is[1]
See also
References
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
This article is issued from Wikipedia - version of the Tuesday, November 10, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.