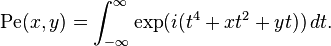Pearcey integral

In mathematics, the Pearcey integral is defined as[1]

\operatorname{Pe}(x,y)=\int_{-\infty}^\infty \exp(i(t^4+xt^2+yt)) \, dt.

The Percey integral is a class of canonical diffraction integrals, often used in wave propagation and optical diffraction problems[2]

References

  1. ↑ Frank J. Oliver, Daniel W. Lozier, NIST Handbook of Mathematical Functions, p. 777, Cambridge, 2010
  2. ↑ R.B.Paris, Hadamard Expansions and Hyperasymptotic Evaluation, p. 207, Encyclopedia of Mathematics and its Applications, 141, Cambridge, 2011


This article is issued from Wikipedia - version of the Wednesday, August 12, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.