One-way quantum computer

The one-way or measurement based quantum computer (MBQC) is a method of quantum computing that first prepares an entangled resource state, usually a cluster state or graph state, then performs single qubit measurements on it. It is "one-way" because the resource state is destroyed by the measurements.

The outcome of each individual measurement is random, but they are related in such a way that the computation always succeeds. In general the choices of basis for later measurements need to depend on the results of earlier measurements, and hence the measurements cannot all be performed at the same time.

Equivalence to quantum circuit model

Any one-way computation can be made into a quantum circuit by using quantum gates to prepare the resource state. For cluster and graph resource states, this requires only one two-qubit gate per bond, so is efficient.

Conversely, any quantum circuit can be simulated by a one-way computer using a two-dimensional cluster state as the resource state, by laying out the circuit diagram on the cluster; Z measurements (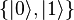\{|0\rangle,|1\rangle\} basis) remove physical qubits from the cluster, while measurements in the X-Y plane (|0\rangle\pm e^{i\theta}|1\rangle basis) teleport the logical qubits along the "wires" and perform the required quantum gates.[1] This is also polynomially efficient, as the required size of cluster scales as the size of the circuit (qubits x timesteps), while the number of measurement timesteps scales as the number of circuit timesteps.

Topological cluster state quantum computer

Measurement-based computation on a periodic 3D lattice cluster state can be used to implement topological quantum error correction.[2] Topological cluster state computation is closely related to Kitaev's toric code, as the 3D topological cluster state can be constructed and measured over time by a repeated sequence of gates on a 2D array.[3]

Implementations

One-way quantum computation has been demonstrated by running the 2 qubit Grover's algorithm on a 2x2 cluster state of photons.[4][5] A linear optics quantum computer based on one-way computation has been proposed.[6]

Cluster states have also been created in optical lattices,[7] but were not used for computation as the atom qubits were too close together to measure individually.

AKLT state as a resource

It has been shown that the (spin \tfrac{3}{2}) AKLT state on a 2D Honeycomb lattice can be used as a resource for MBQC.[8][9] More recently it has been shown that a spin-mixture AKLT state can be used as a resource.[10]

References

  1. ↑ R. Raussendorf, D. E. Browne, and H. J. Briegel (2003). "Measurement based Quantum Computation on Cluster States". Phys. Rev. A 68 (2): 022312. arXiv:quant-ph/0301052. Bibcode:2003PhRvA..68b2312R. doi:10.1103/PhysRevA.68.022312.
  2. ↑ Robert Raussendorf, Jim Harrington, Kovid Goyal (2007). "Topological fault-tolerance in cluster state quantum computation". New Journal of Physics 9: 199. arXiv:quant-ph/0703143. Bibcode:2007NJPh....9..199R. doi:10.1088/1367-2630/9/6/199.
  3. ↑ Robert Raussendorf, Jim Harrington (2007). "Fault-tolerant quantum computation with high threshold in two dimensions". Phys. Rev. Lett. 98: 190504. doi:10.1103/physrevlett.98.190504.
  4. ↑ P. Walther, K. J. Resch, T. Rudolph, E. Schenck, H. Weinfurter, V. Vedral, M. Aspelmeyer and A. Zeilinger (2005). "Experimental one-way quantum computing". Nature 434 (7030): 169–76. arXiv:quant-ph/0503126. Bibcode:2005Natur.434..169W. doi:10.1038/nature03347. PMID 15758991.
  5. ↑ Robert Prevedel, Philip Walther, Felix Tiefenbacher, Pascal Böhi, Rainer Kaltenbaek, Thomas Jennewein and Anton Zeilinger (2007). "High-speed linear optics quantum computing using active feed-forward". Nature 445 (7123): 65–69. arXiv:quant-ph/0701017. Bibcode:2007Natur.445...65P. doi:10.1038/nature05346. PMID 17203057.
  6. ↑ Daniel E. Browne, Terry Rudolph (2005). "Resource-efficient linear optical quantum computation". Physical Review Letters 95 (1): 010501. arXiv:quant-ph/0405157. Bibcode:2005PhRvL..95a0501B. doi:10.1103/PhysRevLett.95.010501. PMID 16090595.
  7. ↑ Olaf Mandel, Markus Greiner, Artur Widera, Tim Rom, Theodor W. Hänsch and Immanuel Bloch (2003). "Controlled collisions for multi-particle entanglement of optically trapped atoms". Nature 425 (6961): 937–40. arXiv:quant-ph/0308080. Bibcode:2003Natur.425..937M. doi:10.1038/nature02008. PMID 14586463.
  8. ↑ Tzu-Chieh Wei, Ian Affleck, and Robert Raussendorf (2012). "Two-dimensional Affleck-Kennedy-Lieb-Tasaki state on the honeycomb lattice is a universal resource for quantum computation". PRA 86 (032328). arXiv:1009.2840. doi:10.1103/PhysRevA.86.032328.
  9. ↑ Akimasa Miyake (2011). "Quantum computational capability of a 2D valence bond solid phase". Annals of Physics 236 (7): 1656–1671. arXiv:1009.3491. doi:10.1016/j.aop.2011.03.006.
  10. ↑ Tzu-Chieh Wei, Poya Haghnegahdar, Robert Raussendorf. "Spin mixture AKLT states for universal quantum computation".
General
This article is issued from Wikipedia - version of the Wednesday, October 14, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.