Nice name

In set theory, a nice name is used in forcing to impose an upper bound on the number of subsets in the generic model. It is used in the context of forcing to prove independence results in set theory such as Easton's theorem.

Formal definition

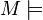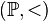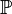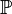Let $M \models$ ZFC be transitive, $(\mathbb{P}, <)$ a forcing notion in $M$ , and suppose $G \subseteq \mathbb{P}$ is generic over $M$ . Then for any $\mathbb{P}$ -name in $M$ , $\tau$ ,

$\eta$ is a nice name for a subset of $\tau$ if $\eta$ is a $\mathbb{P}$ -name satisfying the following properties:

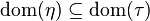
(1) $\textrm{dom}(\eta) \subseteq \textrm{dom}(\tau)$

(2) For all 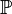$\mathbb{P}$ -names 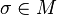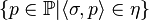$\sigma \in M$ , $\{p \in \mathbb{P}| \langle\sigma, p\rangle \in \eta\}$ forms an antichain.

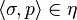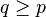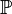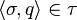(3) (Natural addition): If $\langle\sigma, p\rangle \in \eta$ , then there exists $q \geq p$ in $\mathbb{P}$ such that $\langle\sigma, q\rangle \in \tau$ .

References

Kenneth Kunen (1980) Set theory: an introduction to independence proofs, Volume 102 of Studies in logic and the foundations of mathematics (Elsevier) ISBN 0-444-85401-0, p.208

This article is issued from Wikipedia - version of the Sunday, November 22, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.