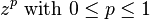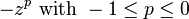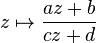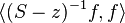Nevanlinna function
In mathematics, in the field of complex analysis, a Nevanlinna function is a complex function which is an analytic function on the open upper half-plane H and has non-negative imaginary part. A Nevanlinna function maps the upper half-plane to itself or to a real constant,[1] but is not necessarily injective or surjective. Functions with this property are sometimes also known as Herglotz, Pick or R functions.
Integral representation
Every Nevanlinna function N admits a representation
where C is a real constant, D is a non-negative constant and μ is a Borel measure on R satisfying the growth condition
Conversely, every function of this form turns out to be a Nevanlinna function. The constants in this representation are related to the function N via
and the Borel measure μ can be recovered from N by employing the Stieltjes inversion formula (related to the inversion formula for the Stieltjes transformation):
A very similar representation of functions is also called the Poisson representation.[2]
Examples
- Some elementary examples of Nevanlinna functions follow (with appropriately chosen branch cuts in the first three). (
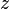 can be replaced by
can be replaced by  for some real number
for some real number 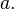 )
)
- These are injective but when p does not equal 1 or −1 they are not surjective and can be rotated to some extent around the origin, such as
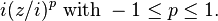
- These are injective but when p does not equal 1 or −1 they are not surjective and can be rotated to some extent around the origin, such as
- A sheet of
 such as the one with
such as the one with 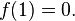
- A sheet of
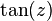 (an example that is surjective but not injective)
(an example that is surjective but not injective)
- is a Nevanlinna function if (but not only if)
 is a positive real number and
is a positive real number and  This is equivalent to the set of such transformations that map the real axis to itself. One may then add any constant in the upper half-plane, and move the pole into the lower half-plane, giving new values for the parameters. Example:
This is equivalent to the set of such transformations that map the real axis to itself. One may then add any constant in the upper half-plane, and move the pole into the lower half-plane, giving new values for the parameters. Example: 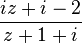
-
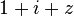 and
and 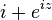 are examples which are entire functions. The second is neither injective nor surjective.
are examples which are entire functions. The second is neither injective nor surjective. - If S is a self-adjoint operator in a Hilbert space and f is an arbitrary vector, then the function
- is a Nevanlinna function.
- If M(z) and N(z) are Nevanlinna functions, then the composition M(N(z)) is a Nevanlinna function as well.
References
- ↑ A real number is not considered to be in the upper half-plane.
- ↑ See for example Section 4, "Poisson representation", of Louis de Branges. Hilbert spaces of entire functions. Prentice-Hall.. De Branges gives a form for functions whose real part is non-negative in the upper half-plane.
- Vadim Adamyan, ed. (2009). Modern analysis and applications. p. 27. ISBN 3-7643-9918-X.
- Naum Ilyich Akhiezer and I. M. Glazman (1993). Theory of linear operators in Hilbert space. ISBN 0-486-67748-6.
- Marvin Rosenblum and James Rovnyak (1994). Topics in Hardy Classes and Univalent Functions. ISBN 3-7643-5111-X.
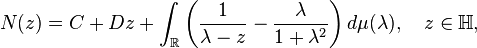
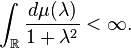
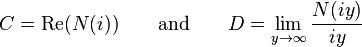
![\mu((\lambda_1,\lambda_2]) = \lim_{\delta\rightarrow0} \lim_{\varepsilon\rightarrow 0} \frac{1}{\pi} \int_{\lambda_1+\delta}^{\lambda_2+\delta} \mathrm{Im}(N(\lambda+i\varepsilon))d\lambda.](../I/m/b317bd6a7296e08cbef2562f359a4155.png)