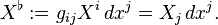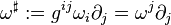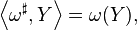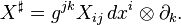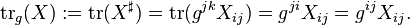Musical isomorphism

In mathematics, the musical isomorphism (or canonical isomorphism) is an isomorphism between the tangent bundle $TM$ and the cotangent bundle $T * M$ of a Riemannian manifold given by its metric. There are similar isomorphisms on symplectic manifolds. The term musical refers to the use of the symbols ♭ and ♯.^[1]

It is also known as raising and lowering indices.

Discussion

Let $(M, g)$ be a Riemannian manifold. Suppose ${\partial i}$ is a local frame for the tangent bundle $TM$ with dual coframe ${dx i}.$ Then, locally, we may express the Riemannian metric (which is a $2$ -covariant tensor field which is symmetric and positive-definite) as $g = g ij dx i \otimes dx j$ (where we employ the Einstein summation convention). Given a vector field $X = X i \partial i$ we define its flat by

X^\flat := g_{ij} X^i \, dx^j=X_j \, dx^j.

This is referred to as "lowering an index". Using the traditional diamond bracket notation for inner product defined by $g$ , we obtain the somewhat more transparent relation

X^\flat (Y) = \langle X, Y \rangle

for all vectors $X$ and $Y$ .

Alternatively, given a covector field $ω = ω i dx i$ we define its sharp by

\omega^\sharp :=g^{ij} \omega_i \partial_j = \omega^j \partial_j

where $g ij$ are the elements of the inverse matrix to $g ij$ . Taking the sharp of a covector field is referred to as "raising an index". In inner product notation, this reads

\left \langle \omega^\sharp, Y \right \rangle = \omega(Y),

for $ω$ an arbitrary covector and $Y$ an arbitrary vector.

Through this construction we have two inverse isomorphisms

\flat:TM \to T^*M, \qquad \sharp:T^*M \to TM.

These are isomorphisms of vector bundles and hence we have, for each $p$ in $M$ , inverse vector space isomorphisms between $T p M$ and $T * p M$ .

The musical isomorphisms may also be extended to the bundles

\bigotimes ^k TM, \qquad \bigotimes ^k T^*M.

It must be stated which index is to be raised or lowered. For instance, consider the $(2, 0)$ tensor field $X = X ij dx i \otimes dx j$ . Raising the second index, we get the $(1, 1)$ tensor field

X^\sharp = g^{jk}X_{ij} \, dx^i \otimes \partial _k.

Trace of a tensor through a metric

Given a $(0, 2)$ tensor field $X = X ij dx i \otimes dx j$ , we define the trace of $X$ through the metric $g$ by

\operatorname{tr}_g(X):=\operatorname{tr}(X^\sharp)=\operatorname{tr}(g^{jk}X_{ij}) = g^{ji}X_{ij} = g^{ij}X_{ij}.

Observe that the definition of trace is independent of the choice of index we raise since the metric tensor is symmetric.

References

↑ http://mathoverflow.net/questions/69074/the-origin-of-the-musical-isomorphisms

This article is issued from Wikipedia - version of the Monday, January 25, 2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.

Musical isomorphism

Discussion

Trace of a tensor through a metric

See also

References