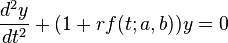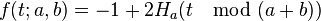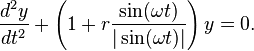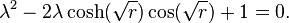Meissner equation
The Meissner equation is a linear ordinary differential equation that is a special case of Hill's equation with the periodic function given as a square wave.[1] [2] There are many ways to write the Meissner equation. One is as
or
where
and 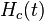 is the Heaviside function shifted to
is the Heaviside function shifted to  . Another version is
. Another version is
The Meissner equation was first studied as a toy problem for certain resonance problems. It is also useful for understand resonance problems in evolutionary biology.
Because the time-dependence is piecewise linear, many calculations can be performed exactly, unlike for the Mathieu equation. When 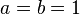 , the Floquet exponents are roots of the quadratic equation
, the Floquet exponents are roots of the quadratic equation
The determinant of the Floquet matrix is 1, implying that origin is a center if 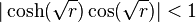 and a saddle node otherwise.
and a saddle node otherwise.