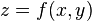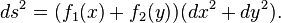Liouville surface
In the mathematical field of differential geometry a Liouville surface is a type of surface which in local coordinates may be written as a graph in R3
such that the first fundamental form is of the form
Sometimes a metric of this form is called a Liouville metric. Every surface of revolution is a Liouville surface.
References
- Gelfand, I.M. and Fomin, S.V. (2000). Calculus of variations. Dover. ISBN 0-486-41448-5. (Translated from the Russian by R. Silverman.)
- Guggenheimer, Heinrich (1977). "Chapter 11: Inner geometry of surfaces". Differential Geometry. Dover. ISBN 0-486-63433-7.
This article is issued from Wikipedia - version of the Monday, March 18, 2013. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.