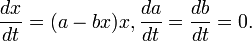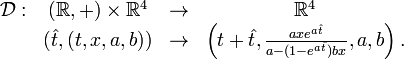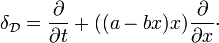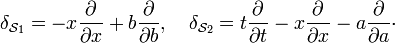Lie point symmetry
| Group theory → Lie groups Lie groups |
|---|
|
Towards the end of the nineteenth century, Sophus Lie introduced the notion of Lie group in order to study the solutions of ordinary differential equations[1][2][3] (ODEs). He showed the following main property: the order of an ordinary differential equation can be reduced by one if it is invariant under one-parameter Lie group of point transformations.[4] This observation unified and extended the available integration techniques. Lie devoted the remainder of his mathematical career to developing these continuous groups that have now an impact on many areas of mathematically based sciences. The applications of Lie groups to differential systems were mainly established by Lie and Emmy Noether, and then advocated by Élie Cartan.
Roughly speaking, a Lie point symmetry of a system is a local group of transformations that maps every solution of the system to another solution of the same system. In other words, it maps the solution set of the system to itself. Elementary examples of Lie groups are translations, rotations and scalings.
The Lie symmetry theory is a well-known subject. In it are discussed continuous symmetries opposed to, for example, discrete symmetries. The literature for this theory can be found, among other places, in these notes.[5][6][7][8][9]
Overview
Types of symmetries
Lie groups and hence their infinitesimal generators can be naturally "extended" to act on the space of independent variables, state variables (dependent variables) and derivatives of the state variables up to any finite order. There are many other kinds of symmetries. For example, contact transformations let coefficients of the transformations infinitesimal generator depend also on first derivatives of the coordinates. Lie-Bäcklund transformations let them involve derivatives up to an arbitrary order. The possibility of the existence of such symmetries was recognized by Noether.[10] For Lie point symmetries, the coefficients of the infinitesimal generators depend only on coordinates, denoted by 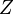 .
.
Applications
Lie symmetries were introduced by Lie in order to solve ordinary differential equations. Another application of symmetry methods is to reduce systems of differential equations, finding equivalent systems of differential equations of simpler form. This is called reduction. In the literature, one can find the classical reduction process,[4] and the moving frame-based reduction process.[11][12][13] Also symmetry groups can be used for classifying different symmetry classes of solutions.
Geometrical framework
Infinitesimal approach
Lie's fundamental theorems underline that Lie groups can be characterized by their infinitesimal generators. These mathematical objects form a Lie algebra of infinitesimal generators. Deduced "infinitesimal symmetry conditions" (defining equations of the symmetry group) can be explicitly solved in order to find the closed form of symmetry groups, and thus the associated infinitesimal generators.
Let 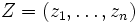 be the set of coordinates on which a system is defined where
be the set of coordinates on which a system is defined where  is the cardinal of
is the cardinal of 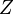 . An infinitesimal generator
. An infinitesimal generator 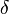 in the field
in the field  is a linear operator
is a linear operator  that has
that has 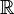 in its kernel and that satisfies the Leibniz rule:
in its kernel and that satisfies the Leibniz rule:
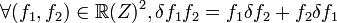 .
.
In the canonical basis of elementary derivations 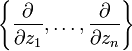 , it is written as:
, it is written as:
where 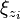 is in
is in  for all
for all  in
in 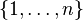 .
.
Lie groups and Lie algebras of infinitesimal generators
Lie algebras can be generated by a generating set of infinitesimal generators. To every Lie group, one can associate a Lie algebra. Roughly, a Lie algebra 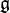 is an algebra constituted by a vector space equipped with Lie bracket as additional operation. The base field of a Lie algebra depends on the concept of invariant. Here only finite-dimensional Lie algebras are considered.
is an algebra constituted by a vector space equipped with Lie bracket as additional operation. The base field of a Lie algebra depends on the concept of invariant. Here only finite-dimensional Lie algebras are considered.
Continuous dynamical systems
A dynamical system (or flow) is a one-parameter group action. Let us denote by 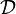 such a dynamical system, more precisely, a (left-)action of a group
such a dynamical system, more precisely, a (left-)action of a group  on a manifold
on a manifold  :
:
such that for all point 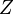 in
in  :
:
-
 where
where  is the neutral element of
is the neutral element of  ;
; - for all
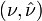 in
in  ,
, 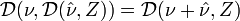 .
.
A continuous dynamical system is defined on a group  that can be identified to
that can be identified to 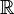 i.e. the group elements are continuous.
i.e. the group elements are continuous.
Invariants
An invariant, roughly speaking, is an element that does not change under a transformation.
Definition of Lie point symmetries
In this paragraph, we consider precisely expanded Lie point symmetries i.e. we work in an expanded space meaning that the distinction between independent variable, state variables and parameters are avoided as much as possible.
A symmetry group of a system is a continuous dynamical system defined on a local Lie group  acting on a manifold
acting on a manifold  . For the sake of clarity, we restrict ourselves to n-dimensional real manifolds
. For the sake of clarity, we restrict ourselves to n-dimensional real manifolds 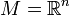 where
where  is the number of system coordinates.
is the number of system coordinates.
Lie point symmetries of algebraic systems
Let us define algebraic systems used in the forthcoming symmetry definition.
Algebraic systems
Let 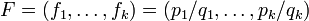 be a finite set of rational functions over the field
be a finite set of rational functions over the field 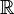 where
where  and
and  are polynomials in
are polynomials in ![\mathbb{R}[Z]](../I/m/5263328a2bf84a72a192594864f0086f.png) i.e. in variables
i.e. in variables 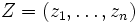 with coefficients in
with coefficients in 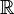 . An algebraic system associated to
. An algebraic system associated to 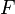 is defined by the following equalities and inequalities:
is defined by the following equalities and inequalities:
An algebraic system defined by 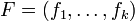 is regular (a.k.a. smooth) if the system
is regular (a.k.a. smooth) if the system 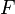 is of maximal rank
is of maximal rank  , meaning that the Jacobian matrix
, meaning that the Jacobian matrix 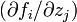 is of rank
is of rank  at every solution
at every solution 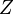 of the associated semi-algebraic variety.
of the associated semi-algebraic variety.
Definition of Lie point symmetries
The following theorem (see th. 2.8 in ch.2 of [5]) gives necessary and sufficient conditions so that a local Lie group  is a symmetry group of an algebraic system.
is a symmetry group of an algebraic system.
Theorem. Let  be a connected local Lie group of a continuous dynamical system acting in the n-dimensional space
be a connected local Lie group of a continuous dynamical system acting in the n-dimensional space 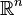 . Let
. Let 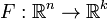 with
with 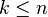 define a regular system of algebraic equations:
define a regular system of algebraic equations:
Then  is a symmetry group of this algebraic system if, and only if,
is a symmetry group of this algebraic system if, and only if,
for every infinitesimal generator 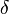 in the Lie algebra
in the Lie algebra 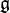 of
of  .
.
Example
Let us consider the algebraic system defined on a space of 6 variables, namely 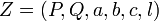 with:
with:
The infinitesimal generator
is associated to one of the one-parameter symmetry groups. It acts on 4 variables, namely 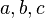 and
and  . One can easily verify that
. One can easily verify that 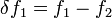 and
and  . Thus the relations
. Thus the relations 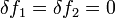 are satisfied for any
are satisfied for any 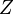 in
in  that vanishes the algebraic system.
that vanishes the algebraic system.
Lie point symmetries of dynamical systems
Let us define systems of first-order ODEs used in the forthcoming symmetry definition.
Systems of ODEs and associated infinitesimal generators
Let 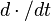 be a derivation w.r.t. the continuous independent variable
be a derivation w.r.t. the continuous independent variable  . We consider two sets
. We consider two sets 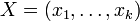 and
and 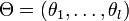 . The associated coordinate set is defined by
. The associated coordinate set is defined by  and its cardinal is
and its cardinal is  . With these notations, a system of first-order ODEs is a system where:
. With these notations, a system of first-order ODEs is a system where:
and the set 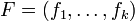 specifies the evolution of state variables of ODEs w.r.t. the independent variable. The elements of the set
specifies the evolution of state variables of ODEs w.r.t. the independent variable. The elements of the set  are called state variables, these of
are called state variables, these of 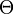 parameters.
parameters.
One can associate also a continuous dynamical system to a system of ODEs by resolving its equations.
An infinitesimal generator is a derivation that is closely related to systems of ODEs (more precisely to continuous dynamical systems). For the link between a system of ODEs, the associated vector field and the infinitesimal generator, see section 1.3 of.[4] The infinitesimal generator 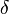 associated to a system of ODEs, described as above, is defined with the same notations as follows:
associated to a system of ODEs, described as above, is defined with the same notations as follows:
Definition of Lie point symmetries
Here is a geometrical definition of such symmetries. Let 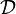 be a continuous dynamical system and
be a continuous dynamical system and 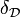 its infinitesimal generator. A continuous dynamical system
its infinitesimal generator. A continuous dynamical system  is a Lie point symmetry of
is a Lie point symmetry of 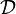 if, and only if,
if, and only if,  sends every orbit of
sends every orbit of 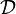 to an orbit. Hence, the infinitesimal generator
to an orbit. Hence, the infinitesimal generator 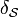 satisfies the following relation[8] based on Lie bracket:
satisfies the following relation[8] based on Lie bracket:
where  is any constant of
is any constant of 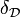 and
and 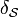 i.e.
i.e. 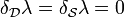 . These generators are linearly independent.
. These generators are linearly independent.
One does not need the explicit formulas of 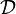 in order to compute the infinitesimal generators of its symmetries.
in order to compute the infinitesimal generators of its symmetries.
Example
Consider Pierre François Verhulst's logistic growth model with linear predation,[14] where the state variable  represents a population. The parameter
represents a population. The parameter  is the difference between the growth and predation rate and the parameter
is the difference between the growth and predation rate and the parameter 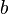 corresponds to the receptive capacity of the environment:
corresponds to the receptive capacity of the environment:
The continuous dynamical system associated to this system of ODEs is:
The independent variable  varies continuously; thus the associated group can be identified with
varies continuously; thus the associated group can be identified with 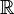 .
.
The infinitesimal generator associated to this system of ODEs is:
The following infinitesimal generators belong to the 2-dimensional symmetry group of  :
:
Software
There exist many software packages in this area.[15][16][17] For example, the package liesymm of Maple provides some Lie symmetry methods for PDEs.[18] It manipulates integration of determining systems and also differential forms. Despite its success on small systems, its integration capabilities for solving determining systems automatically are limited by complexity issues. The DETools package uses the prolongation of vector fields for searching Lie symmetries of ODEs. Finding Lie symmetries for ODEs, in the general case, may be as complicated as solving the original system.
References
- ↑ Lie, Sophus (1881). "Über die Integration durch bestimmte Integrale von einer Klasse linearer partieller Differentialgleichungen". Arch. for Math (in German) 6: 328–368.
- ↑ Lie, Sophus (1890). Theorie der Transformationsgruppen (in German) 2. Teubner, Leipzig.
- ↑ Lie, Sophus (1893). Theorie der Transformationsgruppen (in German) 3. Teubner, Leipzig.
- 1 2 3 Olver, Peter J. (1993). Applications of Lie Groups to Differential Equations (Second ed.). Springer-Verlag.
- 1 2 Olver, Peter J. (1995). Equivalence, Invariance and Symmetry. Cambridge University Press.
- ↑ Olver, Peter J. (1999). Classical Invariant Theory (First ed.). Cambridge University Press.
- ↑ Bluman, G.; Kumei, S. (1989). Symmetries and Differential Equations. Applied Mathematical Sciences Series 81 (Second ed.). New York: Springer-Verlag.
- 1 2 Stephani, H. (1989). Differential Equations (First ed.). Cambridge University Press.
- ↑ Levi, D.; Winternitz, P. (2006). "Continuous symmetries of difference equations". Journal of Physics: Mathematical and General 39: R1–R63. doi:10.1088/0305-4470/39/2/r01.
- ↑ Noether, E. (1918). "Invariante Variationsprobleme. Nachr. König. Gesell. Wissen.". Math.-Phys. Kl. (in German) (Göttingen): 235–257.
- ↑ Cartan, Elie (1935). "La méthode du repère mobile, la théorie des groups continus et les espaces généralisés.". Exposés de géométrie - 5 Hermann (in French) (Paris).
- ↑ Fels, M.; Olver, Peter J. (April 1998). "Moving Coframes: I. A Practical Algorithm". Acta Applicandae Mathematicae 51 (2): 161–213. doi:10.1023/a:1005878210297.
- ↑ Fels, M.; Olver, Peter J. (January 1999). "Moving Coframes: II. Regularization and theoretical foundations". Acta Applicandae Mathematicae 55 (2): 127–208. doi:10.1023/A:1006195823000.
- ↑ Murray, J. D. (2002). Mathematical Biology. Interdisciplinary Applied Mathematics 17. Springer.
- ↑ Heck, A. (2003). Introduction to Maple (Third ed.). Springer-Verlag.
- ↑ Schwarz, F. (1988). "Symmetries of differential equations: from Sophus Lie to computer algebra". SIAM Review 30: 450–481. doi:10.1137/1030094.
- ↑ Dimas, S.; Tsoubelis, T. (2005). "SYM: A new symmetry-finding package for Mathematica" (PDF). The 10th International Conference in MOdern GRoup ANalysis (University of Cyprus, Nicosia, Cyprus): 64–70.
- ↑ Carminati, J.; Devitt, J. S.; Fee, G. J. (1992). "Isogroups of differential equations using algebraic computing". Journal of Symbolic Computation 14 (1): 103–120. doi:10.1016/0747-7171(92)90029-4.

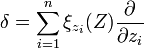
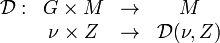


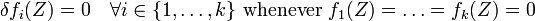

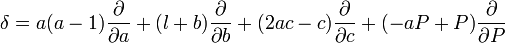
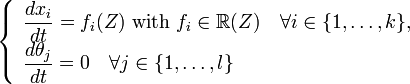
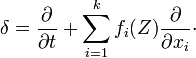
![[\delta_\mathcal{D}, \delta_\mathcal{S}] = \lambda \delta_\mathcal{D}](../I/m/9f64eb85fdbdc819ef8c2afd7fc076ee.png)