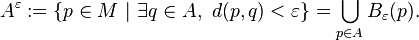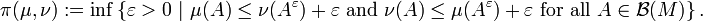Lévy–Prokhorov metric
In mathematics, the Lévy–Prokhorov metric (sometimes known just as the Prokhorov metric) is a metric (i.e., a definition of distance) on the collection of probability measures on a given metric space. It is named after the French mathematician Paul Lévy and the Soviet mathematician Yuri Vasilyevich Prokhorov; Prokhorov introduced it in 1956 as a generalization of the earlier Lévy metric.
Definition
Let  be a metric space with its Borel sigma algebra
be a metric space with its Borel sigma algebra 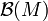 . Let
. Let 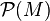 denote the collection of all probability measures on the measurable space
denote the collection of all probability measures on the measurable space 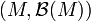 .
.
For a subset 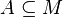 , define the ε-neighborhood of
, define the ε-neighborhood of  by
by
where 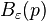 is the open ball of radius
is the open ball of radius  centered at
centered at  .
.
The Lévy–Prokhorov metric 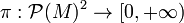 is defined by setting the distance between two probability measures
is defined by setting the distance between two probability measures 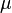 and
and 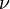 to be
to be
For probability measures clearly 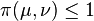 .
.
Some authors omit one of the two inequalities or choose only open or closed  ; either inequality implies the other, and
; either inequality implies the other, and  , but restricting to open sets may change the metric so defined (if
, but restricting to open sets may change the metric so defined (if  is not Polish).
is not Polish).
Properties
- If
 is separable, convergence of measures in the Lévy–Prokhorov metric is equivalent to weak convergence of measures. Thus,
is separable, convergence of measures in the Lévy–Prokhorov metric is equivalent to weak convergence of measures. Thus, 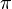 is a metrization of the topology of weak convergence on
is a metrization of the topology of weak convergence on 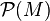 .
. - The metric space
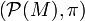 is separable if and only if
is separable if and only if  is separable.
is separable. - If
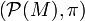 is complete then
is complete then  is complete. If all the measures in
is complete. If all the measures in 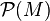 have separable support, then the converse implication also holds: if
have separable support, then the converse implication also holds: if  is complete then
is complete then 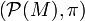 is complete.
is complete. - If
 is separable and complete, a subset
is separable and complete, a subset 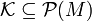 is relatively compact if and only if its
is relatively compact if and only if its 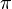 -closure is
-closure is 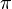 -compact.
-compact.
See also
- Lévy metric
- Prokhorov's theorem
- Tightness of measures
- weak convergence of measures
- Wasserstein metric
References
- Billingsley, Patrick (1999). Convergence of Probability Measures. John Wiley & Sons, Inc., New York. ISBN 0-471-19745-9. OCLC 41238534.
- Zolotarev, V.M. (2001), "Lévy–Prokhorov metric", in Hazewinkel, Michiel, Encyclopedia of Mathematics, Springer, ISBN 978-1-55608-010-4