Krull ring
In commutative algebra, a Krull ring or Krull domain is a commutative ring with a well behaved theory of prime factorization. They were introduced by Wolfgang Krull (1931). They are a higher-dimensional generalization of Dedekind domains, which are exactly the Krull domains of dimension at most 1.
In this article, a ring is commutative and has unity.
Formal definition
Let  be an integral domain and let
be an integral domain and let  be the set of all prime ideals of
be the set of all prime ideals of  of height one, that is, the set of all prime ideals properly containing no nonzero prime ideal. Then
of height one, that is, the set of all prime ideals properly containing no nonzero prime ideal. Then  is a Krull ring if
is a Krull ring if
-
 is a discrete valuation ring for all
is a discrete valuation ring for all  ,
,  is the intersection of these discrete valuation rings (considered as subrings of the quotient field of
is the intersection of these discrete valuation rings (considered as subrings of the quotient field of  ).
).- Any nonzero element of
 is contained in only a finite number of height 1 prime ideals.
is contained in only a finite number of height 1 prime ideals.
Properties
A Krull domain is a unique factorization domain if and only if every prime ideal of height one is principal.[1]
Let A be a Zariski ring (e.g., a local noetherian ring). If the completion 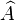 is a Krull domain, then A is a Krull domain.[2]
is a Krull domain, then A is a Krull domain.[2]
Examples
- Every integrally closed noetherian domain is a Krull ring. In particular, Dedekind domains are Krull rings. Conversely Krull rings are integrally closed, so a Noetherian domain is Krull if and only if it is integrally closed.
- If
 is a Krull ring then so is the polynomial ring
is a Krull ring then so is the polynomial ring ![A[x]](../I/m/fb69d622f2a34064c64bd69b99c1c711.png) and the formal power series ring
and the formal power series ring ![A[[x]]](../I/m/a033f6eb3b5d22bb3bcd9d208caa6c2a.png) .
. - The polynomial ring
![R[x_1, x_2, x_3, \ldots]](../I/m/0e13bad506b575e8aa95fed5bdcd89c2.png) in infinitely many variables over a unique factorization domain
in infinitely many variables over a unique factorization domain 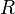 is a Krull ring which is not noetherian. In general, any unique factorization domain is a Krull ring.
is a Krull ring which is not noetherian. In general, any unique factorization domain is a Krull ring. - Let
 be a Noetherian domain with quotient field
be a Noetherian domain with quotient field 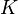 , and
, and  be a finite algebraic extension of
be a finite algebraic extension of 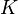 . Then the integral closure of
. Then the integral closure of  in
in  is a Krull ring (Mori–Nagata theorem).[3]
is a Krull ring (Mori–Nagata theorem).[3]
The divisor class group of a Krull ring
A (Weil) divisor of a Krull ring A is a formal integral linear combination of the height 1 prime ideals, and these form a group D(A). A divisor of the form div(x) for some non-zero x in A is called a principal divisor, and the principal divisors form a subgroup of the group of divisors. The quotient of the group of divisors by the subgroup of principal divisors is called the divisor class group of A.
A Cartier divisor of a Krull ring is a locally principal (Weil) divisor. The Cartier divisors form a subgroup of the group of divisors containing the principal divisors. The quotient of the Cartier divisors by the principal divisors is a subgroup of the divisor class group, isomorphic to the Picard group of invertible sheaves on Spec(A).
Example: in the ring k[x,y,z]/(xy–z2) the divisor class group has order 2, generated by the divisor y=z, but the Picard subgroup is the trivial group.
References
- N. Bourbaki. Commutative algebra.
- Hazewinkel, Michiel, ed. (2001), "Krull ring", Encyclopedia of Mathematics, Springer, ISBN 978-1-55608-010-4
- Krull, Wolfgang (1931), "Allgemeine Bewertungstheorie", J. Reine Angew. Math. 167: 160–196
- Hideyuki Matsumura, Commutative Algebra. Second Edition. Mathematics Lecture Note Series, 56. Benjamin/Cummings Publishing Co., Inc., Reading, Mass., 1980. xv+313 pp. ISBN 0-8053-7026-9
- Hideyuki Matsumura, Commutative Ring Theory. Translated from the Japanese by M. Reid. Cambridge Studies in Advanced Mathematics, 8. Cambridge University Press, Cambridge, 1986. xiv+320 pp. ISBN 0-521-25916-9
- Samuel, Pierre (1964), Murthy, M. Pavman, ed., Lectures on unique factorization domains, Tata Institute of Fundamental Research Lectures on Mathematics 30, Bombay: Tata Institute of Fundamental Research, MR 0214579