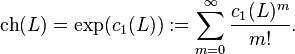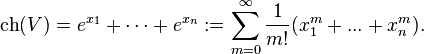K-theory

In mathematics, K-theory is, roughly speaking, the study of certain kinds of invariants of large matrices.^[1] It originated as the study of a ring generated by vector bundles over a topological space or scheme. In algebraic topology, it is an extraordinary cohomology theory known as topological K-theory. In algebra and algebraic geometry, it is referred to as algebraic K-theory. It is also a fundamental tool in the field of operator algebras.

K-theory involves the construction of families of K-functors that map from topological spaces or schemes to associated rings; these rings reflect some aspects of the structure of the original spaces or schemes. As with functors to groups in algebraic topology, the reason for this functorial mapping is that it is easier to compute some topological properties from the mapped rings than from the original spaces or schemes. Examples of results gleaned from the K-theory approach include Bott periodicity, the Atiyah-Singer index theorem and the Adams operations.

In high energy physics, K-theory and in particular twisted K-theory have appeared in Type II string theory where it has been conjectured that they classify D-branes, Ramond–Ramond field strengths and also certain spinors on generalized complex manifolds. In condensed matter physics K-theory has been used to classify topological insulators, superconductors and stable Fermi surfaces. For more details, see K-theory (physics).

Early history

The subject can be said to begin with Alexander Grothendieck (1957), who used it to formulate his Grothendieck–Riemann–Roch theorem. It takes its name from the German Klasse, meaning "class".^[2] Grothendieck needed to work with coherent sheaves on an algebraic variety X. Rather than working directly with the sheaves, he defined a group using isomorphism classes of sheaves as generators of the group, subject to a relation that identifies any extension of two sheaves with their sum. The resulting group is called K(X) when only locally free sheaves are used, or G(X) when all are coherent sheaves. Either of these two constructions is referred to as the Grothendieck group; K(X) has cohomological behavior and G(X) has homological behavior.

If X is a smooth variety, the two groups are the same. If it is a smooth affine variety, then all extensions of locally free sheaves split, so the group has an alternative definition.

In topology, by applying the same construction to vector bundles, Michael Atiyah and Friedrich Hirzebruch defined K(X) for a topological space X in 1959, and using the Bott periodicity theorem they made it the basis of an extraordinary cohomology theory. It played a major role in the second proof of the Index Theorem (circa 1962). Furthermore this approach led to a noncommutative K-theory for C*-algebras.

Already in 1955, Jean-Pierre Serre had used the analogy of vector bundles with projective modules to formulate Serre's conjecture, which states that every finitely generated projective module over a polynomial ring is free; this assertion is correct, but was not settled until 20 years later. (Swan's theorem is another aspect of this analogy.)

Developments

The other historical origin of algebraic K-theory was the work of Whitehead and others on what later became known as Whitehead torsion.

There followed a period in which there were various partial definitions of higher K-theory functors. Finally, two useful and equivalent definitions were given by Daniel Quillen using homotopy theory in 1969 and 1972. A variant was also given by Friedhelm Waldhausen in order to study the algebraic K-theory of spaces, which is related to the study of pseudo-isotopies. Much modern research on higher K-theory is related to algebraic geometry and the study of motivic cohomology.

The corresponding constructions involving an auxiliary quadratic form received the general name L-theory. It is a major tool of surgery theory.

In string theory the K-theory classification of Ramond–Ramond field strengths and the charges of stable D-branes was first proposed in 1997.^[3]

Applications

Chern characters

Main article: Chern character

Chern classes can be used to construct a homomorphism of rings from the topological K-theory of a space to (the completion of) its rational cohomology. For a line bundle L, the Chern character ch is defined by

\operatorname{ch}(L) = \exp(c_{1}(L)) := \sum_{m=0}^\infty \frac{c_1(L)^m}{m!}.

More generally, if 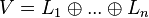$V = L_1 \oplus ... \oplus L_n$ is a direct sum of line bundles, with first Chern classes 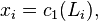$x_i = c_1(L_i),$ the Chern character is defined additively

\operatorname{ch}(V) = e^{x_1} + \dots + e^{x_n} :=\sum_{m=0}^\infty \frac{1}{m!}(x_1^m + ... + x_n^m).

The Chern character is useful in part because it facilitates the computation of the Chern class of a tensor product. The Chern character is used in the Hirzebruch-Riemann-Roch theorem.

Equivariant K-theory

Main article: Equivariant K-theory

The equivariant algebraic K-theory is an algebraic K-theory associated to the category 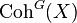$\operatorname{Coh}^G(X)$ of equivariant coherent sheaves on an algebraic scheme X with action of a linear algebraic group G, via Quillen's Q-construction; thus, by definition,

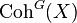K_i^G(X) = \pi_i(B^+ \operatorname{Coh}^G(X)).

In particular, $K_0^G(C)$ is the Grothendieck group of $\operatorname{Coh}^G(X)$ . The theory was developed by R. W. Thomason in 1980s.^[4] Specifically, he proved equivariant analogs of fundamental theorems such as the localization theorem.

Notes

↑ Atiyah, Michael (2000), K-Theory Past and Present, v1, arXiv:math/0012213
↑ Karoubi, 2006
↑ by Ruben Minasian (http://string.lpthe.jussieu.fr/members.pl?key=7), and Gregory Moore (http://www.physics.rutgers.edu/~gmoore) in K-theory and Ramond–Ramond Charge.
↑ Charles A. Weibel, Robert W. Thomason (1952–1995).

References

Atiyah, Michael Francis (1989), K-theory, Advanced Book Classics (2nd ed.), Addison-Wesley, ISBN 978-0-201-09394-0, MR 1043170
Friedlander, Eric; Grayson, Daniel, eds. (2005), Handbook of K-Theory, Berlin, New York: Springer-Verlag, ISBN 978-3-540-30436-4, MR 2182598
Swan, R. G. (1968), Algebraic K-Theory, Lecture Notes in Mathematics No. 76, Springer
Max Karoubi (1978), K-theory, an introduction Springer-Verlag
Max Karoubi (2006), "K-theory. An elementary introduction", arXiv:math/0602082
Allen Hatcher, Vector Bundles & K-Theory, (2003)
Charles Weibel (2013), "The K-book: an introduction to algebraic K-theory," Grad. Studies in Math. 145, American Math Society.

External links

Topology

Fields	General (point-set) Algebraic Combinatorial Continuum Differential Geometric Homology (cohomology) Set-theoretic

Key concepts	Open set / Closed set Continuity Space compact Hausdorff metric uniform Homotopy (homotopy group) Fundamental group Simplicial complex CW complex Exact sequence Homological algebra K-theory

Glossary Lists	Topics general algebraic geometric Publications

This article is issued from Wikipedia - version of the Saturday, November 15, 2014. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.