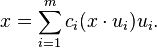John ellipsoid
In mathematics, the John ellipsoid E(K) associated to a convex body K in n-dimensional Euclidean space Rn is the ellipsoid of maximal n-dimensional volume contained within K. The John ellipsoid is named after the German mathematician Fritz John. The following refinement of John's original theorem, due to Ball (1992), gives necessary and sufficient conditions for the John ellipsoid of K to be the closed unit ball B of Rn:
The John ellipsoid E(K) of a convex body K ⊂ Rn is B if and only if B ⊆ K and there exists an integer m ≥ n and, for i = 1, ..., m, real numbers ci > 0 and unit vectors ui ∈ Sn−1 ∩ ∂K such that
and, for all x ∈ Rn
See also
- Steiner inellipse, the special case of the John ellipsoid for a triangle
References
- Ball, Keith M. (1992). "Ellipsoids of maximal volume in convex bodies". Geom. Dedicata 41 (2): 241–250. doi:10.1007/BF00182424. ISSN 0046-5755.
- John, Fritz. "Extremum problems with inequalities as subsidiary conditions". Studies and Essays Presented to R. Courant on his 60th Birthday, January 8, 1948, 187—204. Interscience Publishers, Inc., New York, N. Y., 1948. OCLC 1871554 MR 30135
- Gardner, Richard J. (2002). "The Brunn-Minkowski inequality". Bull. Amer. Math. Soc. (N.S.) 39 (3): 355–405 (electronic). doi:10.1090/S0273-0979-02-00941-2. ISSN 0273-0979.
This article is issued from Wikipedia - version of the Sunday, March 17, 2013. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.