Hurwitz's theorem (composition algebras)
In mathematics, Hurwitz's theorem is a theorem of Adolf Hurwitz (1859–1919), published posthumously in 1923, on finite-dimensional unital real non-associative algebras endowed with a positive-definite quadratic form. The theorem states that if the quadratic form defines a homomorphism into the positive real numbers on the non-zero part of the algebra, then the algebra must be isomorphic to the real numbers, the complex numbers, the quaternions or the octonions. The non-associative algebras occurring are called Hurwitz algebras or composition algebras. The problem has an equivalent formulation in terms of quadratic forms q(x), composability requiring the existence of a bilinear "composition" z(x, y) such that q(x) q(y) = q(z (x, y)). Subsequent proofs have used the Cayley–Dickson construction. Although neither commutative nor associative, composition algebras have the special property of being alternative algebras, i.e. left and right multiplication preserves squares, a weakened version of associativity. Their theory has subsequently been generalized to arbitrary quadratic forms and arbitrary fields.[1]
Hurwitz's theorem implies that multiplicative formulas for sums of squares can only occur in 1, 2, 4 and 8 dimensions, a result originally proved by Hurwitz in 1898. It is a special case of the Hurwitz problem, solved also in Radon (1922). Subsequent proofs of the restrictions on the dimension have been given by Eckmann (1943) using the representation theory of finite groups and by Lee (1948) and Chevalley (1954) using Clifford algebras. Hurwitz's theorem has been applied in algebraic topology to problems on vector fields on spheres and the homotopy groups of the classical groups[2] and in quantum mechanics to the classification of simple Jordan algebras.[3]
Euclidean Hurwitz algebras
Definition
A Hurwitz algebra or composition algebra is a finite-dimensional not necessarily nonassociative algebra A with identity endowed with a nondegenerate quadratic form q such that q(a b) = q(a) q(b). If the underlying coefficient field is the reals and q is positive-definite, so that (a, b) = 1/2[q(a + b) − q(a) − q(b)] is an inner product, then A is called a Euclidean Hurwitz algebra.[4]
If A is a Euclidean Hurwitz algebra and a is in A, define the involution and right and left multiplication operators by
Evidently the involution has period two and preserves the inner product and norm. These operators have the following properties:
- the involution is an antiautomorphism, i.e. (a b)*=b* a*
- a a* = ‖ a ‖2 1 = a* a
- L(a*) = L(a)*, R(a*) = R(a)*, so that the involution on the algebra corresponds to taking adjoints
- Re(a b) = Re(b a) if Re x = (x + x*)/2 = (x, 1)1
- Re(a b) c = Re a(b c)
- L(a2) = L(a)2, R(a2) = R(a)2, so that A is an alternating algebra
These properties are proved starting from the polarized version of the identity (a b, a b) = (a, a)(b, b):
Setting b = 1 or d = 1 yields L(a*) = L(a)* and R(c*) = R(c)*.
Hence Re(a b) = (a b, 1)1 = (a, b*)1 = (b a, 1)1 = Re(b a).
Similarly Re (a b)c = ((a b)c,1)1 = (a b, c*)1 = (b, a* c*)1 = (bc,a*)1 = (a(bc),1)1 = Re a(b c).
Hence ((ab)*,c) = (ab,c*) = (b,a*c*) = (1,b*(a*c*)) = (1,(b*a*)c*) = (b*a*,c), so that (ab)* = b*a*.
By the polarized identity ‖ a ‖2 (c, d) = (a c, a d) = (a* a c, d) so L(a*) L(a) = ‖ a ‖2. Applied to 1 this gives a* a = ‖ a ‖2. Replacing a by a* gives the other identity.
Substituting the formula for a* in L(a*) L(a) = L(a* a) gives L(a)2 = L(a2).
Classification
It is routine to check that the real numbers R, the complex numbers C and the quaternions H are examples of associative Euclidean Hurwitz algebras with their standard norms and involutions. There are moreover natural inclusions R ⊂ C ⊂ H.
Analysing such an inclusion leads to the Cayley–Dickson construction, formalized by A.A. Albert. Let A be a Euclidean Hurwitz algebra and B a proper unital subalgebra, so a Euclidean Hurwitz algebra in its own right. Pick a unit vector j in A orthogonal to B. Since (j, 1) = 0, it follows that j* = −j and hence j2 = −1. Let C be subalgebra generated by B and j. It is unital and is again a Euclidean Hurwitz algebra. It satisfies the following Cayley–Dickson multiplication laws:
To check this note that B and B j are orthogonal, since j is orthogonal to B. If a is in B, then j a = a* j, since by orthogonal 0 = 2 (j, a*) = j a − a* j. The formula for the involution follows. To show that B ⊕ B j is closed under multiplication note that Bj = j B. Since B j is orthogonal to 1, (b j)* = −b j.
- b(c j) = (c b)j since (b, j) = 0 so that, for x in A, (b(c j), x) = (b(j x), j(c j) = −(b(j x), c*) = −(c b, (j x)*) = −((c b)j, x*) = ((c b)j, x).
- (j c)b = j(b c) taking adjoints above.
- (b j)(c j) = −c* b since (b, c j) = 0, so that, for x in A, ((b j)(c j), x) = −((c j)x*, b j) = (b x*, (c j)j) = −(c* b, x).
Imposing the multiplicativity of the norm on C for a + b j and c + d j gives:
which leads to
Hence d(a c) = (d a)c, so that B must be associative.
This analysis applies to the inclusion of R in C and C in H. Taking O = H ⊕ H with the product and inner product above gives a noncommutative nonassociative algebra generated by J = (0, 1). This recovers the usual definition of the octonions or Cayley numbers. If A is a Euclidean algebra, it must contain R. If it is strictly larger than R, the argument above shows that it contains C. If it is larger than C, it contains H. If it is larger still, it must contain O. But there the process must stop, because O is not associative. In fact H is not commutative and a(b j) = (b a) j ≠ (a b)j in O.[5]
Theorem. The only Euclidean Hurwitz algebras are the real numbers, the complex numbers, the quaternions and the octonions.
Other proofs
The proofs of Lee (1948) and Chevalley (1954) use Clifford algebras to show that the dimension N of A must be 1, 2, 4 or 8. In fact the operators L(a) with (a, 1) = 0 satisfy L(a)2 = −‖ a ‖2 and so form a real Clifford algebra. If a is a unit vector, then L(a) is skew-adjoint with square −I. So N must be even. The real Clifford algebra and its complexification act on the complexification of A, an N-dimensional complex space. Since N is even, N − 1 is odd, so the Clifford algebra has exactly two complex irreducible representations of dimension 2N/2 − 1. So this power of 2 must divide N. It is easy to see that this implies N can only be 1, 2, 4 or 8.
The proof of Eckmann (1954) uses the representation theory of finite groups, or the projective representation theory of elementary Abelian 2-groups, known to be equivalent to the representation theory of real Clifford algebras. Indeed, taking an orthonormal basis ei of the orthogonal complement of 1 gives rise to operators Ui = L(ei) satisfying
This is a projective representation of a direct product of N − 1 groups of order 2. (N is assumed to be greater than 1.) The operators Ui by construction are skew-symmetric and orthogonal. In fact Eckmann constructed operators of this type in a slightly different but equivalent way. It is in fact the method originally followed in Hurwitz (1923).[6] Assume that there is a composition law for two forms
where zi is bilinear in x and y. Thus
where the matrix T(x) = (aij) is linear in x. The relations above are equivalent to
Writing
the relations become
Now set Vi = (TN)t Ti. Thus VN = I and the V1, ... , VN − 1 are skew-adjoint, orthogonal satisfying exactly the same relations as the Ui's:
Since Vi is an orthogonal matrix with square −I on a real vector space, N is even.
Let G be the finite group generated by elements vi such that
where ε is central of order 2. The commutator subgroup [G, G] is just formed of 1 and ε. If N is odd this coincides with the center while if N is even the center has order 4 with extra elements γ = v1, ... , vN − 1 and ε γ. If g in G is not in the center its conjugacy class is exactly g and ε g. Thus there are 2N − 1 + 1 conjugacy classes for N odd and 2N − 1 + 2 for N even. G has | G / [G, G] | = 2N − 1 1-dimensional complex representations. The total number of irreducible complex representations is the number of conjugacy classes. So since N is even, there are two further irreducible complex representations. Since the sum of the squares of the dimensions equals | G | and the dimensions divide | G |, the two irreducibles must have dimension 2(N − 2)/2. When N is even, there are two and their dimension must divide the order of the group, so is a power of two, so they must both have dimension 2(N − 2)/2. The space on which the Vi's act can be complexified. It will have complex dimension N. It breaks up into some of complex irreducible representations of G, all having dimension 2(N − 2)/2. In particular this dimension is ≤ N, so N is less than or equal to 8. If N = 6, the dimension is 4, which does not divide 6. So N can only be 1, 2, 4 or 8.
Applications to Jordan algebras
Let A be a Euclidean Hurwitz algebra and let Mn(A) be the algebra of n-by-n matrices over A. It is a unital nonassociative algebra with an involution given by
The trace Tr(X) is defined as the sum of the diagonal elements of X and the real-valued trace by TrR(X) = Re Tr(X). The real-valued trace satisfies:
These are immediate consequences of the known identities for n = 1.
In A define the associator by
It is trilinear and vanishes identically if A is associative. Since A is an alternating algebra [a, a, b] = 0 and [b, a, a] = 0. Polarizing it follows that the associator is antisymmetric in its three entries. Note also that a, b or c lie in R then the [a, b, c] = 0. This implies that M3(A) has certain commutation properties. In fact if X is a matrix in M3(A) with real entries on the diagonal then
with a in A. In fact if Y = [X, X2], then
Since the diagonal entries of X are real, the off diagonal entries of Y vanish. Each diagonal entry of Y is a sum of two associators involving only off diagonal terms of X. Since the associators are invariant under cyclic permutations, the diagonal entries of Y are all equal.
Let Hn(A) be the space of self-adjoint elements in Mn(A) with product X∘Y = 1/2(X Y + Y X) and inner product (X, Y) = TrR(X Y).
Theorem. Hn(A) is a Euclidean Jordan algebra if A is associative (the real numbers, complex numbers or quaternions) and n ≥ 3 or if A is nonassociative (the octonions) and n = 3.
The exceptional Jordan algebra H3(O) is called the Albert algebra after A.A. Albert.
To check that Hn(A) satisfies the axioms for a Euclidean Jordan algebra, note that the real trace defines a symmetric bilinear form with (X, X) = ∑ ‖ xij ‖2. So it is an inner product. It satisfies the associativity property (Z∘X, Y) = (X, Z∘Y) because of the properties of the real trace. The main axiom to check is the Jordan condition for the operators L(X) defined by L(X)Y = X∘Y:
This is easy to check when A is associative, since Mn(A) is an associative algebra so a Jordan algebra with X∘Y = 1/2(X Y + Y X). When A = O and n = 3 a special argument is required, one of the shortest being due to Freudenthal (1951).[7]
In fact if T is in H3(O) with Tr T = 0, then
defines a skew-adjoint derivation of H3(O). Indeed,
so that
Polarizing yields:
Setting Z = 1, shows that D is skew-adjoint. The derivation property D(X∘Y) = D(X)∘Y + X∘D(Y) follows by this and the associativity property of the inner product in the identity above.
With A and n as in the statement of the theorem, let K be the group of automorphisms of E = Hn(A) leaving invariant the inner product. It is a closed subgroup of O(E) so a compact Lie group. Its Lie algebra consists of skew-adjoint derivations. Freudenthal (1951) showed that given X in E there is an automorphism k in K such that k(X) is a diagonal matrix. (By self-adjointness the diagonal entries will be real.) Freudenthal's diagonalization theorem immediately implies the Jordan condition, since Jordan products by real diagonal matrices commute on Mn(A) for any non-associative algebra A.
To prove the diagonalization theorem, take X in E. By compactness k can be chosen in K minimizing the sums of the squares of the norms of the off-diagonal terms of k(X). Since K preserves the sums of all the squares, this is equivalent to maximizing the sums of the squares of the norms of the diagonal terms of k(X). Replacing X by k X, it can be assumed that the maximum is attained at X. Since the symmetric group Sn, acting by permuting the coordinates, lies in K, if X is not diagonal, it can be supposed that x12 and its adjoint x21 are non-zero. Let T be the skew-adjoint matrix with (2, 1) entry a, (1, 2) entry −a* and 0 elsewhere and let D be the derivation ad T of E. Let kt = exp tD in K. Then only the first two diagonal entries in X(t) = ktX differ from those of X. The diagonal entries are real. The derivative of x11(t) at t = 0 is the (1, 1) coordinate of [T, X], i.e. a* x21 + x12 a = 2(x21, a). This derivative is non-zero if a = x21. On the other hand, the group kt preserves the real-valued trace. Since it can only change x11 and x22, it preserves their sum. However, on the line x + y =constant, x2 + y2 has no local maximum (only a global minimum), a contradiction. Hence X must be diagonal.
See also
Notes
- ↑ See:
- ↑ See:
- ↑ Jordan, von Neumann & Wigner 1934
- ↑ Faraut, Koranyi & 1994 p82
- ↑ Faraut & Koranyi 1994, pp. 81–86
- ↑ See:
- Hurwitz 1923, p. 11
- Herstein 1968, pp. 141–144
- ↑ See:
- Faraut & Koranyi 1994, pp. 88–91
- Postnikov 1986
References
- Albert, A. A. (1934), "On a certain algebra of quantum mechanics", Ann. of Math. 35: 65–73, JSTOR 1968118
- Chevalley, C. (1954), The algebraic theory of spinors and Clifford algebras, Columbia University Press
- Eckmann, Beno (1943), "Gruppentheoretischer Beweis des Satzes von Hurwitz–Radon über die Komposition quadratischer Formen", Comment. Math. Helv. 15: 358–366, doi:10.1007/bf02565652
- Eckmann, Beno (1989), "Hurwitz–Radon matrices and periodicity modulo 8", Enseign. Math. 35: 77–91
- Eckmann, Beno (1999), "Topology, algebra, analysis—relations and missing links", Notices Amer. Math. Soc. 46: 520–527
- Faraut, J.; Koranyi, A. (1994), Analysis on symmetric cones, Oxford Mathematical Monographs, Oxford University Press, ISBN 0198534779
- Freudenthal, Hans (1951), Oktaven, Ausnahmegruppen und Oktavengeometrie, Mathematisch Instituut der Rijksuniversiteit te Utrecht
- Freudenthal, Hans (1985), "Oktaven, Ausnahmegruppen und Oktavengeometrie", Geom. Dedicata 19: 7–63, doi:10.1007/bf00233101 (reprint of 1951 article)
- Herstein, I. N. (1968), Noncommutative rings, Carus Mathematical Monographs 15, Mathematical Association of America, ISBN 088385015X
- Hurwitz, A. (1898), "Über die Composition der quadratischen Formen von beliebig vielen Variabeln", Goett. Nachr.: 309–316
- Hurwitz, A. (1923), "Über die Komposition der quadratischen Formen", Math. Ann. 88: 1–25, doi:10.1007/bf01448439
- Jacobson, N. (1968), Structure and representations of Jordan algebras, American Mathematical Society Colloquium Publications 39, American Mathematical Society
- Jordan, P.; von Neumann, J.; Wigner, E. (1934), "On an algebraic generalization of the quantum mechanical formalism", Ann. of Math. 35: 29–64, JSTOR 1968117
- Lam, Tsit-Yuen (2005), Introduction to Quadratic Forms over Fields, Graduate Studies in Mathematics 67, American Mathematical Society, ISBN 0-8218-1095-2, MR 2104929, Zbl 1068.11023
- Lee, H. C. (1948), "Sur le théorème de Hurwitz-Radon pour la composition des formes quadratiques", Comment. Math. Helv. 21: 261–269, doi:10.1007/bf02568038
- Porteous, I.R. (1969), Topological Geometry, Van Nostrand Reinhold, ISBN 0-442-06606-6, Zbl 0186.06304
- Postnikov, M. (1986), Lie groups and Lie algebras. Lectures in geometry. Semester V, Mir
- Radon, J. (1922), "Lineare scharen orthogonaler matrizen", Abhandlungen aus dem Mathematischen Seminar der Universität Hamburg 1: 1–14, doi:10.1007/bf02940576
- Rajwade, A. R. (1993), Squares, London Mathematical Society Lecture Note Series 171, Cambridge University Press, ISBN 0-521-42668-5, Zbl 0785.11022
- Schafer, Richard D. (1995) [1966], An introduction to non-associative algebras, Dover Publications, ISBN 0-486-68813-5, Zbl 0145.25601
- Shapiro, Daniel (2000), Compositions of quadratic forms, De Gruyter Expositions in Mathematics 33, Walter de Gruyter, ISBN 311012629X
Further reading
- Baez, John C. (2002), "The octonions", Bull. Amer. Math. Soc. 39: 145–205, doi:10.1090/S0273-0979-01-00934-X
- Conway, John H.; Smith, Derek A. (2003), On quaternions and octonions: their geometry, arithmetic, and symmetry, A K Peters, ISBN 1568811349
- Kantor, I.L.; Solodovnikov, A.S. (1989), "Normed algebras with an identity. Hurwitz's theorem.", Hypercomplex numbers. An elementary introduction to algebras, Trans. A. Shenitzer (2nd ed.), Springer-Verlag, p. 121, ISBN 0-387-96980-2, Zbl 0669.17001
- Max Koecher & Reinhold Remmert (1990) "Composition Algebras. Hurwitz's Theorem — Vector-Product Algebras", chapter 10 of Numbers by Heinz-Dieter Ebbinghaus et al., Springer, ISBN 0-387-97202-1
- Shapiro, Daniel B. (2000), "Appendix to Chapter 1. Composition algebras", Compositions of quadratic forms, de Gruyter Expositions in Mathematics 33, Walter de Gruyter, pp. 21 ff, ISBN 3-11-012629-X, Zbl 0954.11011
- Springer, T. A.; F. D. Veldkamp (2000), Octonions, Jordan Algebras and Exceptional Groups, Springer-Verlag, ISBN 3-540-66337-1 Cite uses deprecated parameter
|coauthors=(help)
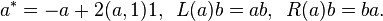

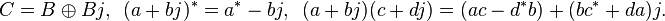




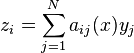




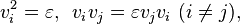


![\displaystyle{[a,b,c]=a(bc) - (ab)c.}](../I/m/3e58b393db4c7b432be4f4ff4e1bc6d5.png)
![\displaystyle{[X,X^2]=aI,}](../I/m/436a3f1913203c3f088653592ec2c494.png)
![\displaystyle{y_{ij}=\sum_{k,\ell} [x_{ik},x_{k\ell},x_{\ell j}].}](../I/m/e3f9a9bf417862a8c8406d35b0f0d363.png)
![\displaystyle{[L(X),L(X^2)]=0.}](../I/m/1b49f1c20a6398d20deb5382fba2fe15.png)



